Pyrochoron (EntityTopic, 17)
From Hi.gher. Space
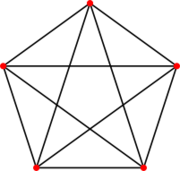
The pyrochoron, also known as the pentachoron and the 5-cell, is the four-dimensional simplex, and has the lowest possible element count of any flat, non-degenerate four-dimensional shape. It consists of 5 regular tetrahedra joined at their faces, folded into 4D to form a 4D volume. It is a special case of the pyramid where the base is a tetrahedron. There are 3 tetrahedra surrounding every edge.
Coordinates
The coordinates of a pentachoron centered at the origin are:
(-1, -√3/3, -√6/6, -√10/10)
(1, -√3/3, -√6/6, -√10/10)
(0, 2√3/3, -√6/6, -√10/10)
(0, 0, √6/2, -√10/10)
(0, 0, 0, 2√10/5)
Equations
- The hypervolumes of a pentachoron with side length l are given by:
total edge length = 10l
total surface area = 5√3∕2 · l2
surcell volume = 5√2∕12 · l3
bulk = √5∕96 · l4
Net
The net of a pentachoron is a tetrahedron surrounded by 4 more tetrahedra.
Projection
Cell-first / vertex-first projection
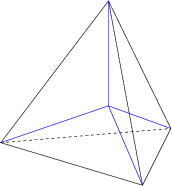
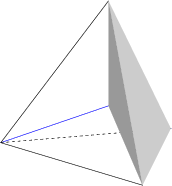
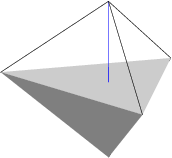
The following diagram shows a perspective projection of the pentachoron.
The dotted line shows the far edge of the outer tetrahedron. The blue lines are inside the outer tetrahedron in this projection. The center of the projection where these blue lines meet is actually the apex of the pentachoron pointing away from us in the 4th direction.
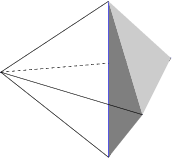
All 5 tetrahedral cells of the pentachoron are present in this diagram: the outer tetrahedron, and the 4 “inner” tetrahedra outlined by one triangular face of the outer tetrahedron and 3 of the blue lines each. Although they appear as slightly flattened tetrahedra, this is only because they are being viewed at from an angle. In actuality, they are perfectly regular tetrahedra.
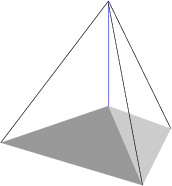
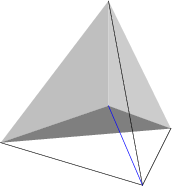
The following diagrams illustrate 3 of these cells.
Note that if a 4D being were to look at an actual pentachoron, it would not be able to see all 5 cells at once. Rather, it would either see only the outer tetrahedron (if it looks at the “base” of the pentachoron), or the 4 inner cells (if it looks at the apex of the pentachoron).
Edge-first / face-first projection
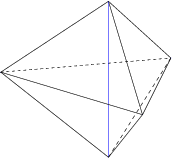
The next diagram shows the pentachoron viewed at from another angle.
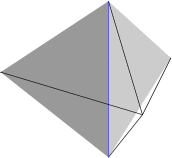
In this diagram, three of the pentachoron's cells are arranged around the central axis indicated by the blue line. The other two cells are the upper and lower halves of the outer shape, which is called a trigonal bipyramid. All the cells appear somewhat deformed from a regular tetrahedron, because they are all being viewed at from an angle. Some of these cells are shown below:
Again, this projection represents two possible views of the pentachoron. From one view, the 4D being would see only the upper and lower tetrahedra. From the opposite view, it would see the 3 inner tetrahedra. It cannot see all 5 cells at once unless the pentachoron is transparent.
Incidence matrix
Dual: Self-dual
| # | TXID | Va | Ea | 3a | C1a | Type | Name |
|---|---|---|---|---|---|---|---|
| 0 | Va | = point | ; | ||||
| 1 | Ea | 2 | = digon | ; | |||
| 2 | 3a | 3 | 3 | = triangle | ; | ||
| 3 | C1a | 4 | 6 | 4 | = base of pyramid: tetrahedron | ; | |
| 4 | H4.1a | 5 | 10 | 10 | 5 | = pyrochoron | ; |
Usage as facets
- 32× 1-facets of a aeroteron
- pyramid: 6× 1-facets of a pyroteron
- 24× 1-facets of a (dual of triangular triprism)
| Simplices |
| triangle • tetrahedron • pyrochoron • pyroteron • pyropeton |
| Notable Tetrashapes | |
| Regular: | pyrochoron • aerochoron • geochoron • xylochoron • hydrochoron • cosmochoron |
| Powertopes: | triangular octagoltriate • square octagoltriate • hexagonal octagoltriate • octagonal octagoltriate |
| Circular: | glome • cubinder • duocylinder • spherinder • sphone • cylindrone • dicone • coninder |
| Torii: | tiger • torisphere • spheritorus • torinder • ditorus |
| 27. [111]1 Triangular prismic pyramid | 28. 13 Pentachoron | 29. 1111 Duotrianglinder |
| List of tapertopes | ||