Cosmochoron (EntityTopic, 12)
From Hi.gher. Space
The cosmochoron, also known as the hecatonicosachoron and the 120-cell, is a 4D polytope bounded by 120 dodecahedra. It is the highest dimensional analog of the pentagon and the dodecahedron.
Each dodecahedron in its net lies on a ring of ten dodecahedra along a great circle of the glome. There are four dodecahedra on such a ring between any dodecahedron and its antipodal dodecahedron.
Coordinates
The coordinates of the 120-cell with edge length 2/φ2 are all permutations of coordinates and changes of sign of:
(2, 2, 0, 0)
(√5, 1, 1, 1)
(φ, φ, φ, 2-φ)
(φ+1, φ−1, φ−1, φ−1)
Together with all even permutations of coordinates and all changes of sign of:
(φ+1, 2-φ, 1, 0)
(√5, φ−1, φ, 0)
(2, 1, φ, φ−1)
Equations
- The hypervolumes of a cosmochoron with side length l are given by:
total edge length = 1200l
total surface area = 180√(25 + 10√5) · l2
surcell volume = 30(15 + 7√5) · l3
bulk = 15∕4(105 + 47√5) · l4
Projection
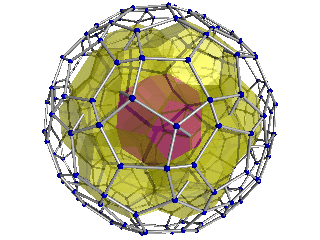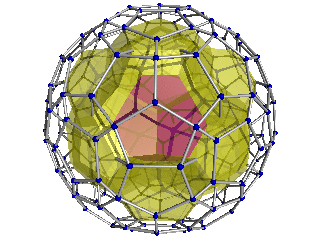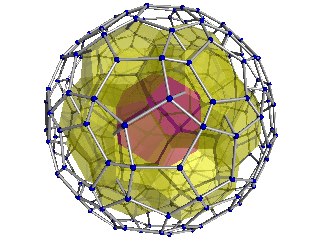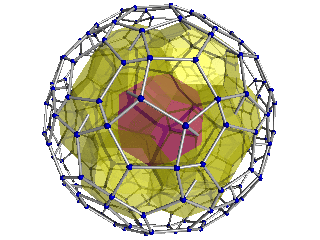
Here are two perspective projections of the hecatonicosachoron into 3D. The highlighted center cell is closest to the 4D viewpoint. It is surrounded by 12 dodecahedra in a second layer. The right-hand image also shows the third layer of 32 dodecahedra. After this is a fourth layer of 30 dodecahedra lying on the spherical "equator", followed by three more layers mirroring the three layers seen here (not seen here, because they lie on the 4D "back" of the shape).
Note that only the projection is rotating; the 4D shape itself is not.
Incidence matrix
Dual: hydrochoron
| # | TXID | Va | Ea | 5a | C1a | Type | Name |
|---|---|---|---|---|---|---|---|
| 0 | Va | = point | ; | ||||
| 1 | Ea | 2 | = digon | ; | |||
| 2 | 5a | 5 | 5 | = pentagon | ; | ||
| 3 | C1a | 20 | 30 | 12 | = dodecahedron | ; | |
| 4 | H4.1a | 600 | 1200 | 720 | 120 | = cosmochoron | ; |
Usage as facets
This polytope does not currently appear as facets in any higher-dimensional polytopes in the database.
| Notable Tetrashapes | |
| Regular: | pyrochoron • aerochoron • geochoron • xylochoron • hydrochoron • cosmochoron |
| Powertopes: | triangular octagoltriate • square octagoltriate • hexagonal octagoltriate • octagonal octagoltriate |
| Circular: | glome • cubinder • duocylinder • spherinder • sphone • cylindrone • dicone • coninder |
| Torii: | tiger • torisphere • spheritorus • torinder • ditorus |