Spheritorus (EntityTopic, 11)
From Hi.gher. Space
(Redirected from Toratope 5b)
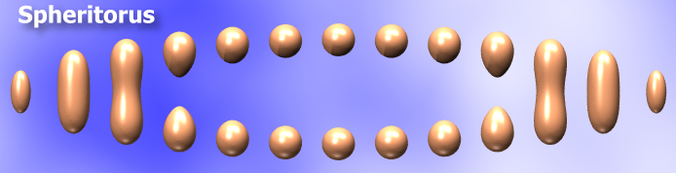
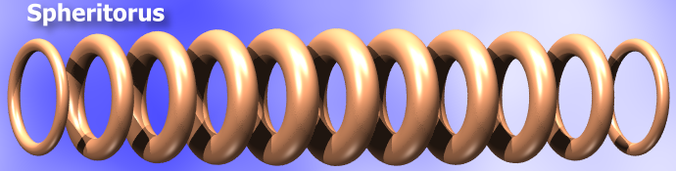
Of all the four-dimensional torii, the spheritorus, previously known as the toracubinder, is the closest analog to the three-dimensional torus. It is formed by taking an uncapped spherinder and connecting its ends in a loop. Its toratopic dual is the torisphere. It has two possible cross-sections in coordinate planes through the origin: the torus, and two disjoint spheres.
Equations
- Variables:
R ⇒ major radius of the spheritorus
r ⇒ minor radius of the spheritorus
h ⇒ height of the spheritorus
- All points (x, y, z, w) that lie on the surcell of a spheritorus will satisfy the following equation:
(√(x2 + y2) − R)2 + z2 + w2 = r2
- The parametric equations are:
x = r cos a cos b cos c + R cos c
y = r cos a cos b sin c + R sin c
z = r cos a sin b
w = r sin a
- The hypervolumes of a spheritorus are given by:
total edge length = Unknown
total surface area = Unknown
surcell volume = 4π2Rr(r+h)
bulk = 2π2Rr2h
- The realmic cross-sections (n) of a spheritorus are:
Unknown
Cross-sections
Jonathan Bowers aka Polyhedron Dude created these two excellent cross-section renderings:
| Notable Tetrashapes | |
| Regular: | pyrochoron • aerochoron • geochoron • xylochoron • hydrochoron • cosmochoron |
| Powertopes: | triangular octagoltriate • square octagoltriate • hexagonal octagoltriate • octagonal octagoltriate |
| Circular: | glome • cubinder • duocylinder • spherinder • sphone • cylindrone • dicone • coninder |
| Torii: | tiger • torisphere • spheritorus • torinder • ditorus |
| 4a. IIII Tesseract | 4b. (IIII) Glome | 5a. (II)II Cubinder | 5b. ((II)II) Spheritorus | 6a. (II)(II) Duocylinder | 6b. ((II)(II)) Tiger |
| List of toratopes | |||||
