Spheritorus (EntityTopic, 11)
From Hi.gher. Space
(Difference between revisions)
m (K6.4 upgrade: img -> embed) |
|||
| (10 intermediate revisions not shown) | |||
| Line 1: | Line 1: | ||
| + | <[#ontology [kind topic] [cats 4D Curved Toratope]]> | ||
{{STS Shape | {{STS Shape | ||
| - | |||
| dim=4 | | dim=4 | ||
| elements=1, ?, ?, 0 | | elements=1, ?, ?, 0 | ||
| Line 6: | Line 6: | ||
| ssc2=T((2)2) | | ssc2=T((2)2) | ||
| extra={{STS Toratope | | extra={{STS Toratope | ||
| - | | | + | | expand=[[Cylspherinder|32]] |
| notation=((II)II) | | notation=((II)II) | ||
| index=5b | | index=5b | ||
}}}} | }}}} | ||
| - | Of all the four-dimensional torii, the toracubinder is the closest analog to the three-dimensional [[torus]]. It is formed by taking an uncapped [[spherinder]] and connecting its ends in a loop | + | Of all the [[four-dimensional torii]], the '''spheritorus''', previously known as the '''toracubinder''', is the closest analog to the three-dimensional [[torus]]. It is formed by taking an uncapped [[spherinder]] and connecting its ends in a loop. Its [[toratopic dual]] is the [[torisphere]]. It has two possible cross-sections in coordinate planes through the origin: the [[torus]], and two disjoint [[sphere]]s. |
== Equations == | == Equations == | ||
*Variables: | *Variables: | ||
| - | <blockquote>''R'' ⇒ major radius of the | + | <blockquote>''R'' ⇒ major radius of the spheritorus<br> |
| - | ''r'' ⇒ minor radius of the | + | ''r'' ⇒ minor radius of the spheritorus<br> |
| - | ''h'' ⇒ height of the | + | ''h'' ⇒ height of the spheritorus</blockquote> |
| - | *All points (''x'', ''y'', ''z'', ''w'') that lie on the [[surcell]] of a | + | *All points (''x'', ''y'', ''z'', ''w'') that lie on the [[surcell]] of a spheritorus will satisfy the following equation: |
| - | <blockquote>( | + | <blockquote>(√(''x''<sup>2</sup> + ''y''<sup>2</sup>) − ''R'')<sup>2</sup> + ''z''<sup>2</sup> + ''w''<sup>2</sup> = ''r''<sup>2</sup></blockquote> |
*The parametric equations are: | *The parametric equations are: | ||
| Line 28: | Line 28: | ||
w = r sin a </blockquote> | w = r sin a </blockquote> | ||
| - | *The [[hypervolume]]s of a | + | *The [[hypervolume]]s of a spheritorus are given by: |
<blockquote>total edge length = ''Unknown''<br> | <blockquote>total edge length = ''Unknown''<br> | ||
total surface area = ''Unknown''<br> | total surface area = ''Unknown''<br> | ||
| Line 34: | Line 34: | ||
bulk = 2π<sup>2</sup>''Rr''<sup>2</sup>''h''</blockquote> | bulk = 2π<sup>2</sup>''Rr''<sup>2</sup>''h''</blockquote> | ||
| - | *The [[realmic]] [[cross-section]]s (''n'') of a | + | *The [[realmic]] [[cross-section]]s (''n'') of a spheritorus are: |
<blockquote>''Unknown''</blockquote> | <blockquote>''Unknown''</blockquote> | ||
| + | |||
| + | == Cross-sections == | ||
| + | [[User:Polyhedron Dude|Jonathan Bowers aka Polyhedron Dude]] created these two excellent cross-section renderings:<br/> | ||
| + | <[#embed [hash ZW0YGY7T1RAMWQP9SZH1JG565J] [width 676]]><br/> | ||
| + | <[#embed [hash FM78R1H3E99EGCA10DWAJMX0M5] [width 676]]> | ||
| + | |||
{{Tetrashapes}} | {{Tetrashapes}} | ||
| - | {{Toratope Nav B|4|5|6|IIII<br>Tesseract|(IIII)<br>Glome|(II)II<br>Cubinder|((II)II)<br> | + | {{Toratope Nav B|4|5|6|IIII<br>Tesseract|(IIII)<br>Glome|(II)II<br>Cubinder|((II)II)<br>Spheritorus|(II)(II)<br>Duocylinder|((II)(II))<br>Tiger|chora}} |
Latest revision as of 20:47, 11 February 2014
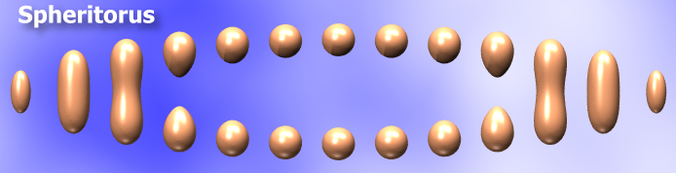
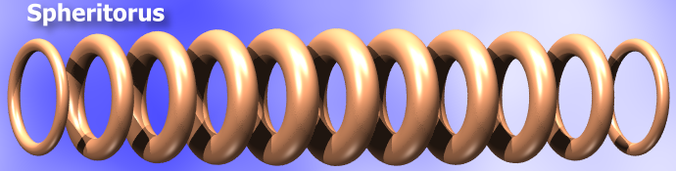
Of all the four-dimensional torii, the spheritorus, previously known as the toracubinder, is the closest analog to the three-dimensional torus. It is formed by taking an uncapped spherinder and connecting its ends in a loop. Its toratopic dual is the torisphere. It has two possible cross-sections in coordinate planes through the origin: the torus, and two disjoint spheres.
Equations
- Variables:
R ⇒ major radius of the spheritorus
r ⇒ minor radius of the spheritorus
h ⇒ height of the spheritorus
- All points (x, y, z, w) that lie on the surcell of a spheritorus will satisfy the following equation:
(√(x2 + y2) − R)2 + z2 + w2 = r2
- The parametric equations are:
x = r cos a cos b cos c + R cos c
y = r cos a cos b sin c + R sin c
z = r cos a sin b
w = r sin a
- The hypervolumes of a spheritorus are given by:
total edge length = Unknown
total surface area = Unknown
surcell volume = 4π2Rr(r+h)
bulk = 2π2Rr2h
- The realmic cross-sections (n) of a spheritorus are:
Unknown
Cross-sections
Jonathan Bowers aka Polyhedron Dude created these two excellent cross-section renderings:
| Notable Tetrashapes | |
| Regular: | pyrochoron • aerochoron • geochoron • xylochoron • hydrochoron • cosmochoron |
| Powertopes: | triangular octagoltriate • square octagoltriate • hexagonal octagoltriate • octagonal octagoltriate |
| Circular: | glome • cubinder • duocylinder • spherinder • sphone • cylindrone • dicone • coninder |
| Torii: | tiger • torisphere • spheritorus • torinder • ditorus |
| 4a. IIII Tesseract | 4b. (IIII) Glome | 5a. (II)II Cubinder | 5b. ((II)II) Spheritorus | 6a. (II)(II) Duocylinder | 6b. ((II)(II)) Tiger |
| List of toratopes | |||||
