Cyltrianglinder (EntityTopic, 11)
From Hi.gher. Space
The cyltrianglinder is the limiting shape of an n,3-duoprism as n approaches infinity. In other words, it is the Cartesian product of a circle and a triangle. It is bounded by three cylinders and a curved cell formed by bending a triangular prism in 4D and joining the ends. Its faces are three circles and three curved faces formed by joining the ends of a rectangle in 3D.
The net of a cyltrianglinder is a triangular prism surrounded by three cylinders.
Equations
- Variables:
r ⇒ radius of the circular faces
l ⇒ length of the edges in the triangles
- The hypervolumes of a cubinder are given by:
total edge length = 6πr
total surface area = 3πr(r + 2l)
surcell volume = πr(3rl + √3∕2 · l2)
bulk = √3∕4 · πr2 · l2
- The realmic cross-sections (n) of a cyltrianglinder are:
Unknown
Projection
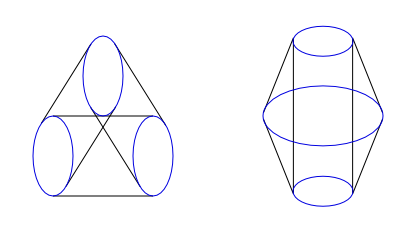
The following are two possible projections of the cyltrianglinder:
| Notable Tetrashapes | |
| Regular: | pyrochoron • aerochoron • geochoron • xylochoron • hydrochoron • cosmochoron |
| Powertopes: | triangular octagoltriate • square octagoltriate • hexagonal octagoltriate • octagonal octagoltriate |
| Circular: | glome • cubinder • duocylinder • spherinder • sphone • cylindrone • dicone • coninder |
| Torii: | tiger • torisphere • spheritorus • torinder • ditorus |
| 19. [111]1 Cubic pyramid | 20. 211 Cyltrianglinder | 21. 1111 Triangular diprism |
| List of tapertopes | ||