Stauroperihedron (EntityTopic, 11)
From Hi.gher. Space
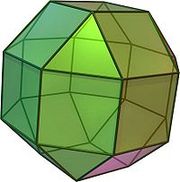
The stauroperihedron, also known as the (small) rhombicuboctahedron and cuboctahedral rectate, abbreviated here as COR, is a uniform polyhedron which can be seen as a 3-dimensional analog of the octagon. The other possible analog is the cubic truncate (CT). While the CT has the octagons on the surface of the shape, the COR has them embedded inside it. Thus when one is concerned with powertopes, the COR comprises three "long and thin" cuboids whereas the CT comprises three "wide and flat" cuboids.
For a figure centred at the origin, with edge length 2, its 24 = 3×23 vertices can be given as all permutations of
- 〈±1, ±1, ±(1+√2)〉.
As such, the most obvious 4D analog is the stauroperichoron.
Incidence matrix
Dual: deltoidal icositetrahedron
| # | TXID | Va | Ea | Eb | 4a | 4b | 3a | Type | Name |
|---|---|---|---|---|---|---|---|---|---|
| 0 | Va | = point | ; | ||||||
| 1 | Ea | 2 | = digon | ; | |||||
| 2 | Eb | 2 | = digon | ; | |||||
| 3 | 4a | 4 | 4 | 0 | = square | ; | |||
| 4 | 4b | 4 | 2 | 2 | = square | ; | |||
| 5 | 3a | 3 | 0 | 3 | = triangle | ; | |||
| 6 | C1a | 24 | 24 | 24 | 6 | 12 | 8 | = stauroperihedron | ; |
Usage as facets
This polytope does not currently appear as facets in any higher-dimensional polytopes in the database.
| Notable Trishapes | |
| Regular: | tetrahedron • cube • octahedron • dodecahedron • icosahedron |
| Direct truncates: | tetrahedral truncate • cubic truncate • octahedral truncate • dodecahedral truncate • icosahedral truncate |
| Mesotruncates: | stauromesohedron • stauroperihedron • stauropantohedron • rhodomesohedron • rhodoperihedron • rhodopantohedron |
| Snubs: | snub staurohedron • snub rhodohedron |
| Curved: | sphere • torus • cylinder • cone • frustum • crind |