student91 wrote:quickfur wrote:[...]
In general, a good number of diminishable 600-cell family uniforms can be diminished in the same way as the grand antiprism. And I think the icositetradiminished x5o3o3x may have already been found by Klitzing, as part of a general search for this pattern of diminishing in the 600-cell family. I'll let him confirm that.
What you just said here gave me an interesting idea, although I find it hard to phrase. I'll try anyway:
There is a great similarity between every cuts in the 600-cell family. In fact, the diminishings I've seen so far can all be placed in a number of groups.(e.g. the diminishings of x5o3o3x correspond to the diminishings of the 600-cell, the mesodiminishing of the x3x3o5o corresponds to the "special cuts" of the 600-cell, and so on). Now if we determine the diminishing for one of these, we can say it's applyable to all the others. (e.g. we could state there are the same amound of "special cuts" of the 600-cell as there are "normal" diminishings of the x3x3o5o.)
Well, since all the uniform polychora from the 600-cell family share the same symmetry group, they all have corresponding elements (e.g., 600-cell vertices = 120-cell cells = o5oxo icosahedra = ... etc.). Strictly speaking, any subset of these elements may be deleted in a diminishing. Of course, our interest is in CRF results, so the trick here is identifying for each uniform which symmetry elements admit CRF diminishings, and identifying which two uniforms have corresponding CRF-diminishable elements.
Which uniforms are CRF-diminishable, however, it pretty much a matter of coincidence: the 3D uniforms exhibit a more limited number of diminishings, so in 4D we are constrained by which 3D uniforms have CRF diminishings -- for example, if the dodecahedron had been CRF-diminishable, then the 120-cell and x5oox would also be CRF-diminishable in all positions where x5ox and o5xo cells could be diminished. So really, it's the intersection of the CRF requirement with the general geometric operation (which is universally applicable) that produces the "random" occurrence of CRF-diminishings. The geometric operation itself is completely general and can be applied across the board, and will produce consistent results (truncated element X in one uniform will have the corresponding result to the truncation of element Y in another uniform where X and Y correspond with the same symmetry element). It's just that many of the results will not be CRF.
But you might ask, why do these correspond. I'm still not sure about this, but I think it has something to do with the fundamental domains layout.
Actually, the reason is that all elements of the underlying 600-cell symmetry group have specific hyperplane orientations associated with them. This is easiest to see if you consider the runcinated 120-cell (x5oox), where all symmetry elements are expressed in the cells. The hyperplanes in which the pentagonal prisms sit, for example, are parallel to the hyperplanes of the octagonal prisms in x5xxo and x5xxx. So in a sense, all of these uniforms are "the same", since each symmetry element has a fixed orientation, and therefore dichoral angles among these uniforms are the same few combinations.
Furthermore, because of this same relationship among the 3D uniforms -- the rhombicosidodecahedron x5ox, for example, is just the expanded icosahedron o5ox, so cutting off a pentagonal cupola from x5ox is equivalent to cutting off a pentagonal pyramid from an icosahedron, because pentagonal cupola = Stott-expanded pentagonal pyramid. Similarly, given some CRF diminishing of the 600-cell, if some other 600-cell family uniform has similarly-diminishable elements in the same positions, then it can also be CRF-diminished in the same way. In fact, one could argue that the CRF-diminishing of the latter is just the Stott-expanded version of the 600-cell diminishing.
[...]
One interesting diminishing that I intend to construct at some point is the "swirl-diminished" o5o3x3o: it's possible to delete the top/bottom vertices of each icosahedron in a great circle of icosahedra, to form a ring of alternating pentagonal prisms and antiprisms. Since the vertices of the 600-cell can be partitioned into 12 great circles of 10 vertices each, we can perform this diminishing on 12 great circles on icosahedra in the o5o3x3o. This produces a CRF with swirlprism symmetry, consisting of 12 rings of alternating pentagonal prisms/antiprisms that swirl around each other (according to a subset of the Hopf fibration of the 3-sphere), along with a bunch of square pyramids filling in the gaps between them. This should make a good Polytope of the Month, if I find the time to resume that program.
this one indeed is interesting, do more polytopes have a dept 1 o.o5o-oriented possible diminishing? they too could be "swirl-diminished"
student91
Certainly! Any 600-cell family uniform that has CRF-diminishable elements corresponding with 600-cell edges (resp. 120-cell pentagons) will admit a CRF swirldiminishing. The 600-cell itself admits it, but is a degenerate case (you end up deleting all vertices
); o5oxo admits it as I indicated, so does x5oxo.
In any case, I have just finished constructing the swirldiminished o5oxo: as expected, it consists of 12 rings of alternating pentagonal prisms and antiprisms. Each ring has 10 of each prism, so there are a total of 120 pentagonal prisms and 120 pentagonal antiprisms. The original octahedra get bisected into square pyramids, so that's 600 square pyramids + 120 pentagonal prisms + 120 pentagonal antiprisms = 840 cells.
What's interesting, however, is that not only there are 12 rings of alternating prisms/antiprisms, the original octahedra of the o5oxo share half their faces with each other, and after the diminishing, the square pyramids also share their faces with each other. Furthermore, these square pyramids are isolated by the alternating prisms/antiprisms into individual rings of pyramids that also run around great circles of the 3-sphere. I didn't count them, but I believe there should be 20 such rings, corresponding with the
dual swirlprism symmetry to the 12 rings of prisms! So here, in a single polychoron, you have the combination of both the dodecahedral Hopf fibration and its dual icosahedral Hopf fibration, represented by two sets of rings of cells. (One could say this corresponds with an icosidodecahedral Hopf fibration, or an icosidodecahedral uniform polytwister, in which the two types of twisters correspond with the the alternating prism rings and the square pyramid rings).
This polychoron is, of course, chiral because the underlying swirlprism symmetry is chiral. Moreover, I'm almost certain that it's vertex-transitive, which is ultracool, because that would make it scaliform.
Anyway, here's a preliminary render of this cute little baby:
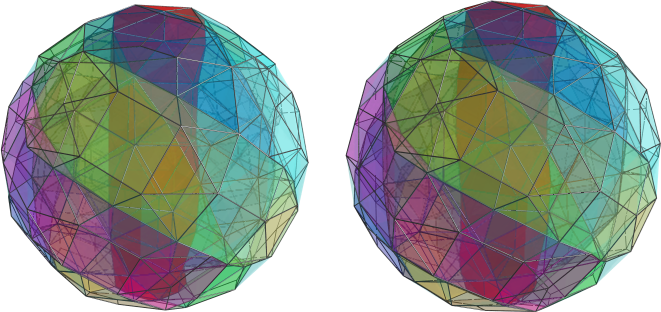
I haven't tweaked the colors yet, so it looks a bit ugly, but you can clearly see the swirling rings of alternating prisms/antiprisms here. Or rather, half-rings, since I have visibility clipping on. The red vertical column in the center is actually a half-ring seen from a 90° in the 4D viewpoint, so it appears like a vertical column, but it's actually curved in a half-circle. Its other half, of course, lies on the far side and isn't shown here. It's kinda hard to see in this poorly-colored image, but there are two layers of rings around this central column. There are 5 rings in the inner layer and 5 rings in the outer layer. You can sorta see the slanted green column swirling around the central column, behind the yellow and magenta rings, and the blue ring to the right of it. These are 2 of the 5 inner rings. The yellow and magenta columns, of course, are 2 of the 6 outer rings. There is a ring that runs orthogonal to the red column, but it is not shown here because it lies just behind the limb of the polytope, so it got vis-clipped. So we see the structure of the rings is 1+5+5+1; the outer ring of the central column is the inner ring of the orthogonal ring, and vice versa. All of the rings are equivalent to each other under the underlying swirlprism symmetry group.
Now if you look carefully at the interface between the magenta and yellow rings, you can sorta discern the partial outlines (I omitted some edges to make the image less cluttered) of a wavy trail of triangles and squares: this is where one of the rings of square pyramids are. I didn't render the square pyramids in full because it would make the image too obscured. As you can see, the pyramids interface the pentagonal prisms of one ring to the pentagonal antiprisms of the other ring, in a skewed, alternating fashion. Really fascinating.
So anyway, here you have it, the swirl-diminished rectified 600-cell.
EDIT: Here's the same projection with a slightly better coloring scheme:
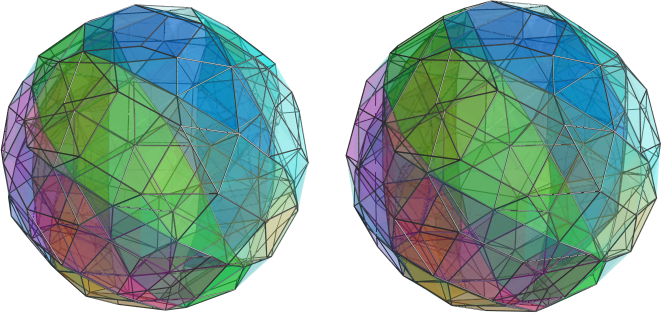
Red seems to work poorly when embedded deep inside the projection, so I changed it to white. Other than that, other colors remain the same but with transparencies tweaked.
)
)
Some of these things could compete for the crown jewels category, they are so unexpected (like the truncated dodecahedron / icosahedron mega-wedge I discovered recently)!
. could you explain this to me? 
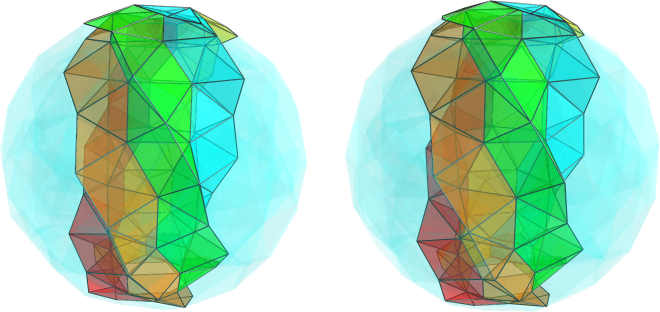
So what goes around, comes around. 
)