Klitzing wrote:Neither know whether I've writing access, nor how to locate all occurancies...quickfur wrote:[...] But maybe your terminology is more consistent. Would you mind updating the wiki to reflect this change?
(Thus it might be better, when someone would take hands on, who knows what to do... I might provide some guidance, if required, with respect to the contents.)
You just have to sign up for an account (it's separate from the forum account), and you'll have write access. To my knowledge, the only place where the term 'rotunda' is used is in the CRF polychora project page, maybe a few pages linked from that page. It will probably be helpful to dedicate another page to the terminology, so that we can put in the details/rationale, etc., and link to it from the main CRF page.
Florets have 2 rings. One clearly is the flower, a rose is an example of. The other is that of a rapier. And that obviously leads into the wrong direction.I was using the term 'florets' for these polychora, but I guess rosette works just as well, too.
[...]
Well, if it's two rings, then we just have to throw duoprism into the mix and it should all work out.

But OK, jokes aside, I guess rosette is a better term.
Also, an update on the bicyclodiminishings: it turns out that I did make a mistake -- or rather, there was a bug in one of my utilities that factors coordinates in terms of wendy's apacs/epacs operators, so that 10 vertices failed to be deleted from the original polychoron, thus causing the result to be non-CRF. I decided to bypass the factoring (it was just so the diminishing script I was writing can have nicely-factored forms instead of just a long list of coordinates), and managed to successfully get a CRF polychoron. So, allow me to introduce the bicyclopentadiminished o5x3o3o:

The yellow cells are truncated dodecahedra in one orthogonal ring (only 3 are visible because I left visibility clipping on, but there are 5 in total), and the green cells are truncated dodecahedra in the other orthogonal ring. So we have some kind of 5,5-duoprism symmetry here. Interestingly, the cells in the two rings are bridged by single tetrahedra, with the remaining gaps filled in by a fascinating alternating pattern of pentagonal rotundae plus more tetrahedra in between them. There are 310 cells in total; 10 truncated dodecahedra, 100 pentagonal rotundae, and 200 tetrahedra.
This is wonderful news!! It means that o5x3o3x should also have a bicyclopentadiminishing!



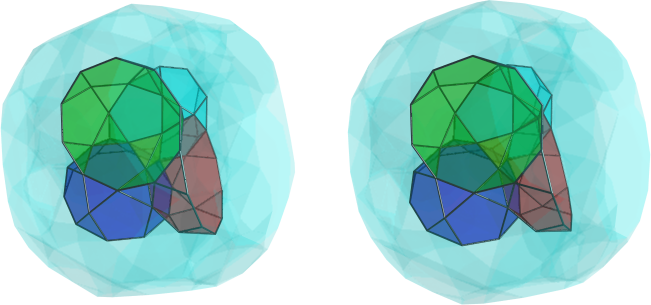


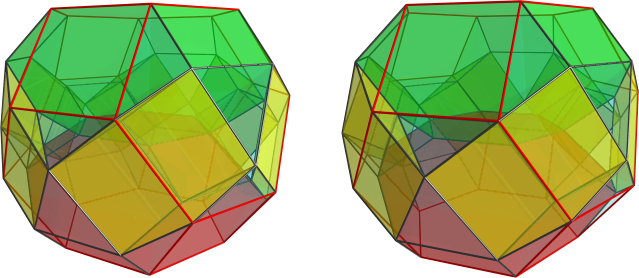




 )
) ). Depending on how many axis we contract, we can write 1-contracted, 2-contracted, etc..
). Depending on how many axis we contract, we can write 1-contracted, 2-contracted, etc..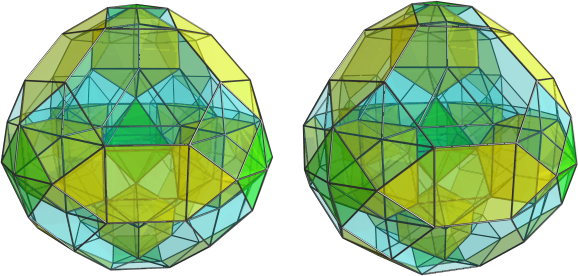
 What do you think about those proposed names? Any preference? Any other ideas? Perhaps there even might be some different construction devices? (@hedrondude: what about either of those proposed OBSAs?)
What do you think about those proposed names? Any preference? Any other ideas? Perhaps there even might be some different construction devices? (@hedrondude: what about either of those proposed OBSAs?)