gonegahgah wrote:There are so many levels to fathom that it is just mind blowing.
The reason I'm exploring rotation, primarily I hope, is in an attempt to understand 4D 'surfaces' to a greater degree.
Hopefully this need to understand 4D 'surfaces' will tie back to the archery example that I wish to explore for the purposes of understanding this poly-sidedness.
4D surfaces are basically 3D manifolds (a manifold is just an n-dimensional "cloth" that can be bent, flattened, twisted, etc.). In the case of flat objects like polytopes (the generalization of polygons & polyhedra to higher dimensions), the 4D surface consists of an assembly of polyhedra.
We may have trouble understanding how such surfaces work, but it helps to think of how a 2Der would have trouble understanding how surfaces in our 3D world work. To them, a 2D area occupies space, so they find it hard to grasp the concept of us 3D beings putting a cup on a table, say. How could you possibly put an object inside an area? It's already hard enough to understand how said object doesn't need to pass through the edges of the area, much less how it could possibly "rest on" that area.
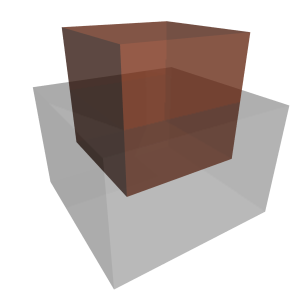
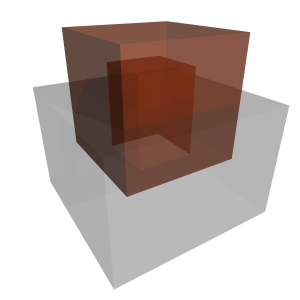
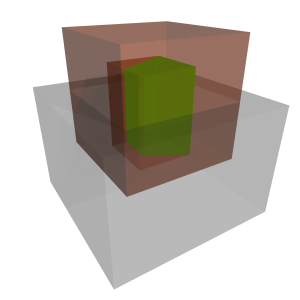
In 3D, of course, this is intuitively obvious. But we in turn have trouble understanding how a 3D volume can possibly act as a surface. How can one, for example, rest a tesseract on top of a large cubical surface? Where would the tesseract go in the volume of that surface? By dimensional analogy, we can understand that a cubical volume from the 4D perspective is a two-sided surface with a cube-shaped boundary. So the tesseract can sit upon one side of it, just as a cube in our world can sit upon a large square surface. And just as we tend to rest the cube roughly in the middle of the surface, such that the bottom face of the cube lies within the square boundary of the surface, so also a 4Der would tend to place a tesseract upon a cubical desk in such a way that its bottom cube rests within the cubical confines of the desk's surface.
Many such analogies can be drawn, which are quite helpful in grasping the concept of a 3D volume that behaves like a surface.
As an aside - but probably still relevant - one of the important aspects, I feel now, is the cross-conception across the dimensions of the modes of space.
By this I mean that 'volume', for example, is a concept greater than a straight definition of occupying 3D space as we are used to defining it.
Yes, this is what I was driving at in my earlier post, upon 4DSpace's request, on dimensional terminology. In a higher dimensional space, objects of particular dimensions have a different function than in our space. A 2D object in our world is a space-dividing surface, but in 4D, it is relegated to the role of a mere margin where two surfaces may meet. A 3D object in our world occupies space, but in 4D, it is relegated to being a mere surface where two objects may meet.
Entering the word 'volume' at
http://dictionary.reference.com has the relevant definition:
"The amount of space, measured in cubic units, that an object or substance occupies."
The key sense of this definition is: "the amount of space ... that an object or substance occupies."
It is simply measured in cubic units for us because our space has cubic proportions.
For a 4Der their space has quartic proportions so for a 4Der their 'volume' (or 4-volume) is measured in quartic units (tessaratic units).
Correct. And this is where it's helpful to distinguish between the
absolute number of dimensions of an object, and its number of dimensions
relative to the ambient space. The absolute number of dimensions is an inherent property of the object, and defines properties that are
attributive to the object. A cube has 3 dimensions, no matter how high or how low the dimensionality of the surrounding space. It will not cease to have 3D volume just because you put it in a higher-dimensional space. Its
function with respect to the surrounding space, though, depends on the relative difference between its number of dimensions and the dimensionality of the space.
A cube in 4D, for example, has a different function from a cube in 3D; in 3D, we may use cubes as building blocks to assemble 3D objects, say things like a Rubik's cube or some such. But in 4D, because of the presence of one more dimension in the surrounding space, the cube ceases to function as a building block, and becomes instead a surface. In that sense, it plays a role analogous to that of a polygon in 3D. In 3D, we may use polygons to assemble a polyhedral surface, for example -- 12 pentagons can be assembled to make the surface of a dodecahedron. In 4D, we use cubes and other polyhedra to make surfaces of 4D objects. None of this changes the absolute dimensionality of the cube or polyhedron -- a cube is still 3D, and a polygon is still 2D. It's just that their roles have changed because they compare differently to the dimensionality of the surrounding space.
So it's useful to have two different sets of terminology, one for describing absolute dimensionality, and another for describing relative dimensionality. Conflating the two only leads to confusion when one starts speaking of spaces of different dimensions. Wendy's Polygloss defines a consistent set of terminology that respects this difference, which is why it has utility even up to 6 to 8 dimensions. When we go up that high, our usual 3D-centric terminology utterly fails us, because it doesn't clearly differentiate between absolute dimensionality and relative dimensionality.
Maybe we should be calling a tesseract a quard (pronounced kword) (ie. square, cube, quard) instead of a tesseract? Too late now but it would follow the progression.
For a 2Der their space has squared proportions so for a 2Der their 'volume' (or 2-volume) is measured in squared units.
The mathematicians are way ahead of us.

The usual terminology for n-dimensional cubes is n-cube. So a 2-cube is a square, a 3-cube is what we think of as a cube, a 4-cube is a tesseract, and so on.
In any case, I'd like to restate some of the terms I defined in the post alluded to earlier:
Relative terminology (relative to a space of n dimensions):
A
facet is an (n-1)-dimensional polytope that serves as part of the surface of an n-dimensional object.
A
surface is an (n-1)-dimensional manifold (not necessarily flat) that serves as part of the boundary of an n-dimensional object.
A
ridge is an (n-2)-dimensional manifold where two surfaces meet. For example, two square faces in the cube meet at an edge, so the edge is a ridge of the cube. In higher dimensions, a 4-cube (i.e. tesseract) has cube-shaped facets that meet at squares -- thus, these squares are the 4-cube's ridges.
A
peak is an (n-3)-dimensional manifold where three or more ridges meet. For example, the cube's corners have three edges meeting at them, so these corners are the cube's peaks. A tesseract has three cubes that meet at an edge, so the edges of a tesseract are its peaks.
The
bulk of an object is the amount of n-dimensional space it occupies. Bulk is measured in terms of unit
n. For example, the bulk of a cube is a 3D quantity measured in, say, meters cubed. The bulk of a tesseract is a 4D quantity measured in, say, meters to the 4th power. The two are incompatible -- you can't compare meters cubed to meters to the 4th power, just as you can't compare meters squared to meters cubed. It's comparing apples and oranges.
Absolute terminology:
A
vertex is a 0-dimensional point that where other elements of an object meet. For example, a cube's corners are its vertices, and a tesseract's corners are its vertices. The cube's vertices happen to be the same as its peaks; but a tesseract's peaks are not the same as its vertices. A tesseract's peaks are its edges, which are 1 dimension higher than its vertices.
An
edge is a 1-dimensional line segment where other higher-dimensional elements of an object meet. A cube has 12 edges, for example, and a tesseract has 32 edges. Whereas a cube's edges are its ridges, a tesseract's edges are not ridges, but peaks.
A
face is a 2-dimensional polygon where other higher-dimensional elements of an object meet. (This one is a bit debatable, since "face" could be construed to be a better used for what I use "facet" for. But then most of existing terminology is debatable, so we just have to agree on which term to use and go with that.) A cube has 6 faces, which are the same as its facets. But a tesseract's faces are not its facets; they are merely its ridges.
A
cell is a 3-dimensional polyhedron where other higher-dimensional elements of an object meet. A cube's cell is the cube itself, but a tesseract's surface has 8 cells, each of which is a cube.
Length is the extent of a 1-dimensional object. For example, an edge's length is the Euclidean distance between its two vertices. Length is measured in units.
Area is the extent of a 2-dimensional object. It is measured in units
2.
Volume is the extent of a 3-dimensional object. It is measured in units
3. Even though we like to think of volume as the unit that fills space, I prefer to let it remain 3-dimensional, and use "bulk" to refer to the unit that fills n-dimensional space -- just so we minimize the confusion that arises when people see the word "volume" and think "units cubed".
If we want to be more precise, we may prefix the dimension number to designate a specific dimension. For example, 1-bulk = length; 2-bulk = area, 3-bulk = volume.
Same goes for the concept of face.
Entering the word 'face' at
http://dictionary.reference.com has the relevant defintion:
"The main side of an object, building, etc, or the front"
For uniform objects, like a cube, we refer to all the outward squares as faces because they are all the same and any of them could be looked at from front on.
So again for a 4Der the 'face' of a tesseract is any of its cube 'faces' and for a 2Der the 'face' of a square is any of its line 'faces'.
Hmm. I may have to retract my definition for "face" and use it to mean "facet". I think this is what Wendy did in her polygloss too -- face means that part of the object that's facing you, so there's no reason to fix it to 2D. So that's two votes against my definition of "face".

What do you think? Should we adopt Wendy's definition of "face" instead? We will have to find another term for 2D element, though, since we tend to refer to those a lot.
I think it is useful to parallel our thoughts to those of our 2Der and 4Der counterparts so that we can think more like them.
This may, or may not, assist us towards discovering the design of real world artifacts for our 4Der counterparts.
Hopefully it does and I feel it gives me a better sense of their lives. [...]
Absolutely. I have found, in the course of my learning to visualize 4D, that it's essential to think in terms of 3-bulk as surfaces and 2-bulk as ridges. As 3D beings, our natural tendency is to interpret 3-bulk as space-filling, and 2-bulk (area) as space-dividing surfaces, but that only leads to confusion when dealing with 4D objects. Once we wrap our mind around the idea of 3-bulk being only a surface, and 2-bulk being only a ridge, then we begin to have a true glimpse into the nature of the 4D world.
Then one begins to discover interesting things, such as the fact that 4Ders walk on floors that fill 3-bulk, and so have a much greater degree of freedom than we do. In your terms, gonegahgah, they have an additional 360° of sideways where they can move about. One also begins to note that a 4D room doesn't just have 4 walls and 4 corners; it has six walls and two different
kinds of corners: twelve edge-corners where two walls meet, and eight vertex-corners where three walls meet. (That's a lot of corners!) It took me a long time to wrap my head around how a room could possibly have three walls that meet at a corner. I used to play John McIntosh's 4D maze game, and in free-movement mode, where you have full 4D freedom to move about, I often got "stuck in a corner" and didn't know how to get out, because I didn't understand what I was seeing -- three cubes meeting at an edge -- and more specifically, what that meant in terms of my relative position/orientation relative to the room.
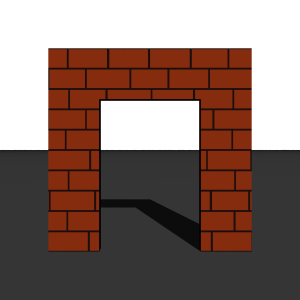
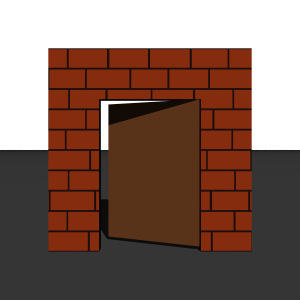
Their doors are not mere 2D panels of wood, but 3D "blocks" (which aren't actually blocks in 4D -- we just tend to think of them that way because of our 3D-centric understanding). Door hinges don't just lie along a vertical axis, and doors don't turn around an axis; they are hinged on a 2D ridge and turn around this ridge. Because of this, a door frame in 4D doesn't just have two sides with one side hinged to the door; 4D door frames have 4 sides, and the door can be hinged on any one of these 4 sides. Unlike in 3D, where the two doorposts are not connected to each other, the 4D door frame's 4 sides are rectangles that touch each other in a cuboidal arrangement. The locking mechanism would tend to be on the opposite ridge from where the door is hinged, since that's where the lock has maximum leverage to hold the door shut against its pivoting ridge. But you can also have auxiliary locking mechanisms on the other two ridges, which may serve as additional support to keep the locked door closed. We don't have direct equivalents of this in 3D.
Lots of other fun stuff can be discovered, once we get used to "thinking in terms of volumes", as I like to put it.