Number of CRF polychora

[quickfur]

Starting this as a separate thread, 'cos the main thread is seriously growing unmanageably long.

So, today I was updating my website (in my local version, not uploaded yet), and wrote a little history of CRF polychora. In the course of researching the history, I finally got around to reading Mathieu Sikirić, et al's paper that enumerates all non-adjacent diminishings of the 600-cell. These are diminishings that produce CRFs with regular cells (tetrahedra and icosahedra), and it turns out that their number, up to isomorphism, is a whopping 314,248,344. That's 314 million distinct diminishings of the 600-cell, and this doesn't even count the CRFs that involve adjacent diminishings (that produce things with J62, J63, or pentagonal antiprism cells)! Now, consider the subset of these 314 million non-adjacent diminishings, that will continue to yield a CRF if an adjacent diminishing was introduced. I'm pretty sure a significant subset have this property, so if we include adjacent diminishings (as long as they are CRF), the final count of 600-cell diminishings must far exceed the 314 million figure.

But this is only the beginning!!! Consider, for example, the runcinated 120-cell x5o3o3x. It admits diminishings where we cut off a dodecahedron||rhombicosidodecahedron. Now notice that if two dodecahedra correspond with non-adjacent 600-cell vertices, then they can be simultaneously diminished with a CRF result. This means there's a 1-to-1 correspondence between diminishings of the runcinated 120-cell with the non-adjacent diminishings of the 600-cell. Which means that there are (at least) 314 million diminishings of the runcinated 120-cell (!!).

This applies not just to the runcinated 120-cell; it applies to other 120-cell family uniforms too. The rectified 600-cell o5o3x3o, for example, admits diminishings by cutting off o5o3x||o5x3o, and again, positions corresponding to non-adjacent 600-cell vertices can be diminished, so there's a 1-to-1 correspondence with the 314 million non-adjacent diminishings of the 600-cell. AFAICT, the 120-cell family uniforms that admit analogous diminishings are: o5o3x3o, o5o3x3x, o5x3o3o, o5x3o3x, x5o3o3x, x5o3x3o, x5o3x3x, and x5x3o3x. This means that, including the 600-cell, the non-adjacent diminishings of these polychora give at least 2,828,235,096 CRFs. That's 2.8 billion CRFs!!!!

And remember, many of these polychora admit adjacent diminishings, as well as deeper diminishings, not to mention other modifications like pseudo-bisection, etc.. The 2 billion figure doesn't even include these!

And now consider how many of these diminishings happen in a localized-enough region, that we can apply bilbiro'ing and thawro'ing to them. Now if my conjecture is true, that all the BT polychora we found so far can be formed by assembling various patches of surface from the 120-cell family uniforms (with suitable modifications thereof), then the number of possible combinations truly boggles the mind!!

So, what do you think will be the final count of CRF polychora? The lower bound is now 2.8 billion. That's huuuge.

And of course, we shouldn't forget the duoprism augmentations. The 1633 augmentations that Marek & I found are only the beginning; there's at least 1633 more augmentations with 2n-prism||n-gons, which means the lower bound on duoprism augmentations is 3266, probably more, since there are some 2n-prism||n-gon augmentations that have no equivalent in the n-prism pyramid augmentations, and those that do also have gyrated variations. Anybody wanna take a stab at counting duoprisms in this latter category? (Yes I know, this looks like nothing compared to the 600-cell family diminishings -- 2 billion of them, probably many more -- but at least it's something tractible that we can actually achieve in the short term. )

Anyway. I'm hoping this thread can be the start of a more systematic attempt to count the CRF polychora. In the process, we may end up supplementing the main thread by identifying areas that haven't received adequate attention, that may potentially yield more interesting CRFs.

[quickfur]

Alright, here are some preliminary results: I wrote a program for enumerating duoprism augments, and found that the following duoprisms are augmentable by either some manner of n-prism pyramid, or n-gonal magnabicupolic ring (i.e. 2n-prism||n-gon):

3,3-duoprism: intra1=60.000° aug1=52.239° intra2=60.000° aug2=52.239°
3,4-duoprism: intra1=60.000° aug1=45.000° intra2=90.000° aug2=52.239°
3,5-duoprism: intra1=60.000° aug1=18.000° intra2=108.000° aug2=52.239°
3,6-duoprism: intra1=60.000° aug1=52.239° intra2=120.000° aug2=52.239°
3,8-duoprism: intra1=60.000° aug1=45.000°
3,10-duoprism: intra1=60.000° aug1=18.000°
4,4-duoprism: intra1=90.000° aug1=45.000° intra2=90.000° aug2=45.000°
4,5-duoprism: intra1=90.000° aug1=18.000° intra2=108.000° aug2=45.000°
4,6-duoprism: intra1=90.000° aug1=52.239° intra2=120.000° aug2=45.000°
4,7-duoprism: intra2=128.571° aug2=45.000°
4,8-duoprism: intra1=90.000° aug1=45.000° intra2=135.000° aug2=45.000°
4,10-duoprism: intra1=90.000° aug1=18.000°
5,5-duoprism: intra1=108.000° aug1=18.000° intra2=108.000° aug2=18.000°
5,6-duoprism: intra1=108.000° aug1=52.239° intra2=120.000° aug2=18.000°
5,7-duoprism: intra2=128.571° aug2=18.000°
5,8-duoprism: intra1=108.000° aug1=45.000° intra2=135.000° aug2=18.000°
5,9-duoprism: intra2=140.000° aug2=18.000°
5,10-duoprism: intra1=108.000° aug1=18.000° intra2=144.000° aug2=18.000°
5,11-duoprism: intra2=147.273° aug2=18.000°
5,12-duoprism: intra2=150.000° aug2=18.000°
5,13-duoprism: intra2=152.308° aug2=18.000°
5,14-duoprism: intra2=154.286° aug2=18.000°
5,15-duoprism: intra2=156.000° aug2=18.000°
5,16-duoprism: intra2=157.500° aug2=18.000°
5,17-duoprism: intra2=158.824° aug2=18.000°
5,18-duoprism: intra2=160.000° aug2=18.000°
5,19-duoprism: intra2=161.053° aug2=18.000°
5,20-duoprism: intra2=162.000° aug2=18.000°
6,6-duoprism: intra1=120.000° aug1=52.239° intra2=120.000° aug2=52.239°
6,8-duoprism: intra1=120.000° aug1=45.000°
6,10-duoprism: intra1=120.000° aug1=18.000°
7,8-duoprism: intra1=128.571° aug1=45.000°
7,10-duoprism: intra1=128.571° aug1=18.000°
8,8-duoprism: intra1=135.000° aug1=45.000° intra2=135.000° aug2=45.000°
8,10-duoprism: intra1=135.000° aug1=18.000°
9,10-duoprism: intra1=140.000° aug1=18.000°
10,10-duoprism: intra1=144.000° aug1=18.000° intra2=144.000° aug2=18.000°
10,11-duoprism: intra2=147.273° aug2=18.000°
10,12-duoprism: intra2=150.000° aug2=18.000°
10,13-duoprism: intra2=152.308° aug2=18.000°
10,14-duoprism: intra2=154.286° aug2=18.000°
10,15-duoprism: intra2=156.000° aug2=18.000°
10,16-duoprism: intra2=157.500° aug2=18.000°
10,17-duoprism: intra2=158.824° aug2=18.000°
10,18-duoprism: intra2=160.000° aug2=18.000°
10,19-duoprism: intra2=161.053° aug2=18.000°
10,20-duoprism: intra2=162.000° aug2=18.000°

Given an m,n-duoprism, the program regards the first ring as the one that has m n-prisms, and the second ring as the one that has n m-prisms.

intra1 and intra2 are the dichoral angles between two prisms in the two respective rings;
aug1 and aug2 give the intra-ring dichoral angles of the prospective augments.

Where one or the other set of angles are not shown, it means that the corresponding ring cannot be augmented -- either no such augment exists (e.g., the heptagonal prism has no known augments), or the augment is too tall and will make the result non-convex. (So for example, the 3,10-duoprism can be augmented only in its first ring, whereas the 4,7-duoprism can only be augmented in its second ring.)

I haven't gotten around to actually counting the number of distinct augmentations yet, but I thought I'd post the current results first since nobody is replying to this thread. But as you can see, duoprisms up to 10,20-duoprisms are augmentable, so duoprism augmentations do contribute significantly to the total number of CRFs!

[Keiji]

Err, is this anything new/different to CRFP4DP/Augmentations#Augmented_duoprisms..?

[quickfur]

Err, is this anything new/different to CRFP4DP/Augmentations#Augmented_duoprisms..?

Yes, because this one accounts for augmentations with magnabicupolic rings, whereas what is listed on that page only accounts for prism pyramid augments. (Yes I know it talks about magnabicupolic ring augments, but it doesn't actually count them, which is what I'm trying to do here.)

[quickfur]

Whoa. So, I got my program to the point where it's mostly working, and then I discover something astounding.

First of all, the preliminary results all point to the fact that allowing magnabicupolic ring augments does greatly increase the number of possible augmentations -- the 5,10-duoprism, for example, experiences a combinatorial explosion due to the fact that there are 22 possible augmentations of the decagonal prisms, and since all augments' inter-ring angles are very shallow, the two rings can be independently augmented in all possible combinations, so this single duoprism generates 1715 augmentations all on its own.

But that's only the tip of the iceberg. Something horrible happens when we get to the 10,10-duoprism: the pentagonal magnabicupolic ring augments are, again, very shallow, so both rings can be independently augmented. Furthermore, each augment has two distinct orientations, which greatly increases the number of combinations (now each prism has 3 possible states: unaugmented, ortho-augmented, and gyro-augmented). It's not quite 3^n, because some gyrations are isomorphic to others under the duoprism's symmetry group, but nevertheless, there is an absolutely humongous number of combinations here. Add to this the fact that the intra-ring angles are shallow enough for for adjacent decagonal prisms to be simultaneously augmented and still remain convex, and we have (up to) 3^10 * 3^10 combinations, divided by the size of the symmetry group of the 10,10-duoprism. Of course, the symmetry group is pretty big, but not very big compared to the sheer number of combinations. The final count of 10,10-duoprism CRF augmentations, if my program is correct, is 1,365,377, up to isomorphism(!!) (and not counting the unaugmented 10,10-duoprism).

And now I'm waiting for the program to finish counting the 10,19-duoprism augmentations -- which, fortunately, have no such horrible combinatorial explosion because the 19-gonal prisms cannot be augmented, and adjacent decagonal prisms cannot be simultaneously augmented due to the intra-ring angle being too large. However, the (dumb) algorithm I'm using is apparently hitting some lousy bottleneck, so it's taking much longer to count only 7000+ augmentations of the 10,19-duoprism than to count the 1.3 million augmentations of the 10,10-duoprism.

Anyway, here's the (partial) output so far:

Code: Select all

3,3-duoprism:
        3 augmentations
3,4-duoprism:
        5 augmentations
3,5-duoprism:
        11 augmentations
3,6-duoprism:
        9 augmentations
3,8-duoprism:
        5 augmentations
3,10-duoprism:
        5 augmentations
4,4-duoprism:
        20 augmentations
4,5-duoprism:
        17 augmentations
4,6-duoprism:
        7 augmentations
4,7-duoprism:
        4 augmentations
4,8-duoprism:
        103 augmentations
4,10-duoprism:
        12 augmentations
5,5-duoprism:
        35 augmentations
5,6-duoprism:
        51 augmentations
5,7-duoprism:
        17 augmentations
5,8-duoprism:
        119 augmentations
5,9-duoprism:
        45 augmentations
5,10-duoprism:
        1715 augmentations
5,11-duoprism:
        15 augmentations
5,12-duoprism:
        25 augmentations
5,13-duoprism:
        30 augmentations
5,14-duoprism:
        48 augmentations
5,15-duoprism:
        63 augmentations
5,16-duoprism:
        98 augmentations
5,17-duoprism:
        132 augmentations
5,18-duoprism:
        208 augmentations
5,19-duoprism:
        290 augmentations
5,20-duoprism:
        454 augmentations
6,6-duoprism:
        7 augmentations
6,8-duoprism:
        7 augmentations
6,10-duoprism:
        51 augmentations
7,8-duoprism:
        8 augmentations
7,10-duoprism:
        105 augmentations
8,8-duoprism:
        170 augmentations
8,10-duoprism:
        265 augmentations
9,10-duoprism:
        629 augmentations
10,10-duoprism:
        1365377 augmentations
10,11-duoprism:
        62 augmentations
10,12-duoprism:
        121 augmentations
10,13-duoprism:
        189 augmentations
10,14-duoprism:
        361 augmentations
10,15-duoprism:
        611 augmentations
10,16-duoprism:
        1161 augmentations
10,17-duoprism:
        2055 augmentations
10,18-duoprism:
        3913 augmentations
10,19-duoprism:
        7154 augmentations


There are still two more augmentable duoprisms not yet counted: 10,19-duoprism and 10,20-duoprism, both of which are in progress...

CAVEAT: I'm not 100% sure the counts are exact, because I haven't excluded the possibility of bugs in the counting algorithm. The 4,4-duoprism counts are definitely wrong, because it fails to take tesseractic symmetry into account; and I suspect the 8,8-duoprism counts may also be slightly off for the same reason (since the fully augmented 8,8-duoprism is just the cantellated tesseract x4o3x3o). But I'm pretty confident about the other counts.

You can see the sudden leap in the count going from 9,10-duoprism to 10,10-duoprism: because the 9,10-duoprism can only be augmented in a single ring. Adding 1 to the size of the ring obviously greatly increases the number of combinations in that single ring (since it's exponential), but now both rings can be augmented so the count gets squared. Immediately after, the 10,11-duoprism no longer allows both rings to be augmented (there is no known CRF augmentation of an 11-gonal prism), and adjacent augments are also no longer allowed (the 10,10-duoprism is the last case where adjacent augments are possible: they in fact have cells coplanar with the adjacent augments' cells, so they would merge into pentagonal (gyro/ortho)bicupolae). So the count drops back to a mere 62.

Would somebody else like to attempt counting these duoprism augmentations, so that we can double-check our results? I.e., write a program to do it -- originally, I was going to post the list of all canonical augmentations here so that others can review it for mistakes, but the fact that there 1.3 million augmentations of the 10,10-duoprism kinda puts a damper on that.

EDIT: the count for the 10,19-duoprism is out, at 7164 augmentations. I must say, I knew there would be more than just the paltry 1633 augmentations with prism pyramids, but I had no idea there would be this many more!!

[quickfur]

Well finally the program finished enumerating the 13647 augmentations of the 10,20-duoprism... just as I realized that there was a bug in the counting algorithm. So I'm going to fix the algorithm and run it through again.

It turns out, however, that the bug probably caused the program to miss some distinct augmentations, so the numbers I posted should be lower bounds. Meaning that the 10,10-duoprism may turn out to have more augmentations than the 1.4 million found so far!

[quickfur]

Alright, I've fixed the bug in my program (hopefully that's the last of 'em...). The output now is:

Code: Select all

3,3-duoprism:
   ring 1 intra: 60.000°   aug=triangular prism pyramid
      aug intra 52.239° inter +65.905° x48.190°
      adj_augs
   ring 2 intra: 60.000°   aug=triangular prism pyramid
      aug intra 52.239° inter +65.905° x48.190°
      adj_augs
   can only augment one ring at a time
   3 augmentations

3,4-duoprism:
   ring 1 intra: 60.000°   aug=cubical pyramid
      aug intra 45.000° inter +45.000° x35.264°
      adj_augs
   ring 2 intra: 90.000°   aug=triangular prism pyramid
      aug intra 52.239° inter +65.905° x48.190°
      no_adj
   can only augment one ring at a time
   5 augmentations

3,5-duoprism:
   ring 1 intra: 60.000°   aug=pentagonal prism pyramid
      aug intra 18.000° inter +13.283° x10.812°
      adj_augs
   ring 2 intra: 108.000°   aug=triangular prism pyramid
      aug intra 52.239° inter +65.905° x48.190°
      no_adj
   can augment both rings simultaneously
   11 augmentations

3,6-duoprism:
   ring 1 intra: 60.000°   aug=triangular magnabicupolic ring
      aug intra 52.239° inter +65.905° x48.190°
      adj_augs has_gyro
   ring 2 intra: 120.000°   aug=triangular prism pyramid
      aug intra 52.239° inter +65.905° x48.190°
      no_adj
   can only augment one ring at a time
   9 augmentations

3,8-duoprism:
   ring 1 intra: 60.000°   aug=square magnabicupolic ring
      aug intra 45.000° inter +45.000° x35.264°
      adj_augs has_gyro
   ring 2 intra: 135.000°   no augs
   can only augment one ring at a time
   5 augmentations

3,10-duoprism:
   ring 1 intra: 60.000°   aug=pentagonal magnabicupolic ring
      aug intra 18.000° inter +13.283° x10.812°
      adj_augs has_gyro
   ring 2 intra: 144.000°   no augs
   can only augment one ring at a time
   5 augmentations

4,4-duoprism:
   ring 1 intra: 90.000°   aug=cubical pyramid
      aug intra 45.000° inter +45.000° x35.264°
      adj_augs
   ring 2 intra: 90.000°   aug=cubical pyramid
      aug intra 45.000° inter +45.000° x35.264°
      adj_augs
   can augment both rings simultaneously
   20 augmentations

4,5-duoprism:
   ring 1 intra: 90.000°   aug=pentagonal prism pyramid
      aug intra 18.000° inter +13.283° x10.812°
      adj_augs
   ring 2 intra: 108.000°   aug=cubical pyramid
      aug intra 45.000° inter +45.000° x35.264°
      no_adj
   can augment both rings simultaneously
   17 augmentations

4,6-duoprism:
   ring 1 intra: 90.000°   aug=triangular magnabicupolic ring
      aug intra 52.239° inter +65.905° x48.190°
      no_adj has_gyro
   ring 2 intra: 120.000°   aug=cubical pyramid
      aug intra 45.000° inter +45.000° x35.264°
      no_adj
   can only augment one ring at a time
   7 augmentations

4,7-duoprism:
   ring 1 intra: 90.000°   no augs
   ring 2 intra: 128.571°   aug=cubical pyramid
      aug intra 45.000° inter +45.000° x35.264°
      no_adj
   can only augment one ring at a time
   4 augmentations

4,8-duoprism:
   ring 1 intra: 90.000°   aug=square magnabicupolic ring
      aug intra 45.000° inter +45.000° x35.264°
      adj_augs has_gyro
   ring 2 intra: 135.000°   aug=cubical pyramid
      aug intra 45.000° inter +45.000° x35.264°
      no_adj
   can augment both rings simultaneously
   123 augmentations

4,10-duoprism:
   ring 1 intra: 90.000°   aug=pentagonal magnabicupolic ring
      aug intra 18.000° inter +13.283° x10.812°
      adj_augs has_gyro
   ring 2 intra: 144.000°   no augs
   can only augment one ring at a time
   12 augmentations

5,5-duoprism:
   ring 1 intra: 108.000°   aug=pentagonal prism pyramid
      aug intra 18.000° inter +13.283° x10.812°
      adj_augs
   ring 2 intra: 108.000°   aug=pentagonal prism pyramid
      aug intra 18.000° inter +13.283° x10.812°
      adj_augs
   can augment both rings simultaneously
   35 augmentations

5,6-duoprism:
   ring 1 intra: 108.000°   aug=triangular magnabicupolic ring
      aug intra 52.239° inter +65.905° x48.190°
      no_adj has_gyro
   ring 2 intra: 120.000°   aug=pentagonal prism pyramid
      aug intra 18.000° inter +13.283° x10.812°
      adj_augs
   can augment both rings simultaneously
   64 augmentations

5,7-duoprism:
   ring 1 intra: 108.000°   no augs
   ring 2 intra: 128.571°   aug=pentagonal prism pyramid
      aug intra 18.000° inter +13.283° x10.812°
      adj_augs
   can only augment one ring at a time
   17 augmentations

5,8-duoprism:
   ring 1 intra: 108.000°   aug=square magnabicupolic ring
      aug intra 45.000° inter +45.000° x35.264°
      no_adj has_gyro
   ring 2 intra: 135.000°   aug=pentagonal prism pyramid
      aug intra 18.000° inter +13.283° x10.812°
      adj_augs
   can augment both rings simultaneously
   166 augmentations

5,9-duoprism:
   ring 1 intra: 108.000°   no augs
   ring 2 intra: 140.000°   aug=pentagonal prism pyramid
      aug intra 18.000° inter +13.283° x10.812°
      adj_augs
   can only augment one ring at a time
   45 augmentations

5,10-duoprism:
   ring 1 intra: 108.000°   aug=pentagonal magnabicupolic ring
      aug intra 18.000° inter +13.283° x10.812°
      adj_augs has_gyro
   ring 2 intra: 144.000°   aug=pentagonal prism pyramid
      aug intra 18.000° inter +13.283° x10.812°
      adj_augs
   can augment both rings simultaneously
   3013 augmentations

5,11-duoprism:
   ring 1 intra: 108.000°   no augs
   ring 2 intra: 147.273°   aug=pentagonal prism pyramid
      aug intra 18.000° inter +13.283° x10.812°
      no_adj
   can only augment one ring at a time
   15 augmentations

5,12-duoprism:
   ring 1 intra: 108.000°   no augs
   ring 2 intra: 150.000°   aug=pentagonal prism pyramid
      aug intra 18.000° inter +13.283° x10.812°
      no_adj
   can only augment one ring at a time
   25 augmentations

5,13-duoprism:
   ring 1 intra: 108.000°   no augs
   ring 2 intra: 152.308°   aug=pentagonal prism pyramid
      aug intra 18.000° inter +13.283° x10.812°
      no_adj
   can only augment one ring at a time
   30 augmentations

5,14-duoprism:
   ring 1 intra: 108.000°   no augs
   ring 2 intra: 154.286°   aug=pentagonal prism pyramid
      aug intra 18.000° inter +13.283° x10.812°
      no_adj
   can only augment one ring at a time
   48 augmentations

5,15-duoprism:
   ring 1 intra: 108.000°   no augs
   ring 2 intra: 156.000°   aug=pentagonal prism pyramid
      aug intra 18.000° inter +13.283° x10.812°
      no_adj
   can only augment one ring at a time
   63 augmentations

5,16-duoprism:
   ring 1 intra: 108.000°   no augs
   ring 2 intra: 157.500°   aug=pentagonal prism pyramid
      aug intra 18.000° inter +13.283° x10.812°
      no_adj
   can only augment one ring at a time
   98 augmentations

5,17-duoprism:
   ring 1 intra: 108.000°   no augs
   ring 2 intra: 158.824°   aug=pentagonal prism pyramid
      aug intra 18.000° inter +13.283° x10.812°
      no_adj
   can only augment one ring at a time
   132 augmentations

5,18-duoprism:
   ring 1 intra: 108.000°   no augs
   ring 2 intra: 160.000°   aug=pentagonal prism pyramid
      aug intra 18.000° inter +13.283° x10.812°
      no_adj
   can only augment one ring at a time
   208 augmentations

5,19-duoprism:
   ring 1 intra: 108.000°   no augs
   ring 2 intra: 161.053°   aug=pentagonal prism pyramid
      aug intra 18.000° inter +13.283° x10.812°
      no_adj
   can only augment one ring at a time
   290 augmentations

5,20-duoprism:
   ring 1 intra: 108.000°   no augs
   ring 2 intra: 162.000°   aug=pentagonal prism pyramid
      aug intra 18.000° inter +13.283° x10.812°
      no_adj
   can only augment one ring at a time
   454 augmentations

6,6-duoprism:
   ring 1 intra: 120.000°   aug=triangular magnabicupolic ring
      aug intra 52.239° inter +65.905° x48.190°
      no_adj has_gyro
   ring 2 intra: 120.000°   aug=triangular magnabicupolic ring
      aug intra 52.239° inter +65.905° x48.190°
      no_adj has_gyro
   can only augment one ring at a time
   7 augmentations

6,8-duoprism:
   ring 1 intra: 120.000°   aug=square magnabicupolic ring
      aug intra 45.000° inter +45.000° x35.264°
      no_adj has_gyro
   ring 2 intra: 135.000°   no augs
   can only augment one ring at a time
   7 augmentations

6,10-duoprism:
   ring 1 intra: 120.000°   aug=pentagonal magnabicupolic ring
      aug intra 18.000° inter +13.283° x10.812°
      adj_augs has_gyro
   ring 2 intra: 144.000°   no augs
   can only augment one ring at a time
   51 augmentations

7,8-duoprism:
   ring 1 intra: 128.571°   aug=square magnabicupolic ring
      aug intra 45.000° inter +45.000° x35.264°
      no_adj has_gyro
   ring 2 intra: 135.000°   no augs
   can only augment one ring at a time
   8 augmentations

7,10-duoprism:
   ring 1 intra: 128.571°   aug=pentagonal magnabicupolic ring
      aug intra 18.000° inter +13.283° x10.812°
      adj_augs has_gyro
   ring 2 intra: 144.000°   no augs
   can only augment one ring at a time
   105 augmentations

8,8-duoprism:
   ring 1 intra: 135.000°   aug=square magnabicupolic ring
      aug intra 45.000° inter +45.000° x35.264°
      no_adj has_gyro
   ring 2 intra: 135.000°   aug=square magnabicupolic ring
      aug intra 45.000° inter +45.000° x35.264°
      no_adj has_gyro
   can augment both rings simultaneously
   416 augmentations

8,10-duoprism:
   ring 1 intra: 135.000°   aug=pentagonal magnabicupolic ring
      aug intra 18.000° inter +13.283° x10.812°
      adj_augs has_gyro
   ring 2 intra: 144.000°   no augs
   can only augment one ring at a time
   265 augmentations

9,10-duoprism:
   ring 1 intra: 140.000°   aug=pentagonal magnabicupolic ring
      aug intra 18.000° inter +13.283° x10.812°
      adj_augs has_gyro
   ring 2 intra: 144.000°   no augs
   can only augment one ring at a time
   629 augmentations

10,10-duoprism:
   ring 1 intra: 144.000°   aug=pentagonal magnabicupolic ring
      aug intra 18.000° inter +13.283° x10.812°
      adj_augs has_gyro
   ring 2 intra: 144.000°   aug=pentagonal magnabicupolic ring
      aug intra 18.000° inter +13.283° x10.812°
      adj_augs has_gyro
   can augment both rings simultaneously
   11921273 augmentations

10,11-duoprism:
   ring 1 intra: 144.000°   no augs
   ring 2 intra: 147.273°   aug=pentagonal magnabicupolic ring
      aug intra 18.000° inter +13.283° x10.812°
      no_adj has_gyro
   can only augment one ring at a time
   62 augmentations

10,12-duoprism:
   ring 1 intra: 144.000°   no augs
   ring 2 intra: 150.000°   aug=pentagonal magnabicupolic ring
      aug intra 18.000° inter +13.283° x10.812°
      no_adj has_gyro
   can only augment one ring at a time
   121 augmentations

10,13-duoprism:
   ring 1 intra: 144.000°   no augs
   ring 2 intra: 152.308°   aug=pentagonal magnabicupolic ring
      aug intra 18.000° inter +13.283° x10.812°
      no_adj has_gyro
   can only augment one ring at a time
   189 augmentations

10,14-duoprism:
   ring 1 intra: 144.000°   no augs
   ring 2 intra: 154.286°   aug=pentagonal magnabicupolic ring
      aug intra 18.000° inter +13.283° x10.812°
      no_adj has_gyro
   can only augment one ring at a time
   361 augmentations

10,15-duoprism:
   ring 1 intra: 144.000°   no augs
   ring 2 intra: 156.000°   aug=pentagonal magnabicupolic ring
      aug intra 18.000° inter +13.283° x10.812°
      no_adj has_gyro
   can only augment one ring at a time
   611 augmentations

10,16-duoprism:
   ring 1 intra: 144.000°   no augs
   ring 2 intra: 157.500°   aug=pentagonal magnabicupolic ring
      aug intra 18.000° inter +13.283° x10.812°
      no_adj has_gyro
   can only augment one ring at a time
   1161 augmentations

10,17-duoprism:
   ring 1 intra: 144.000°   no augs
   ring 2 intra: 158.824°   aug=pentagonal magnabicupolic ring
      aug intra 18.000° inter +13.283° x10.812°
      no_adj has_gyro
   can only augment one ring at a time
   2055 augmentations

10,18-duoprism:
   ring 1 intra: 144.000°   no augs
   ring 2 intra: 160.000°   aug=pentagonal magnabicupolic ring
      aug intra 18.000° inter +13.283° x10.812°
      no_adj has_gyro
   can only augment one ring at a time
   3913 augmentations

10,19-duoprism:
   ring 1 intra: 144.000°   no augs
   ring 2 intra: 161.053°   aug=pentagonal magnabicupolic ring
      aug intra 18.000° inter +13.283° x10.812°
      no_adj has_gyro
   can only augment one ring at a time
   7154 augmentations

10,20-duoprism:
   ring 1 intra: 144.000°   no augs
   ring 2 intra: 162.000°   aug=pentagonal magnabicupolic ring
      aug intra 18.000° inter +13.283° x10.812°
      no_adj has_gyro
   can only augment one ring at a time
   13647 augmentations

Total number of duoprism augmentations: 11956959


EDIT: a brief explanation of some items in the above output: the top few lines of each duoprism's entry describes what kind of augments are possible (or not), as well as some characteristics of those augments. The "intra" angle is the dichoral angle between the prism cell of the augment and the lacing cell that lies in the plane of the duoprism ring it will augment: basically, the sum of this angle with the inner angle B of the n-gon (also indicated by "intra" following the ring number) must be ≤180°, otherwise it will produce a non-convex result. Furthermore, if the "intra" angle A is small enough that 2A + B ≤ 180°, then two adjacent prisms can be simultaneously augmented while remaining convex. This is indicated by "has_adj" in the next line. If this is not true, then "no_adj" is shown instead. The "inter" angles describe the dichoral angles of the two kinds of lacing surtopes of the augments: the one prefixed with "+" is ortho component, which for the n-magnabicupolic ring is the angle between the 2n-gonal prism and the triangular prisms; the one prefixed with "x" is the gyro component, which is the angle between the 2n-gonal prism and the square pyramids. These angles determine whether or not you can simultaneously augment both rings of the duoprism: if the sum of the corresponding "inter" angles of two augments lying in different rings exceeds 90°, then the result is non-convex. So if the "inter" angle of a given augment is too large, then it precludes any augments on the other ring (N.B. an augment in the one ring is adjacent to all members of the orthogonal ring, so it just takes one augment with too large an "inter" angle to force the other ring to be unaugmented). Two "inter" angles are given, because depending on whether an augment is ortho or gyro, a second augment in the orthogonal ring may "see" a different "inter" angle depending on where it's placed. The program does take the orientation of the augments into account when computing the convexity of the "inter" angles, but I don't know if there's actually a case where an ortho augment would allow simultaneous augmentation whereas a gyro augment doesn't, or vice versa. Given the small set of possible augments we're dealing with, it's likely that this never actually makes a difference. But the program does handle it correctly, if it does happen. The "has_gyro" tag means that the augment can be gyrated in-place, or IOW, it can be placed on the duoprism in two distinct orientations. This basically applies to all magnabicupolic rings.

It took more than 6 hours on an 800MHz CPU to finish enumerating all of these augmentations. However, I haven't fixed the problem with the 4,4-duoprism augmentations yet (it still doesn't account for the extra symmetries of the tesseract), but I'm just gonna turn a blind eye for now, since we already know the augmentations of the tesseract very well. I'm still not 100% confident on the count for the 8,8-duoprism, but I'm pretty confident about the others.

As it turns out, the 10,10-duoprism actually has 11,921,273 augmentations (that's 11.9 million!), which is several times more than the previous count. The reason for this is that the bug in the previous version of the program failed to account for the fact that the rings of the duoprism cannot be rotated independently of each other: a rotation in one ring causes a gyration in the other, and vice versa. Furthermore, some augmentations are chiral, so they contribute 2 to the count rather than just 1 -- they cannot be transformed to each other via rotations only; they require a reflection. There's also a slight reduction in the count because the previous version didn't check the full rotational symmetry of the duoprism, so some combinations were duplicated.

However, after accounting for all these issues, there is a net increase in the number of augmentations. The total number of CRF duoprism augmentations with n-prism pyramids and n-gonal magnabicupolic rings, therefore, stands at 11,956,959. More than 99.7% of these augmentations are from the 10,10-duoprism, which just happened to have the right shape for two simultaneous sets of gyratible augments, causing a massive combinatorial explosion of augmentations.

The second place is held by the 10,20-duoprism with 13647 augmentations: pretty impressive considering that these are all augmentations of a single ring (the other ring, consisting of icosagonal prisms, cannot be augmented), and that non-adjacent augments are not possible. It basically corresponds with a partial Stott-expansion of the single-ring augmentations of the 10,10-duoprism. Conversely, if we start with the augmentations of the 10,20-duoprism, which have augments on only one ring, we can map them back to corresponding augmentations of a single ring of the 10,10-duoprism. Since the other ring of the 10,10-duoprism can be independently augmented, we have an upper limit of 13647^2 augmentations of the 10,10-duoprism, which is 186.2 million combinations, but due to the additional symmetry of the 10,10-duoprism (the two rings can be interchanged, unlike the 10,20-duoprism), and also the equivalence of certain sets of augmentations under ring rotation/gyration, the actual number of distinct augmentations drops to 11.9 million.

The third and fourth places are held by the 10,19-duoprism and the 10,18-duoprism, respectively, with counts of 7154 and 3913, showing the power of combinatorial explosion when you have an 18-member ring to augment (even when adjacent augments are not allowed -- the extra freedom in odd/even spacings between augments make their counts far more than the 10,9- and 10,8-duoprisms, where adjacent augments are allowed).

The fifth place is held not by the 10,17-duoprism, which "only" has 2055 augmentations; that honor goes to the 5,10-duoprism with 3013 augmentations. The 5,10-duoprism corresponds with the (partial) Stott contraction of the 10,10-duoprism, and shares many similarities such as both rings being simultaneously augmentable, adjacent augments being allowed on both rings, and the ring of decagonal prisms having distinct gyro augmentations. The restriction to non-gyro augmentations for the ring of pentagonal prisms, and the lack of equivalents to the 10,10-duoprism's oddly-spaced augmentations, greatly reduce the number of possible augmentations here. Nevertheless, it does show us that the 11.9 million augmentations of the 10,10-duoprism has a precedent: the neighbours of the 5,10-duoprism, the 5,9-duoprism and the 5,11-duoprism, have only 45 and 15 augmentations respectively (the count reduction in the 5,11-duoprism is due to the fact that adjacent augments are no longer possible past a 10-membered ring), whereas the 5,10-duoprism sticks out like a sore thumb with a whopping 3013 augmentations. Its counterpart, the 10,10-duoprism, far outdoes this, of course, standing at 11.9 million while the 9,10-duoprism and the 10,11-duoprism only have 629 and 62 augmentations each. (Again, the drastic reduction in the 10,11-duoprism is due to adjacent augments being no longer possible compared to the 9,10-duoprism.)

All of these counts far exceed the counts of augmentations with only n-prism pyramids, the largest of which stood at the 454 augmentations of the 5,20-duoprism. As I said, I knew that there would be many more augmentations once we let the magnabicupolic rings into the mix, but I never suspected it would be this many! I didn't expect that the duoprism augmentations would actually come close to challenging the number of non-adjacent 600-cell diminishings (around 300 million, as I said at the beginning of this topic). But, if the behaviour of the 10,10-duoprism is anything to go by, I suspect the number of 600-cell diminishings will explode to far huger numbers once we allow adjacent diminishings. Looking at the differences between the 5,10-duoprism, 10,10-duoprism, and 10,20-duoprism, it would suggest that we should not underestimate the power of combinatorial explosion even with such an apparently trivial change as allowing adjacent diminishings vs. only non-adjacent diminishings. For all we know, that could easily add several orders of magnitude (or more!) to the count of 600-cell diminishings.

Anyway, I'd really appreciate it now if somebody would step up to verify the numbers I've gotten -- even though I'm now reasonably confident about their accuracy (except for the 4,4-duoprism and the 8,8-duoprism, where extra symmetries are (potentially) involved), I still have this nagging feeling that perhaps there's still a bug somewhere else in my program that's throwing off the counts. So I'd like independent confirmation before taking these numbers as fact.

[Deedlit]

To bring the topic back to diminishings of the 600-cell, I was thinking of taking up the project of enumerating these. But first, I have a question: for a diminishing, can we take any subset of the vertices such that the connected components are either isolated vertices or a pair of adjacent vertices? Or does diminishing a pair of adjacent vertices remove the possibility of some vertices not adjacent to the pair?

[Klitzing]

To bring the topic back to diminishings of the 600-cell, I was thinking of taking up the project of enumerating these. But first, I have a question: for a diminishing, can we take any subset of the vertices such that the connected components are either isolated vertices or a pair of adjacent vertices? Or does diminishing a pair of adjacent vertices remove the possibility of some vertices not adjacent to the pair?

Well, there is a paper with title / authors / abstract

THE SPECIAL CUTS OF THE 600-CELL

Mathieu Dutour Sikirić, Wendy Myrvold

Abstract. A polytope is called regular-faced if every one of its
facets is a regular polytope. The 4-dimensional regular-faced polytopes
were determined by G. Blind and R. Blind [2, 3, 4]. The last
class of such polytopes is the one which consists of polytopes obtained
by removing a set of non-adjacent vertices (an independent
set) of the 600-cell. These independent sets are enumerated up to
isomorphism and it is determined that the number of polytopes in
this last class is 314, 248, 344.

(Submitted on 25 Aug 2007 (v1), last revised 22 Nov 2007 (this version, v2))

There is web access to that on too, cf. either
http://arxiv.org/abs/0708.3443v2 or
http://www.academia.edu/745766/The_special_cuts_of_600-cell.

--- rk

[quickfur]

Has anybody else confirmed my findings for the duoprism augmentations? It would be nice to have some independent confirmation, since I can't be 100% sure my program is bug-free.

[Deedlit]

To bring the topic back to diminishings of the 600-cell, I was thinking of taking up the project of enumerating these. But first, I have a question: for a diminishing, can we take any subset of the vertices such that the connected components are either isolated vertices or a pair of adjacent vertices? Or does diminishing a pair of adjacent vertices remove the possibility of some vertices not adjacent to the pair?

Well, there is a paper with title / authors / abstract

THE SPECIAL CUTS OF THE 600-CELL

Mathieu Dutour Sikirić, Wendy Myrvold

Abstract. A polytope is called regular-faced if every one of its
facets is a regular polytope. The 4-dimensional regular-faced polytopes
were determined by G. Blind and R. Blind [2, 3, 4]. The last
class of such polytopes is the one which consists of polytopes obtained
by removing a set of non-adjacent vertices (an independent
set) of the 600-cell. These independent sets are enumerated up to
isomorphism and it is determined that the number of polytopes in
this last class is 314, 248, 344.

(Submitted on 25 Aug 2007 (v1), last revised 22 Nov 2007 (this version, v2))

There is web access to that on too, cf. either
http://arxiv.org/abs/0708.3443v2 or
http://www.academia.edu/745766/The_special_cuts_of_600-cell.

--- rk

I know, but that paper only counted cuts of non-adjacent vertices. I was looking into counting the number of ways to cut a set consisting of nonadjacent groups, where each group is either a single vertex or a pair of adjacent vertices. But first I wanted to confirm that this always led to a CRF polychora.

[Klitzing]

I know, but that paper only counted cuts of non-adjacent vertices. I was looking into counting the number of ways to cut a set consisting of nonadjacent groups, where each group is either a single vertex or a pair of adjacent vertices. But first I wanted to confirm that this always led to a CRF polychora.

To answer this question of yours the folowing lace city display of the hexacosachoron provides answers:

Code: Select all

                 o5o           o5o                 
                        o5x                       
                                                  
            x5o                     x5o           
     o5o                                   o5o     
                        f5o                       
                 o5f           o5f                 
                                                  
     o5x                                   o5x     
            f5o                     f5o           
                                                  
o5o                     x5x                     o5o
                                                  
            o5f                     o5f           
     x5o                                   x5o     
                                                  
                 f5o           f5o                 
                        o5f                       
     o5o                                   o5o     
            o5x                     o5x           
                                                  
                        x5o                       
                 o5o           o5o                 


That is, the facet underneath a vertex (o5o) is the multistratic stack (lace tower) o5o || x5o || o5x || o5o, aka the icosahedron. Obviously, when diminishing 2 neighbouring vertices, these icosahedra would intersect, thereby diminishing themselves in turn. A such diminished icosahedron x5o || o5x || o5o is known as the gyroelongated pentagonal pyramid, J11.

Other diminishings of the icosahedron, which still contain its center, are the pentagonal antiprism (aka parabidiminishing), the metabidiminished icosahedron (J62), and the tridiminished icosahedron (J63). But note, that in all these diminishings of the icosahedron the chosen vertices are to be taken non-adjacent. Else the facets underneath, the pentagons, would get diminished in turn, then resulting in golden trapezia or even higher diminishings. But all of these would require for other edge lengths than unity.

That is, when aiming for CRFs (convex regular faced polychora) rather than just for CRHs (convex regular hedrated polychora) the to be diminished vertices, say "a" and "b" of the hexadecachoron might well be situated adjacently. But whenever one vertex "a" has a further such vertex "b₁" being situated adjacently, then any further to be diminished adjacent vertex "b₂" has to be situated non-adjacent with respect to the other such selected direct neighbour(s), i.e. to "b₁".

Stated differntly, whenever your potential diminishing of the hexacosachoron includes at least a subset of 3 to be cut off vertices, then these ought not form a unit edged triangle.

--- rk

[Mrrl]

4 years ago I've estimated number of diminishings of 600-cell as 2^100 or more: viewtopic.php?f=32&t=1468&start=180#p16730
Not sure that I can recall this construction now, but I can try.

[quickfur]

I would not be surprised if the actual number of 600-cell CRF diminishings are in the vicinity of 2^100 or so. Besides the "surface" diminishings, which include the adjacent and non-adjacent diminishings, there are also the deeper cuts, producing dodecahedral cross-sections (flanked by pentagonal pyramids) and icosidodecahedral cross-sections (also flanked by pentagonal pyramids). It's possible to make multiple such cuttings on the 600-cell, and the resulting shapes may also have their tetrahedral sections diminished combinatorially too. Besides that, there are also the 600-cell wedges, produced by two non-parallel bisections of the 600-cell, which I've posted before; each of these wedges also have a large number of possible diminishings.

Then there are the special cuts of the 600-cell, which involve deleting sets of vertices that may not be immediately obviously CRF, that produce a good number of the CRFs we found with J91 and J92 cells. IIRC, student95 found a way of obtaining my J92 rhombochoron from a diminishing of the 600-cell. I surmise that many other non-orbiform CRFs may be constructible from (non-CRF) 600-cell fragments by augmentation with one or two vertices.

So we're looking at a veritable gold mine of CRFs just from the 600-cell alone, and of course many of these constructions will have analogs in the other 600-cell family uniform polychora, making the number of CRFs even huger.

[student91]

I would not be surprised if the actual number of 600-cell CRF diminishings are in the vicinity of 2^100 or so. Besides the "surface" diminishings, which include the adjacent and non-adjacent diminishings, there are also the deeper cuts, producing dodecahedral cross-sections (flanked by pentagonal pyramids) and icosidodecahedral cross-sections (also flanked by pentagonal pyramids). It's possible to make multiple such cuttings on the 600-cell, and the resulting shapes may also have their tetrahedral sections diminished combinatorially too. Besides that, there are also the 600-cell wedges, produced by two non-parallel bisections of the 600-cell, which I've posted before; each of these wedges also have a large number of possible diminishings.

Hello there
I've not been very active here lately, because I have started studying, but now that the exams are around I have found some time to look into this a bit deeper.
All diminishings you've described in the quoted section can be created by taking a subset of the 600-cell's vertices, and cutting the other vertices off.
A direct upper bound to all those diminishings can be tought of by calculating in how many ways one can cut random vertices off of the 600-cell, and one thus naively gets 2^120.

Now this upper bound is quite ugly, as it does not take the symmetries of the 600-cell into account.
One way to make a similar upper bound, while considering these symmetries is by using burnsides lemma.
This is what I've just done. I've written a program that enumerated all symmetries of the 600-cell, and then enumerate how big the orbit of every vertex is according to a given symmetry. Here are the results:

Code: Select all

1:120 1
1:30 2:90 60
1:10 5:110 288
1:4 2:116 450
1:2 2:8 10:110 1440
2:10 5:30 10:80 1440
2:6 3:30 6:84 1200
1:6 3:114 400
2:4 4:116 1800
1:2 2:4 6:114 1200
30:120 960
20:120 1440
15:120 960
12:120 1200
10:120 336
1:2 2:118 60
2:10 10:110 288
6:120 40
2:6 6:114 400
5:120 336
4:120 60
3:120 40
2:120 1

So there are 60 symmetries that return 30 vertices after 1 group-operation (this means those vertices are kept fixed), and the other 90 vertices after 2 group-operations, and 288 symmetries that keep 10 vertices fixed, and returns the others after 5 group-operations, and so on.
(It can be shown that those 60 symmetries are the simple reflections of the 600-cell in a 3-plane, and 144 of the 288 symmetries are the rotations around 72 degrees.)
Now Burnside's lemma tels us that the total number of diminishings of the 600-cell thus is

Code: Select all

1/14400*(2^(120/1)*1+2^(30/1+90/2)*60+2^(10/1+110/5)*288+2^(4/1+116/2)*450+2^(2/1+8/2+110/10)*1440+2^(10/2+30/5+80/10)*1440+2^(6/2+30/3+84/6)*1200+2^(6/1+114/3)*400+2^(4/2+116/4)*1800+2^(2/1+4/2+114/6)*1200+2^(120/30)*960+2^(120/20)*1440+2^(120/15)*960+2^(120/12)*1200+2^(120/10)*336+2^(2/1+118/2)*60+2^(10/2+110/10)*288+2^(120/6)*40+2^(6/2+114/6)*400+2^(120/5)*336+2^(120/4)*60+2^(120/3)*40+2^(120/2)*1)

Code: Select all

=92307499707443390526727850063504, approximately 2^106.186

This number includes the complete 600-cell, the empty set of vertices, and other obviously non-CRF solids.
However, it is a solid upper bound on the total diminishings of a normal 600-cell, and the method used can be extended to calculating simple sets of diminishings, such as the "Special Cuts", and the set of diminishings by which no subset of deleted vertices forms a triangle.
I think I will be going to try to recalculate the "Special Cuts" and those triangle diminishings. Other diminishings are not that simple using this method (or any other method I guess).

The CRF's you mention in the other part of the post can't be easily enumerated by these means either. However, the J92-rombochoron can be made by diminishing a EKF of the 600-cell. Because all EKF's of the 600-cell have been enumerated, we could also look at the possible diminishings of those.

[quickfur]

Haha, I've been out of the loop for so long... I don't even know what EKF stands for.

[student91]

Well, EKFs are Expanded Kaleido-Facetions. This is not that easy to explain, but let me try this:
Most CRF's do have some symmetry, eg o5o3o3x has 600-cell symmetry( ), but also demitesseractic(.3.3.*b3.), 3,3-duoprismatic(.3.2.3.), 5,5-duoprismatic(.5.2.5.), 5-cell-ic(.3.3.3.), icoshaedral-prismatic(.5.3.2.) etc.
Good thing about this is that the 600-cell can thus be represented in all those symmetries (e.g. in .3.3.*b3.-symmetry one gets foxo3ooox3xfoo*b3oxfo&#zx iirc).

On a different note: a Kaleido-faceting is a operation on the nodes of such a diagram: Any node (with say value x) can be negated (so the value becomes -x). All nodes next to this node must then be adjusted according to some not too complicated rules. (if two nodes with inital value a and b are connected with a branch labeled '4', then negation of 'a' results in values (-a) and (b+a*sqrt(2)), because sqrt(2) is the shortchord of a 4-gon).
Thus when one has e.g. ike=xofo3ofox&#xt, doing this on the first x yields (-x)ofo3xfox&#xt (the x comes from o+x*[shordchord of 3-gon]=x).
(it might be useful to see that xofo3ofox&#xt=x3o||o3f||f3o||o3x->(-x)3x||o3f||f3o||o3x, so only one element gets changed).
Interpreting such a diagram with negative nodes has proven to be quite difficult though it's certainly not impossible.

Now an EKF is an expansion of such a diagram, such that all (-x)'s become positive again. So (-x)ofo3xfox&#xt will be expanded on the first node to give oxFx3xfox&#xt. This whole process can beautifully be seen in this image:
So that's actually the processes you discovered to change ike into bilbiro/thwaro/pocuro etc.

Now the interesting things come when this is applied to 4-dimensional members of the 600-cell family. You then get very interesting, highly-symmetric polytopes with Bilbiro's, pocuro's and thawro's, that are still intimately related to the former member of the 600-cell family (There is some kind of correspondence between the elements of the KF of the member and the final EKF)

So basically it is the morphing of ike into bilbiro, but then applied to any polytope that produces a CRF.

This topic is dedicated to the enumeration of all the EKF's, most of the 600-cell family.

[quickfur]

Ah I see, so it's just a fancy name for that "partial expansion" thingy we found back then. Got it. Thanks!

[Klitzing]

Ah I see, so it's just a fancy name for that "partial expansion" thingy we found back then. Got it. Thanks!

Not quite. Partial expansion is just one of the processes subsumed here. The other one is kaleido faceting. - My older partial Stott expansions generally started with convex figures already, which get expanded according to some subsymmetry. Whereas in EKFs we first produce an (also subsymmetrical) faceting, which then gets expanded back to convexity.

Cf. here for partial Stott expansions and expanded kaleido facettings. - The latter one provides a lot more than just EKFs obtained from the 600-cell only.

--- rk

[Klitzing]

Dreamed up a nice further CRF.

Here is how I came to it:
Consider ex = 600-cell first. Orient it in its o5o2o5o subsymmetry, i.e. use the according lace city display.

Code: Select all

                  o5o           o5o                 
                        o5x                       
                                                  
            x5o                     x5o           
     o5o                                   o5o     
                        f5o                       
                 o5f           o5f                 
                                                  
     o5x                                   o5x     
            f5o                     f5o           
                                                  
o5o                     x5x                     o5o
                                                  
            o5f                     o5f           
     x5o                                   x5o     
                                                  
                 f5o           f5o                 
                        o5f                       
     o5o                                   o5o     
            o5x                     o5x           
                                                  
                        x5o                       
                 o5o           o5o                 

Then it is obvious that it allows a cyclo-penta-diminishing with 5 tip-to-tip ikes. The same holds true then for the completely orthogonal great circle of vertices (the central "x5x"). Thus the according bicyclic deca-diminishing would look like:

Code: Select all

                          o5o                 
                   o5x                       
                                              
       x5o                     x5o           
o5o                                           
                   f5o                       
            o5f           o5f                 
                                              
o5x                                   o5x     
       f5o                     f5o           
                                              
                   x5o                     o5o
                                              
       o5f                     o5f           
x5o                                   x5o     
                                              
            f5o           f5o                 
                   o5f                       
o5o                                           
       o5x                     o5x           
                                              
                   x5o                       
                          o5o                 


(So far I'm not fully clear whether that one then is an enantiomorph figure or not. At least, you could use "x5o" or "o5x" in the center when already having fixed the "outer" cyclo-diminishing.)

That one so far is not too interesting. It is closely related to "gap" (the great antiprism), the single extra uniform figure, i.e. the non-Wythoffian besides to sadi and the antiprism-prisms. That one has 2 orthogonal rings of 10 pentagonal antiprisms and lots of tetrahedra, which devide in mere adjacent ones and isolated ones. In fact that bicyclic deca-diminishing happens to be just that gap with alternating re-attached pentagonal-antiprismatic pyramids, where the introduced pentagonal pyramids happen to become corealmic to the remaining pentagonal antiprisms, so that these re-combine to full icosahedra again.

The thing here is just that you would have to start with a 4-coloring of gap first, e.g. coloring the paps (pentagonal antiprisms) of the gap alternatingly in red and yellow for the first ring and green and blue for the second. And then decide to re-augment the yellow and blue paps, so that you'll get cycles of 5 red resp. 5 green tip-to-tip icosahedra.

But then, what is much more interesting now, is the following:

When considering rahi (the rectified hecatonicosachoron) in right the same subsymmetry, i.e. as lace city, then it looks like this:

Code: Select all

                                                                x5o                                                               
                                                        o5f             o5f                                                       
                                                                                                                                  
                                            x5x                                     x5x                                           
                                                    o5F                     o5F                                                   
                                f5o                                                             f5o                               
                        o5x             F5o                     o5V                     F5o             o5x                       
                                                                                                                                  
                                                    x5F                     x5F                                                   
                    f5o                     F5x                                     F5x                     f5o                   
                                                                                                                                  
                                V5o                     F5f             F5f                     V5o                               
                                                                B5o                                                               
                    F5o                     f5F                                     f5F                     F5o                   
            x5x                                                                                                     x5x           
                                                                                                                                  
                        F5x             o5B                     V5f                     o5B             F5x                       
                                f5F                                                             f5F                               
            o5F                                                                                                     o5F           
    o5f             x5F                     f5V                                     f5V                     x5F             o5f   
                                                                                                                                  
                                                        F5F             F5F                                                       
x5o                     F5f                                     B5x                                     F5f                     x5o
                                                                                                                                  
            o5V                                     x5B                      x5B                                    o5V           
                                            F5F                                     F5F                                           
o5f                     B5o                                     o5C                                     B5o                     o5f
                                V5f                     C5o             C5o                     V5f                               
                                                                                                                                  
    o5F             F5f                     B5x                                     B5x                     F5f             o5F   
            x5F                                     o5C                     o5C                                     x5F           
                                                                                                                                  
x5x                                     F5F                                             F5F                                     x5x
                                                                                                                                  
            F5x                                     C5o                     C5o                                     F5x           
    F5o             f5F                     x5B                                     x5B                     f5F             F5o   
                                                                                                                                  
                                f5V                     o5C             o5C                     f5V                               
f5o                     o5B                                     C5o                                     o5B                     f5o
                                            F5F                                     F5F                                           
            V5o                                     B5x                      B5x                                    V5o           
                                                                                                                                  
o5x                     f5F                                     x5B                                     f5F                     o5x
                                                        F5F             F5F                                                       
                                                                                                                                  
    f5o             F5x                     V5f                                     V5f                     F5x             f5o   
            F5o                                                                                                     F5o           
                                F5f                                                             F5f                               
                        x5F             B5o                     f5V                     B5o             x5F                       
                                                                                                                                  
            x5x                                                                                                     x5x           
                    o5F                     F5f                                     F5f                     o5F                   
                                                                o5B                                                               
                                o5V                     f5F             f5F                     o5V                               
                                                                                                                                  
                    o5f                     x5F                                     x5F                     o5f                   
                                                    F5x                     F5x                                                   
                                                                                                                                  
                        x5o             o5F                     V5o                     o5F             x5o                       
                                o5f                                                             o5f                               
                                                    F5o                     F5o                                                   
                                            x5x                                     x5x                                           
                                                                                                                                  
                                                        f5o             f5o                                                       
                                                                o5x                                                               


which displays quite nicely its pentagon-to-pentagon attached icosidodecahedra, e.g. as its outer decagonal ring: x5o || o5f || x5x || f5o || o5x.

Now I tried to transfer the above idea to that fellow. Here we don't have the possibility for monostratic diminishings when considering CRF outcomes, but we have the possibility for bistratic ones. In fact we are allowed to reduce full ooo3xox5ofx&#xt caps.

That cap, i.e. ooo3xox5ofx&#xt = id || f-doe || tid, itself is already well known. E.g. pictured by quickfur quite nicely in a post from 2011.

So, now consider we apply an according bistratic cyclo-penta-diminishing onto rahi. That works quite nicely. It clearly eliminates 5 outer icosidodecahedra and replaces those with 5 new truncated dodecahedra, which then connect pairwise at their (opposing) decagons. Further it eliminates 5 times the contained 2*20 tetrahedra. And further each such cap cuts 12 of the neighbouring icosidodecahedra into halves, i.e. into peroes (pentagonal rotundae). But consider these decagonal attachments. Therefore there these icosidodecahedra become bi-diminished from either side, and thus become reduced to just their (subdimensional) equatorial decagon. Thus the net effect of a mere bistratic cyclo-penta-diminishing of rahi reduces the former 120 ids (icosidodecahedra) and 600 tets (tetrahedra) to 120 - 5 - 5 - 5*10 = 60 ids, 600 - 5*2*20 = 400 tets, 5 tids (truncated dodecahedra), 5*10 = 50 peroes.

Its lace city then would be:

Code: Select all

                                                                        x5x                                           
                                                                o5F                                                   
                                                                                                                      
                                                    o5V                     F5o                                       
                                                                                                                      
                                        x5F                     x5F                                                   
                                F5x                                     F5x                                           
                                                                                                                      
                    V5o                     F5f             F5f                     V5o                               
                                                    B5o                                                               
        F5o                     f5F                                     f5F                                           
x5x                                                                                                                   
                                                                                                                      
            F5x             o5B                     V5f                     o5B             F5x                       
                    f5F                                                             f5F                               
o5F                                                                                                                   
        x5F                     f5V                                     f5V                     x5F                   
                                                                                                                      
                                            F5F             F5F                                                       
            F5f                                     B5x                                     F5f                       
                                                                                                                      
o5V                                     x5B                      x5B                                    o5V           
                                F5F                                     F5F                                           
            B5o                                     o5C                                     B5o                       
                    V5f                     C5o             C5o                     V5f                               
                                                                                                                      
        F5f                     B5x                                     B5x                     F5f             o5F   
x5F                                     o5C                     o5C                                     x5F           
                                                                                                                      
                            F5F                                             F5F                                     x5x
                                                                                                                      
F5x                                     C5o                     C5o                                     F5x           
        f5F                     x5B                                     x5B                     f5F             F5o   
                                                                                                                      
                    f5V                     o5C             o5C                     f5V                               
            o5B                                     C5o                                     o5B                       
                                F5F                                     F5F                                           
V5o                                     B5x                      B5x                                    V5o           
                                                                                                                      
            f5F                                     x5B                                     f5F                       
                                            F5F             F5F                                                       
                                                                                                                      
        F5x                     V5f                                     V5f                     F5x                   
F5o                                                                                                                   
                    F5f                                                             F5f                               
            x5F             B5o                     f5V                     B5o             x5F                       
                                                                                                                      
x5x                                                                                                                   
        o5F                     F5f                                     F5f                                           
                                                    o5B                                                               
                    o5V                     f5F             f5F                     o5V                               
                                                                                                                      
                                x5F                                     x5F                                           
                                        F5x                     F5x                                                   
                                                                                                                      
                                                    V5o                     o5F                                       
                                                                                                                      
                                                                F5o                                                   
                                                                        x5x                                           


But clearly we can do that also on both orthogonal rings. Then we get the bistratic bicyclic deca-diminishing of rahi with the following total count of facets: 120 - 2*5 - 2*5 - 2*5*10 = 0 ids, 600 - 2*5*2*20 = 200 tets, 2*5 = 10 tids, 2*5*10 = 100 peroes. I.e. the former Icosidodecahedra are fully gone, and we remain just with the introduced 10 truncated dodecahedra, 100 Johnsonians (the pentagonal rotundae), plus some remaining tetrahedra (200 of them). That's all. - I think that's a nice and quite interesting find, ain't it?

Would like to have some pics of it ...
(Suppose quickfur would like to have some try, ain't he?)

--- rk

[Klitzing]

Hehe,
we might even continue that above idea: What about expansions thereof?

Well, the icosidodecahedron itself cannot be expanded, else we won't have CRF cuts any longer.
But then, what about expanding rahi o3o3x5o into srix x3o3x5o? That should work.

So, what are the cells of srix?
These are 120 ids (icosidodecahedra), 600 coes (cuboctahedra), 720 pips (pentagonal prisms).

What will be here the corresponding to be cut off caps?
Here we would use a tristratic cap: . o3x5o (id) || . x3x5o (ti) || . x3o5f (xf-srid) || . x3x5x (grid) = oxxx3xxox5oofx&#xt.

What then would be the corresponding final figure?
Here we'd thus consider the bicyclically tristratic deca-diminished srix.
That one then has for total cell count: 120-10-10-10*10=0 ids, 0+10*10=100 peroes, 0+10=10 grids, 600-10*20-10*20=200 coes, 0+10*20=200 tricues, 720-10*42=300 pips.

That is, while the former (last mail) was a 310 cell CRF polychoron, that one now is a 810 cell CRF polychoron.

--- rk

[Klitzing]

Still am overwhelmed myself by the coolness of these figures.
But, on the other hand, as I now stumbled upon myself, these are by no means new.

• Rather I myself already had invented the latter one, the bicyclically tristratic deca-diminishing of srix, quite exactly 3 years ago in 2013, cf. this post of mine.
• And in the sequel quickfur and I also then came up with the other one too, the bicyclically bistratic deca-diminishing of rahi.
  From that one he then even already provided several pics, cf. this post and this post.
• What in those days has not being investigated, but within the above now also is contained, is the bicyclically monostratic deca-diminishing of ex.

--- rk

[quickfur]

Yes, I remember we discovered the bicyclo-diminished rahi.

An interesting thought that occurs to me is the inverse operation: what if we take a 120-cell family uniform polychoron with many marked nodes in the CD diagram, and derive a bicyclo-deca-contraction of it? E.g., if we start with x5x3x3x, identify two orthogonal rings of five x5x3x's, and contract them to, say, x5o5x (as an example, I don't know if this will be CRF)? Which combinations would produce a CRF? If a CRF is produced, perhaps it could be equivalent to some augmentation of some underlying polychoron with one or more CD nodes unmarked, like x5o3x3x?

[Klitzing]

Still am wrangling around with these - esp. on the level of classification of elements. Cause the swirl symmetry does not bow to that easily. But for the setup of their incidence matrices it is needed to distinguish between alike elements whenever they are to be used in different orbits of symmetry...

The 2 rings of tids are quite clear. That these are in mutual orthogonal orientation, too. That the peroes are attached to the decagons also is obvious. But then it runs very fast out of easy visualization. - Quickfur, do you have some clues on how these peroes of the two rings are to be connected? Which classes of tetrahedra are to be distinguished? How look the surroundings of the tid-to-tid connections, i.e. the ones of the sides of these decagons? And how do look the souroundings of the equatorial zick-zacks of each tid? How do these more or less parallel stripes of one ring correlate to the orthogonal ones of the other ring?

Moreover: I think, we so far have not even settled down on the question whether these 2 figures are chiral or not...

--- rk

[quickfur]

Still am wrangling around with these - esp. on the level of classification of elements. Cause the swirl symmetry does not bow to that easily. But for the setup of their incidence matrices it is needed to distinguish between alike elements whenever they are to be used in different orbits of symmetry...

The 2 rings of tids are quite clear. That these are in mutual orthogonal orientation, too. That the peroes are attached to the decagons also is obvious. But then it runs very fast out of easy visualization. - Quickfur, do you have some clues on how these peroes of the two rings are to be connected? Which classes of tetrahedra are to be distinguished? How look the surroundings of the tid-to-tid connections, i.e. the ones of the sides of these decagons? And how do look the souroundings of the equatorial zick-zacks of each tid? How do these more or less parallel stripes of one ring correlate to the orthogonal ones of the other ring?

Moreover: I think, we so far have not even settled down on the question whether these 2 figures are chiral or not...

--- rk

Have been meaning to get around to this. Does this post help? I think that tetrahedral chiral configuration of 4 peroes around a tetrahedron, in the first image in that post, basically lies on the interface between the two orthogonal rings. So 2 of the peroes connect to one ring (two adjacent tids) and the other 2 peroes connect to the other ring (also two adjacent tids). The connection between the two rings is perpendicular, basically treating the tetrahedron as a line antiprism.

In the second image you can see vague outlines of these tetrahedra around the equatorial belt of the projection image (they are incomplete because for clarity I omitted the edges that don't belong to a peroe or tid in the vertical ring, but the polygons are still there and you should be able to see them if you look carefully). In the front of the image, the 3 peroes (1 magenta + 2 blue) belonging to the vertical ring trace out an outline of another peroe that attaches to the orthogonal ring. There's another peroe to the right, outlined by 2 magenta peros and 1 blue, slightly higher than the previous one, on the other side of the outlined tetrahedron. So there's 1 magenta, 1 blue, and 2 peroes attached to the other ring around this tetrahedron.

Can you see it?

[quickfur]

As for whether it's chiral or not... I don't know, how would we tell? The configuration of peroes around a tet is certainly chiral, but it doesn't mean the whole polytope is chiral, since there could be another subset of peroes around tets in the opposite orientation, such that the two sets interchange upon a mirror reflection, so the overall polytope remains unchanged.

Perhaps if we determine whether all occurrences of 4 peroes around a tet are of one orientation only? Then upon a mirror reflection the resulting polytope would have all occurrences in the opposite orientation, so it cannot be equivalent to the original polytope and therefore it must be chiral. But is this necessarily so? Is there some other rigid motion that could reverse the sense of surface elements such that the entire polytope is not chiral even when some surface elements are chiral and only in one of the two possible orientations?

[Klitzing]

In fact, I knew about that mail of yours. But it didn't help so far. Esp. it only takes account to the pinched in tets close to the tid-tid decagons. But what then is the local configuration at the equators of those tids?

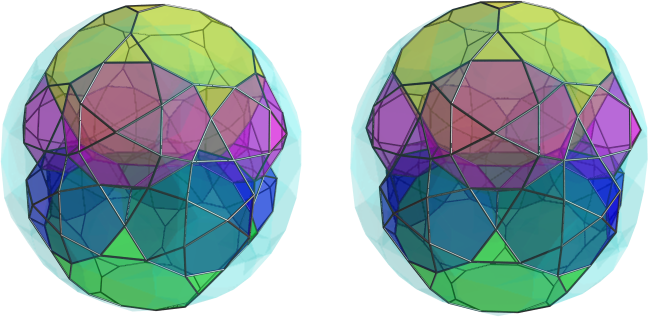You are right, your picture indeed not only displays these mentioned pinched in tets, it further shows up kind a non-flat larger square around, wobbling at its equatorial plane. Each larger square side then is outlined by 2 pentagonal sides and one trigonal one inbetween. One such convex sequence can easily be spotted running on the magenta pero, the opposite one, also convex, on the blue pero, and the other 2 concave sequences run from magento to blue pero. Together with your assertion that all(?) these work in the symmetry of a digonal antiprism, this kind of now provides a clue on how the next peroes will have to fit in. (Else the mere concave patches do not settle that!)

But then, this cannot be the full story either. Right between these magenta and blue peroes there can such pinched in tets be spotted which tilt to the left and ones to the right, alternatingly. But from your mentioned digonal antiprism symmetry no decagon has to connect directly to its edges (as this obviously holds at least for the magenta and blue peroes). But when using this as a rule, say, to the most frontal one, then the uppermost pentagon in axial position of the then to be attached pero (of the opposite ring) ought be the blue pentagon below that tet resp. the magenta pentagon directly above. And then therefrom it would follow that the bottom decagons of these just attached 2 peroes would connect to the outer edges of the 2 neighbouring pinched in tets. So it follows that these 2 types of tiltings will NOT be equivalent after all. Ones may be surrounded with digonal antiprismatic symmetry, esp. with pentagons emanating diametrally from their degenerate bases (by application of your claim), but the other one uses such a pentagon only at one of its bases, while on the other base will get 2 incident decagons. Thus it looks like having there an incident tid, being attached by the remaining part of a dodecahedral edge of the equatorial zick-zack...

I fear, I still need much more light in that fog.

--- rk

[quickfur]

Hmm you are right. This isn't the whole story. I'll have to dig up the model file for this CRF and see if I can figure this out.

This will have to wait a bit, 'cos right now I'm working on elaborating the basis vectors for the CD diagrams in n-simplex symmetry, and I'm discovering some very interesting patterns in the composition of these bases. Using the "native" orientation of the n-simplex that I discovered some years ago, the coordinates thus derived exhibit very nice patterns that recurse as the number of dimensions increase, dual orientation is obtained by negating all coordinates, and it exhibits an interesting connection to the inverse triangular numbers. This I'm doing, because I have found that the CD diagram (Dynkin symbol) can be interpreted as a Minkowski sum of basis vector convex hulls. I'm computing the basis vectors for .3.3.3. symmetry esp. because I have been asked to build and render models for student91's xFfxo3xxxof3foxxx3oxfFx&#zx polychoron, which promises to be a most fascinating BT polychoron with (AFAICT) 5-cell symmetry.

In short, given some symbol aPbQcRd..., and sets S_i of basis vectors corresponding to xPoQoRo..., oPxQoRo..., oPoQxRo..., etc., we can compute the entire polytope as:

convexHull(aPbQcRd...) = convexHull(a*(S₁) + b*(S₂) + c*(S₃) + ...)

where + denotes Minkowski addition. This works not only for a, b, c, = x, o, but for any scalar value marked on the corresponding node. This allows automatic conversion of a CD diagram to its polytope model as a set of vertices. Of course, this depends on knowing what the basis sets S_i are. For the simplex family, the general formula for the regular simplex has been known a while ago, but now I'm seeing patterns in deriving the basis sets of the uniforms o3x3o3o..., o3o3x3o.. etc., which are required to complete the basis sets. They are also recursively defined, and can ultimately be derived from the lowest dimensional basis sets.

[Klitzing]

Not too difficult for the simplices, i.e. when P=Q=R=...=3.

Just consider the (N+1)-dimensional hypercube, its vertex to vertex diagonal, and hyperplanes orthogonal to that one. Then the respective sections at vertex layers would be nothing but the q-scaled quasiregular simplices, i.e. something of type o3o...o3q3o...o3o. You even get the regression, when numbering the layers from 0 to N+1: The k-th layer would require the k-th node being marked q. The zeroth and (N+1)st layers would require the marked node being outside, and indeed, the empty diagram clearly indicates these extremal vertices correctly. Thus these cases a posteriori can be subsumed here too.

--- rk

[Klitzing]

Nearly one whole year of silence within this thread.

I don't have to provide a new CRF today. Rather I want to show up a connection between 3 already known ones.

What do you think is the mental link between

• the segmentochoron 3||teddi,
• the tetrahedral ursachoron, and
• the 3-mibdies-laced-wedge ?

Have a try - before I'll post the answer ...

--- rk