Traversable Klein 'bottle' paths

[gonegahgah]

Hi Teragon,
Sorry for taking awhile. I've been trying to reconcile the following:

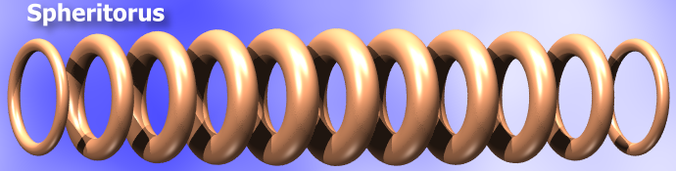
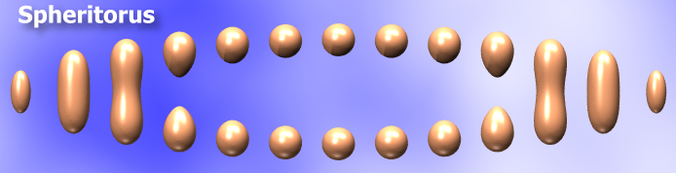
I realised that when we rotate into the 4th dimension that the Klein Strip would also appear to rotate in the opposite way with an in-place twist; and not just appear to inflate and deflate.
However when I add that to the equation I appear to get the above...

I'm still trying to reconcile that with my brain...
It appears that their is some sort of figure 8 motion occuring which may be why it seems to bounce and swap colours at the 90° and 270° mark...
Or it may be that opposite side is bleeding through because the graphing program doesn't know there is an opposite side and presents it as the current side...
I'm yet to figure it out...

I know we can get a similar effect with a piece of cardboard that is coloured one colour on one side and another colour on the opposite side.
If you hold this in front of your face and rotate it towards yourself you will see the first colour shrink to a line and then the second colour will come around and will start to grow then shrink (ie bounce).
It does this sudden colour change for the whole entire edge all at once which may be the equivalent to us seeing the whole surface (4D edge) change at once in our 3D slice of a Klein Strip.

It's interesting that if we did this to a 2Der with the cardboard instead standing vertical and spin it around a vertical axis the 2Der would see the shape flash from blue to red every 180° which is what we are seeing too.
So to my surprise the effect might be correct. Please remember though that I am depicting two halves of the one side. We still don't see the back unless we walk around to the back of the Klein strip.

So the animation may be correct? I must admit I just expected the revolution to continue in one direction for the full 360°. What do others think?
Still I haven't answered your questions yet. I was trying to understand the above. Hopefully soon...

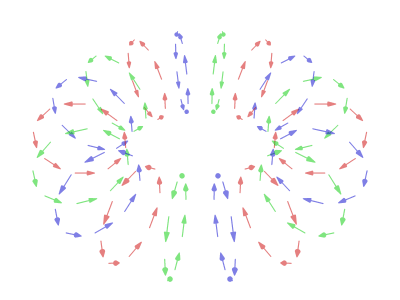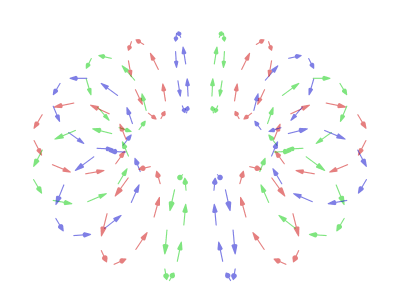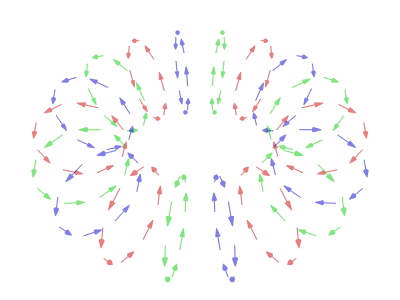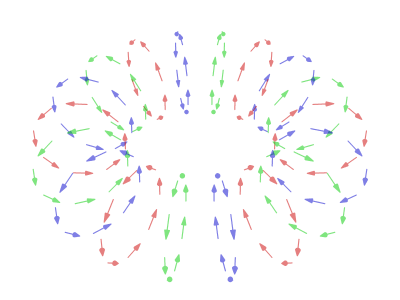
Oh and the current parametric equations for in-place rotation are now:
x(u,v,t) = (R + r * (sin(v / 2) * sin(u + t) * cos(t) * cos(v / 2 + π/2) + cos(u + t) * cos(v / 2))) * sin(v)
y(u,v,t) = (R + r * (sin(v / 2) * sin(u + t) * cos(t) * cos(v / 2 + π/2) + cos(u + t) * cos(v / 2))) * cos(v)
z(u,v,t) = r * (sin(v / 2) * sin(u + t) * cos(t) * sin(v / 2 + π/2) + cos(u + t) * sin(v / 2))
I've used two sets of values for the other variables to produce the two colours:
1) -π/2 ≤ u ≤ π/2 (15 steps)
2) π/2 ≤ u ≤ 3π/2 (15 steps)
both) 0 ≤ v ≤ 2π (90 steps)
both) 0 ≤ t ≤ 2π (120 steps)

[Secret]

Take your time.

Agree with your definitions. So a "strip" in 4D is a 3D object?

Yes, it is. The more technical term for the object gonegahgah is describing is solid klein bottle

The "thickness the Klein surface needs" was maby an inapproprate wording. It's clear that surfaces need a certain thickness in order to sustain even their own weight. What I actually meant is, in 4D you'd have to balance on a (quasi-)2D surface like on a tightrope in 3D. And in 4D, orientability of 2D surfaces is somewhat different than in 3D, because a normal vector may not be defined (only a normal plane). Imagine balancing around a loop on a 2D Klein surface in 4D. You may always stay on top of it. Reaching the same place again, how do you know you've switched side? The only thing that will have happend is that left and right (the directions in which you balance out) have switched.

Consider the following:

backup 1.PNG (63.66 KiB) Viewed 28246 times

Since the klein strip is a 3D nonorientable manifold, we can locally define a vector that is normal to this 3D "surface" (chorix as defined in Polygloss, hypersuface in 4D) at all points. Now if you imagine translating this vector along the klein strip following the black arrow, you will reach the "pinched region" which you will then flip (as looking from our 3D vantage point, that part of the surface dived into the other part, thus seems as if it is moving inside. However inside and outside to a 4Der is not bound by the 2D surface/manifold, rather it is bound by a 3D manifold. To a 4Der, the 3D manifold is basically "thin" in the 4th direction, thus they define the "front" and "rear" of this 3D surface based on the normal vector pointing towards or away in 4D from the surface, analogous to how we define up and down on a 2D plane) (NB the dark blue thing is the analogous situation for 3Ders walking on a moebius strip (assuming they don't fell over)

In order to walk comfortably you need also a certain extension in the second lateral direction. Now the object hase a normal vector pointing always in the vertical direction, the surface has now an upper side and a clearly distinct bottom side. So it's a path on which left and right flip sides within one circle without you turning headfirst.

So the klein strip naturally occupied a xyz region, analogous to how the middle of the moebius strip has a 2D surface for a 3Der to set foot on

If the 2D Klein surface in the middle of this 3D promenade was extended vertically to a wall, you'd be able to cross the wall just by walking along (in this case a bridge is needed). Such a 3D wall has an open, non-orientable surface, just like a Moebius strip in 3D.

Yes, that "tubing" you form by extending the klein bottle (2D bounding surface of the klein strip) will be a nonorientable 3D manifold. However, since the klein bottle is a 2D manifold in 4D space, attempt to build a wall like so will result in self intersection at the twist region, unless the wall twist with it as shown, which if we assume gravity to be pointing katwards, then theoretically our 4Der can hop into the middle or hop out of the klein strip to enter or leave it

EDIT: About the wall thing, no the 4Der cannot leave the loop unless he/she jumped, because buidling such a wall with necessary make it extend both anwards and katwards, because it flips when it loop through the klein strip's twisted region, thus enclosing the klein strip completely (enclosing as in analogous to a cylindrical wall in 3D enclosing access to the region in the middle). however such structure must have a small region of self intersection in 4D space, because the klein bottle is already 4 dimensional thus all avaiable directions in 4D that allow extrusion without any obstruction is being used up
To check whether you will collide into something in 4D, remember that if we assume gravity is in the 4th direction, then a 4Der has only 3 degrees of freedom to walk around

Start at the centre of any of the circles in the klein strip, you can walk towards the edge of any of these circles. Now if I eract a wall surrounding the circles, then if you walk towards the boundary of the circles by walking in x or y direction, you will eventually bump into the wall. The z direction however is unobstracted, thus you can walk along that to get to antoher section of the klein strip. If we build the walls around the klein strip so that it is always ereacted anawards and katward (ignoreing the self intersection that will take placei n 4D for the moment, then our 4Der cannot leave the klein stripe because all possible ways will send the 4Der head on into the klein bottle shaped wall

backup 3.PNG (63.08 KiB) Viewed 28246 times

In another sense it's derived from the 2d Klein surface (closed in two directions). Is this the "Klein strip" you're talking about? This object might be identical with the Moebius strip I described in the last paragraph of my post above. In one direction on the strip you get to the other side with one loop, in another direction you just do a loop without anything happening (analog to the Klein surface in 3D) and yet another direction makes up the width of the strip (analog to the Moebius strip in 3D).

Hope that was somewhat comprehensible.[/quote]

backup 2.PNG (41.59 KiB) Viewed 28246 times

Yes, indeed, the klein bottle (2D bounding manifold of the klein strip) has two different kinds of loops. Imagine you are a 2Der embedded on the klein bottle, then if you walk along the boundary of those grey circles, you end up back where you started without flipping. You will be flipped sideways ,however if you walked all the way around passing that twisting region

A 4Der is similar. If they walked in small circles on the klein strip, they just end up where they started (they cannot walked all the way to the edge (defined by the grey envelope in the projection, which is the klein bottle bounding 2D surface of the klein strip) otherwise they will fall off). If they walked all the way around, then they will be flipped upside down. If gravity does not flip with them, then they will fall off as they pass halfway through the twisted region

The klein strip is derived from a klein bottle in that you fill in the middle, empty region of the klein bottle, similar to the 2D paper middle of a moebius strip. So it is basically a moebius cylinder

P.S. I currently don't have enough time to model them in terms of maths render, sorry for that

Hi Teragon,
Sorry for taking awhile. I've been trying to reconcile the following:

I realised that when we rotate into the 4th dimension that the Klein Strip would also appear to rotate in the opposite way with an in-place twist; and not just appear to inflate and deflate.
However when I add that to the equation I appear to get the above...

backup 4.PNG (187.32 KiB) Viewed 28246 times

Can you try rotate this about the xz plane as shown, I am suspecting the green line region will expand into a circle and the olive region to contract into a line in the projection/3D rotation slide?

[gonegahgah]

Hi Secret, thanks for also talking with Teragon too. I appreciate that.

Your thoughts on what it would look like tipped on its side were what I was starting to think too.
As it is lifted into the vertical I imagine the pinch traveling around the side until, as you say, when fully upright the pinch is now at the top; in our 3D slice.
Tipping the Klein strip further would then see the pinch traveling down the other side until the Klein strip is now on its flip side with the pinch back where it was; and us seeing the before hidden underside instead of the top.
It conversely also becomes the left-handed - to us - version of a Klein Strip in the process (I think; does it?).
Conversely the the full cylinder diametre cross-section would also travel down and to the bottom via the opposite side, to when fully standing, and back up the other side to the old back when we tip it fully over onto its flip side.

I've done a few more animations to help explain the interesting colour flipping which I'll add tomorrow when I'm using my off-peak bandwidth.
It seems that its a bit of both of the reasons I posited...
I have a feeling I should be able to depict what ICN5D was asking for ie. the different side-steps of the Klein strip tipped up at different angles. We'll see...
I think I may be able to define some 4D parametric equations too hopefully; which would be fun if it can be done.

Patterns are always fascinating. For example we have x,y,z for our 3D co-ordinates. 4D space adds w as a fourth spatial co-ordinate.
The program I've used for these models utilises u,v for its parametric equations - which seems to be the norm.
It also uses t for time as also is the norm... So we've used up t,u,v,w,x,y,z. Nice of our alphabet to be arranged that way.

I think I should be able to steal the t to model 4D objects.
I've been doing that here of course but I may have to extend that into the 4th dimension as well rather than just using it to limit us to our 3D frame. We'll see...
Though eventually you want to have t back in its proper place as time and borrow some other letter (q?) r is radius, s is speed...

[Teragon]

Hi Secret,

The "thickness the Klein surface needs" was maby an inapproprate wording. It's clear that surfaces need a certain thickness in order to sustain even their own weight. What I actually meant is, in 4D you'd have to balance on a (quasi-)2D surface like on a tightrope in 3D. And in 4D, orientability of 2D surfaces is somewhat different than in 3D, because a normal vector may not be defined (only a normal plane). Imagine balancing around a loop on a 2D Klein surface in 4D. You may always stay on top of it. Reaching the same place again, how do you know you've switched side? The only thing that will have happend is that left and right (the directions in which you balance out) have switched.

Consider the following:

backup 1.PNG

Since the klein strip is a 3D nonorientable manifold, we can locally define a vector that is normal to this 3D "surface" (chorix as defined in Polygloss, hypersuface in 4D) at all points. Now if you imagine translating this vector along the klein strip following the black arrow, you will reach the "pinched region" which you will then flip (as looking from our 3D vantage point, that part of the surface dived into the other part, thus seems as if it is moving inside. However inside and outside to a 4Der is not bound by the 2D surface/manifold, rather it is bound by a 3D manifold. To a 4Der, the 3D manifold is basically "thin" in the 4th direction, thus they define the "front" and "rear" of this 3D surface based on the normal vector pointing towards or away in 4D from the surface, analogous to how we define up and down on a 2D plane) (NB the dark blue thing is the analogous situation for 3Ders walking on a moebius strip (assuming they don't fell over)

Yes, but you're one step ahead. My paragraph describs the 2D-manifold in 4D.

EDIT: About the wall thing, no the 4Der cannot leave the loop unless he/she jumped, because buidling such a wall with necessary make it extend both anwards and katwards, because it flips when it loop through the klein strip's twisted region, thus enclosing the klein strip completely (enclosing as in analogous to a cylindrical wall in 3D enclosing access to the region in the middle). however such structure must have a small region of self intersection in 4D space, because the klein bottle is already 4 dimensional thus all avaiable directions in 4D that allow extrusion without any obstruction is being used up
To check whether you will collide into something in 4D, remember that if we assume gravity is in the 4th direction, then a 4Der has only 3 degrees of freedom to walk around

It doesn't have to intersect itself if you raise it at the critical location, i.e. build a bridge.

The klein strip is derived from a klein bottle in that you fill in the middle, empty region of the klein bottle, similar to the 2D paper middle of a moebius strip. So it is basically a moebius cylinder

Don't understand what you mean. I thought it had just gotten a certain width, like the width of a Moebius strip. The Klein bottle has no inside and therefore no middle.

[Teragon]

Hey gonegahgah,

I'm just starting to follow your animations, so I can't tell if something's wrong.
Still not sure if we're talking about exactly the same object, because for a 3D surface closed in two directions, open in one, I see no reason why it should intersect itself. When I watch the moving cross-section, the strip seems to be closed in 3 directions instead of two.

[gonegahgah]

Here is the animation to make it clearer why my two colour depiction 'flips' from one colour to the other colour:

The moving blue snake shown is a render of part of the surface as it is in our current 3D slice of the 4D space as we rotate our 3D slice a full 360° around in 4D.

If you watch it closely you can see that while travelling down and while travelling up the blue snake appears to flip from the front to the back.
If you watch the wire frame circles that are spinning in place all along the Strip you will notice that this flip occurs when the circles appear to us to become straight lines.

This is the clue because it is the point in our 360° of rotation of our 3D slice around in the 4D space that the circle representing the actual surface at each cross section flips from one of it's 'faces' to the opposite face.
The effect is that one half of the circle that was at the front is now at the back and the other half that was at the back is now at the front.
This puts the half part of the cross section of the path that we are rendering from front to back in our eyes and the eyes of our renderer software and we see the characteristic 'flip' or instant colour switch.

So in part it is the renderers and our fault because it and we don't immediately see that what we see as the front is not the front in 4D but is just one aspect of 360° of cross-sectional front.
So just as for us where we can have a whole front line of a flat-on-the-ground object at the front; a 4Der can have a whole circle of a line of their flat-on-the-ground at the front.

If you watch the path of the blue snake closely you can also see that it travels in a smooth motion describing a nice figure 8 path in its travel. At no time does it reverse direction.

So there is no 'bounce' even though it appears in the following render to do so:

Instead the part of the cross section being rendered reaches its cycle where it flips to the back and is then rendered behind. The 4Der would not see this effect anywhere like what we do.
You can see the effect more clearly in the following:

Half way through the cycle the blue rectangle flips from being in front to being behind in a sudden flash and back again at the opposite cycle. The effect we are getting is the same.

In reality all the edges of the rotating circles we are seeing from the front aspect are at the front and the back aspect is at the back.
The green filling I have used in the last animation is for our convenience only and does not actually form part of the surface; only the circle edges do.
It's unusual to think of a line as having a front and a back and especially so when it forms part of a shape.
But that is how we need to think when thinking in 4D...
The spinning circles are not as we view them but are the sum of line edge rotations and that sum can be viewed from front, back, side or any direction in its entirety by a 4Der when standing at those corresponding points.
They can't see the front aspect when they are standing at the back; they can only see the back aspect of the entire circle edges when standing at the back. And vice-versa of course...

Actually I'm not sure what the green stuff is presently. It is not the inside aka Klein Bottle. I will have to ponder this...

[gonegahgah]

Hey gonegahgah,
I'm just starting to follow your animations, so I can't tell if something's wrong.
Still not sure if we're talking about exactly the same object, because for a 3D surface closed in two directions, open in one, I see no reason why it should intersect itself. When I watch the moving cross-section, the strip seems to be closed in 3 directions instead of two.

That's cool Teragon. It can be a bit of a mental flip itself to think in 4D instead of 3D and even then those flips can have many levels of developing understanding...
I find I'm learning new things about 4D space all the time.

I think, as you are starting to get some feel of, one of the tricks is that a Klein Bottle is basically a 3D object whereas a Klein Strip is a 4D object...
All those renders I have made look like shells with hollow insides.
However what we see as insides of the Klein object in the renders are not insides at all. As I say I'm not 100% just what that 'space' is just yet and will have to ponder it...

Though it is starting to come to me...

The Klein Strip, just like a Mobius strip, has no insides as you are thinking of them. It is not a tunnel.
The entire Klein Strip path is exposed to the air; just as is the case for a Mobius strip.

Each circular cross section is just narrow view of the path from whatever direction/aspect you are looking at it. Looking at it from the front the entire circular cross-section is at the front (to a 4Der).
Unfortunately we can't view a circle as being entirely at the front except when it is, what we think, face on to us but no matter how you rotate those circle in my renders they are entirely the front representation for their full circumference.

So to understand a Klein Strip you need to move away from thinking of the circular cross-sections as walls of a tunnel. They are just part of the Klein surface and there is no tunnel.

Which gets us back to what is that green stuff...
Actually, as I mentioned, the green stuff is merely there for our convenience. It is not there for the 4Der.
So what is that space then? Well, that space is is just more outside; believe it or not!

To understand this we have to realise that this outside is not bound by the render we are depicting; due to our 3D limitations.
If we look at the Klein strip from above then obviously there is atmosphere above the Klein Strip. There's atmosphere above everything on this Earth so that's natural enough.
But, again aspect, the atmosphere is above the top of the circle cross-section from its vertical aspect and... it is also above the bottom of the circle from its vertical aspect.
There is a full extra 360° of atmosphere (360° x our 360°x360° of atmosphere) which sits above the Klein strip.

Unfortunately because of the way we have to depict things it looks like the Klein Strip is a tunnel but that couldn't be further from correct.
All the surface of the Klein strip is exposed to the atmosphere and has its own render of the atmosphere above it.
It just appears that the top of the circle cuts off the lower edges atmosphere but that's our burden; not the 4Ders... (I'll draw an illustration soon to show more clearly what I mean).

[gonegahgah]

Can you try rotate this about the xz plane as shown, I am suspecting the green line region will expand into a circle and the olive region to contract into a line in the projection/3D rotation slide?

Hi Secret, it occurred to me this morning that the pinch moving around one side and the bulge progressing around the other; as we tilt the Klein Strip means...
...that as we tilt the Klein strip it will keep exactly the same shape but just look like it is revolving in place around its donut centre. Like an orbiting ring system with a crimp.
That's one of the nice things of working on a regular shape which twists through all it's aspects by one travel of its length.

I think I should be able to write the parametric equations for that; probably with something along the lines that ICN5D suggested:

You could also combine both the translation and rotation together, for the animation. The key is to fully translate (side-step) from one side to another in 4D, at certain increments of a rotation. I usually make five scans, at 0, 22.5, 45, 67.5 and 90 degrees. See here for what that looks like with toratopes.
The function that does this is:
(x*sin(t)+a*cos(t))
(x*cos(t)-a*sin(t))
where 'a' is translate, and 't' is rotate. Not sure how to fit that in a parametric function, since I work with implicit forms.

Though in this case we would be rotating the object and not our view...

[Teragon]

I think, as you are starting to get some feel of, one of the tricks is that a Klein Bottle is basically a 3D object whereas a Klein Strip is a 4D object...
All those renders I have made look like shells with hollow insides.
However what we see as insides of the Klein object in the renders are not insides at all. As I say I'm not 100% just what that 'space' is just yet and will have to ponder it...

Though it is starting to come to me...

The Klein Strip, just like a Mobius strip, has no insides as you are thinking of them. It is not a tunnel.
The entire Klein Strip path is exposed to the air; just as is the case for a Mobius strip.

As I understand it there is an inside of the Klein strip, in the same way as there is an inside of a Klein bottle, because of the intersection (even though the 3D surface has only one side). To get inside from the outside you would have to remove part of the surface, just like in the case of the Klein bottle. If there was a hole to the outside, you could see it in several cross sections (3D hole), or the surface would not intersect itself (which is impossible for a closed 3D surface in 4D... the Moebius strip in 3D is an open surface). What you see as inside in the animation are slices of 4D-air.

What I don't understand yet is, and here may be some crucial point, the rotation shows an object that intersects itself in a Moeubius strip, while in the sliced bread animation, the intersection obviously traces out the area of a circle. In the first case it seems like in two directions the surface flips sides, whereas in the second case it seems like only one direction. Where is the middle of the object along the dirction the cuts and taken, where is the start?




In any case it's a nice object. Quite different from the Moebius strips I've described. When I've got all the clues together I'm going to give a systematic summary of the different onesided surfaces in 3D and 4D.

[gonegahgah]

As I understand it there is an inside of the Klein strip, in the same way as there is an inside of a Klein bottle, because of the intersection (even though the 3D surface has only one side). To get inside from the outside you would have to remove part of the surface, just like in the case of the Klein bottle. If there was a hole to the outside, you could see it in several cross sections (3D hole), or the surface would not intersect itself (which is impossible for a closed 3D surface in 4D... the Moebius strip in 3D is an open surface). What you see as inside in the animation are slices of 4D-air.

Actually I now realise that you are correct Teragon in the sense that yes they are closed of tunnels in our 3D space. I acquiesce.
Though to balance this they are not closed off in the 4D space... Still that is good to comprehend, thank you. I told you I was still finding out things too! I find it quite fascinating.

So extending on that now, if we were 'inside' we would not be able to get out as the 4D get-away options are not available to us in our 3D space.
What might happen is that a 4D bird might fly in and we are left wondering how it got inside with us when there are no visible entry holes; and it would disappear just as mysteriously.
The 'tunnels' are open to the 4D sky but not to our 3D sky so the bird enters from the 4th dimension.
Also the atmosphere would not go stale as the air freely moves in, around, and out of the '3D' tunnel via the 4th dimension.
Though to make this 100% correct the back 'tunnel' or bulge part is open to the ana and kata directions (to get to one side or the opposite side).
It is the crimp part at the front that is actually open to the sky and to underneath.

If a 3Der where 'inside' then they couldn't walk around because they would eventually encounter the crimp.
Instead they would need for us to slowly tip the Klein strip up on its side so that the crimp moves away from them and reveals more tunnel to walk further along.
We would need to tip the Klein strip the right way. Turning the Strip the other way would cause the crimp to appear to move towards them.
In that sense I guess this is more like a Klein Bottle than a solid Klein Bottle; though in lieu there is the extra surface available by means of the 4th dimension.

How do they get inside this Klein Strip. If they stand on the front path then if we tip the Klein strip it will simply keep pushing them up until they are standing on a cylindrical section.
So this doesn't seem to be a means to get in. I think it is as you suggest Teragon. To get in would need a hole to be made.
So, unless I'm again wrong, it does appear that there is no way for us to get 'inside' (which is outside in 4D but we are only in 3D) without digging through the shell of the Klein strip that we see.
I will have to ponder this some more; I can see...

It is interesting that if we keep tipping the Klein strip for them to walk around the tunnel then they would take two loops to get back to the beginning.
They could draw some graffiti at their start point and walk around one loop and wonder who cleaned away the graffiti.
The could then continue to walk around the loop again and suddenly find that their graffiti has magically returned. Cool!

What I don't understand yet is, and here may be some crucial point, the rotation shows an object that intersects itself in a Moeubius strip, while in the sliced bread animation, the intersection obviously traces out the area of a circle. In the first case it seems like in two directions the surface flips sides, whereas in the second case it seems like only one direction. Where is the middle of the object along the dirction the cuts and taken, where is the start?

I'm not quite sure what you mean Teragon?
The rotational one shows a full 360° of views of the front of the Klein Strip while we stand in one spot.
The side-step one only shows only the one view rotation at 0° and we are moving ourself sideways into the 4th dimension while maintaining this 0° orientation.
The side-step one involves sideways movement (ana/kata) while the other involves standing on one spot and rotating.

In any case it's a nice object. Quite different from the Moebius strips I've described. When I've got all the clues together I'm going to give a systematic summary of the different onesided surfaces in 3D and 4D.

Thanks Teragon Hopefully we can get to the bottom of this so that it can be included in your summary...
Cheers!

[Teragon]

It is interesting that if we keep tipping the Klein strip for them to walk around the tunnel then they would take two loops to get back to the beginning.
They could draw some graffiti at their start point and walk around one loop and wonder who cleaned away the graffiti.
The could then continue to walk around the loop again and suddenly find that their graffiti has magically returned. Cool!

That tipping thing is an interesting aspect. I think that there is no way to pass between inside and outside (if there is no extra hole), not even for a 4D being. It would be visible in the sequence of cross sections. Otherwise the surface would have to have a 2D edge.

What I don't understand yet is, and here may be some crucial point, the rotation shows an object that intersects itself in a Moeubius strip, while in the sliced bread animation, the intersection obviously traces out the area of a circle. In the first case it seems like in two directions the surface flips sides, whereas in the second case it seems like only one direction. Where is the middle of the object along the dirction the cuts and taken, where is the start?

I'm not quite sure what you mean Teragon?
The rotational one shows a full 360° of views of the front of the Klein Strip while we stand in one spot.
The side-step one only shows only the one view rotation at 0° and we are moving ourself sideways into the 4th dimension while maintaining this 0° orientation.
The side-step one involves sideways movement (ana/kata) while the other involves standing on one spot and rotating.

That was an important information! I had interpreted it as a cross section instead of a projection before, now I can make the question more clear. The moving cross section shows an object reminiscent of a spheritorus:






Essentially, if you exchanged hight (z) for the invisible dimension (w) in this depiction, you would find a simple torus as a cross section. If you exchanged x or y with w, you'd see two ellipsoids or a sphere and a circle.

This cross section is interesting taken by itself. As a 2D shape in 3D, it would be no real Klein bottle (but still some exotic nearly closed Moebius strip), because the surface has a sharp edge at the place where both surfaces intersect each other. It's not closed at exactly two points, where the condition for a Klein bottle is hurt. So So I don't think it's closed in 4D either.

The rotation is more complicated to understand for me. What confuses me is that I can see no invariant plane in the object. Any (single) rotation should have its invariant plane, but in the animation there's only the part that's always flat staying invariant. The segment at the opposite side should also stay the same.

Moreover at a certain angle, the whole torus looks flat. To a 3D beeing. There would still be a shade and a 4Der would still perceive it in 3D. My point is, I'd expect the moving cross section of the same object to look different. Can't say exactly what it should be, because of the missing invariant plane, but something like a 2D Moebius strip changing width.

Same here, it's usually easier to learn and get food for though when there is exchange with like-minded people

[gonegahgah]

Note: I'll have a go at rewriting this tomorrow as a new post as it contains errors. I may have to retract on saying the paths are hollow, sorry Teragon. I'll explain tomorrow...

That tipping thing is an interesting aspect. I think that there is no way to pass between inside and outside (if there is no extra hole), not even for a 4D being. It would be visible in the sequence of cross sections. Otherwise the surface would have to have a 2D edge.

I'll provide a 2Der example to hopefully clarify this Teragon.

If we have a ring standing on its side with the edge facing the 2Der then they will know it as a 'tunnel' or an enclosed space.
To them there is no way to get into the ring without digging into it; or so they think.
But we can just walk into the ring from either the left or right sides through the massive hole we can see.
The entire surface of the inside of the ring is accessable from the left and ride sides to us but not to the 2Der when they can only see the edge.

Now if we take that ring and extend it circle-wise around into a torus.
To us, from our angle in 4D space, the torus is impenetrable.
However to a 4Der it is not at all inaccessable. They can access its 'insides' from the 4th dimension.

A 4D path on its side is just a series of such circular cross sections both along its length and as a series of bigger to smaller circles to describe its surface.
We see each of these inner/outer circles for a 4D path on its side as fully enclosed tunnels (when we side step in 4D) but the 4Der sees each consecutive circle as a ring which is accessable from the 4th dimension.
It is constrained in our dimension but not in theirs.

Does that help?

That was an important information! I had interpreted it as a cross section instead of a projection before, now I can make the question more clear. The moving cross section shows an object reminiscent of a spheritorus:

That is mostly correct. If you take all the side-step frames together you effectively get a 4D projection. ie a series of hollow torus segments with a crimp at front that form the entire path.
If you combine all those side step frames then each cross section will form into a filled circle. Even the crimp at the front will do this.
So, if you take each infinite small sidestep and add them together they form a circle in the following fashion:

The full circle of each cross section of the path is formed by adding all of its 3D side-by-side slices together. You can see here how both result in a circular cross-section at that point in the path.
The reasons that the slices are different is because the back cross section is on its side and the front section is flat on the ground...

Essentially, if you exchanged hight (z) for the invisible dimension (w) in this depiction, you would find a simple torus as a cross section.

That is correct Teragon for the crimp section. Doing that will mean that the Klein strip now has the crimp at the top (where the bulge was); instead of the front (now the bottom). Opposite to where it was.

If you exchanged x or y with w, you'd see two ellipsoids or a sphere and a circle.

That is just like rotating to 90° or 270°. You will now actually just see an ordinary Mobius strip.

The rotation is more complicated to understand for me. What confuses me is that I can see no invariant plane in the object. Any (single) rotation should have its invariant plane, but in the animation there's only the part that's always flat staying invariant. The segment at the opposite side should also stay the same.

I'll work on doing the tipped up angles described by ICN5D soon Teragon.

Same here, it's usually easier to learn and get food for though when there is exchange with like-minded people

Thanks Teragon. I look forward to investigating this further.

[gonegahgah]

Okay, corrections...

For a starter the following is not correct for side stepping ana/kata-wards:
(WRONG!)

The following rotational viewing is still correct where we rotate in one spot between the y and w axes:
(STILL RIGHT!)

As I've already retracted, the Klein Strip is not hollow. It is always a solid (as we think of it) torus cross-section at all times that just twists between the x,y,z axes.
It has a front aspect and a rear aspect and a top aspect and a bottom aspect from our point of view.
It also has the other 360° of sideways views - including ana and kata - that aren't directly available to us; though we can view those aspects one at a time if we rotate our 3D slice around the y and z axes.

If we consider the crimp to be at the front while the Klein Strip is horizontal then we will see:

but only when the rear is fully in our 3D slice. I shall refer to this particular rotation as 0° rotated into the 4th dimension.

When we rotate our slice around into the 4th dimension between the y and z axis the rear appears to shrink.
This is in agreement and concordance with the 'STILL RIGHT!' rotational viewing animation above.

We can also side-step into the 4th dimension without changing our x and y positions.
When we do this the front cross-section will stay the same but shrink in the y direction (and grow if we side-approach).
For the front it doesn't matter what angle we are into the 4th dimension it will shrink/grow in the same fashion; as you will find in the following images.

However, depending on our angle into the 4th dimension the rear will do different things.
The following will help to demonstrate:


These images are useful to demonstrate what the cross-section will be for the front and back as we side-step into the 4th dimension while our 3D slice is orientated differently. You can see for the images that our 3D slice is orientated at 0° in picture 1, 22.5° in picture 2, 45° in picture 3, 67.5° in picture 4 and 90° in picture 5. On the left they show what the cross section of the front will be (doesn't change) and what the cross section of the rear will be (does change for our 3D slices orientation in 4D).
Each of the lines (including ovals/circles is demonstrating a step sideways into the 4th dimension. Although each step is shown slightly side-stepped, because we are side-stepping our 3D slice; and not ourselves, each of those shown side-steps are actually all in the same place as each other. However if I drew it that way it would defeat the purpose as much of the relevant information would be difficult to see. For example, if all the vertical or horizontal lines were in place they would look like a single line and we couldn't see how the line shrinks.

You'll notice for the first picture that there is only 1 surface line (a full filled circle); their is only one side-step shown. This is because as soon as you take any step sideways into the 4th dimension we can no longer see that part of the Klein strip. It exists totally in the x and y (and z) plane - for our purposes - and has no extension in the w direction. Hence it is standing on its side to a 4Der.

If we rotate to 22.5° and side-step we find that we see an oval cross-section for the back with a slight tilt. If we take a side step this will shrink and either drop or rise a little - depending on which way we side step. But, as you can see there aren't many slices, so it quickly disappears as well before the front cross-section does.

As we repeat this, at greater orientations into the 4th dimension, we see that the ovals get skinnier and that they persist for longer as we side-step. Once we get to 90° into the 4th dimension we are looking at a Mobius Strip. When we side-step it remains a Mobius strip that gets narrower. This time the rear persists as long as the front does while we side step.

The other thing to note is that the lines are the surface. The insides of the ovals are the solid (as we think it) part of the Klein Strip.
Remember that we are looking at the Klein Strip from only one 4th dimensional direction.

[gonegahgah]

Here is a follow up image showing only the rear cuts. This time it shows them in place where they would be as we step our 3D slice sideways into the 4th dimension:

These are cross-sections of the rear of the Klein Strip. The smaller ovals within each are side-steps into the 4th dimension.
The cross on the 0° rotation is meant to show that it has no side-step views. Once you step the slightest sideways that section disappears.

None of the ovals/circles are hollow. They form part of the solid part of the Klein Strip.
The lines (forming circles) are the actual surface and by combining all of them into the 4th dimension you create the actual surface that the 4Der sees.
Just like the 4Der we can rotate into the 4th dimension to see these connected wholes but only from the various aspects rotating our slice provides.

It is the connection of the side-step lines to each other and each of these to their neighbour section lines around the Klein Strip that we tend to think of as the 'hollow' or path part of the Klein Strip.
This is what forms what we would normally think of as a volume path where you can walk 'inside' it (again 'inside' is our conception) or with any orientation.

Connecting the diagrams you can see how we 3Der's could walk on top of the Klein Strip when our slice is rotated 0° and even at other angles.
However by 90° (Mobius Strip Form) it becomes as hard for us to walk on top of the Klein Strip as it is for the 4Der to walk on the back of the Klein Strip at any time.
For them, walking across the back of the Klein Strip is like us trying to walk across the paper thin back of the Mobius Strip...

[Teragon]

That's what I meant. The two animations show very different objects. It's only the object you stated was wrong that's closed.

Ok, the right object is flat like a sheet of paper in 4D. You have applied so much efford. Essentially I just wanted to know, how the invariant plane of the rotation is oriented and where the origin of the rotation is. If I don't understand one basic point, it's inefficient to go through all the further details, maby everything else would become clear by itself if just this point was clarified.

Take a 3D object you can see. What you see is a 2D projection. If you rotate the object around your axis of sight, the projection stays the same. It just does a simple rotation around the central point, as a 2D being knows it. If you rotate it around an axis lying within the projection plane, the thing starts to morph, but no matter how you rotate it, there is always an axis along which the length of the object remains the same. If you rotate a 4D object and project it on a 3D plane (actually I prefer this term over volume in 4D), in the samer manner the object would keep its shape in one plane. This is my point. There is no invariant plane in the animation!

Meanwhile I learned a lot about Moebius bands in 3D, 4D and 5D and and found a conceptional way to visualize some of their properties. I'm going to make some figures when I have time.

[gonegahgah]

That's cool Teragon. I apologise for my confusion.
I must admit I'm not sure I understand the usage of 'invariant plane'? Could you explain that a little more for me please?

[Teragon]

Okay, a rotation always involves two dimensions. For an object described by coordinates (x,y,z) rotating in the xy-plane, the x- and y-coordinates of its points interchange in some way. For example, a point of an object might first be at (1,3,2), after 90° it's at (-3,1,2), after 180° it's at (-1,-3,2) and so on. The z-coordinate remains the same for every point as all points of the object are moving around the z-axis. All points (0,0,z) keep their position throughout the whole cycle, so the z-axis is the invariant element of the rotation. In 3D the invariant element is the axis of the rotation.



If you look at the rotating rectangle, it's only at the axis of rotation where the object has always the same length in the projection on 2D.

In 4D the invariant element is a plane. The animation below shows a 3D cube rotating inside the zw-plane and around the xy-plane. All the points of the cube are moving about the xy-plane by moving, casually said, from z to w and from there back to -z. The faces seem to change shape or position, but the horizontal cross section in the middle of the object stays always the same (the vertikal edges seem to rotate around the point where they intersect the invariant plane).

[gonegahgah]

Thanks Teragon. That was, when I read it, and is still a very interesting question.
I don't know if I understand fully yet but some things occurred to me...

First off, thank you for your patience. I thought initially that you were asking me questions to learn but I now realise that I am more the learner in our roles.

Also, I think I possibly realise now some of the confusion that may have existed between quickfur and myself when I have been explaining my 'rotational projection' approach. It may be the aspect of 'invariant planes' that was the disparity.

The idea of an 'invariant plane' was not a notion I was conversant with nor had explored before. So thank you for raising it. My brain has been mulling over it ever since you raised the question, and further provided your explanation.
It certainly needed to be addressed with what I was doing.

I don't know if the following is on the right track to answering your question but I just wanted to pass along some thoughts that occurred to me just this morning. It is always a marvel to me that we can set our brain a question and that it will look for answers unbidden while we sleep.

Let's see what the thoughts were and see if they have any relevance... (Most of this was done on the train so forgive me for any errors).

Your last post shows a square spinning around a vertical axis. Being that the square is spinning around in 3D the 'invariant' is that axis or in other words a line. That can be easily seen in that square spinning animation. The central axis is the only thing that doesn't change (except for its rotational orientation around itself). So in this case, and all cases in 3D, we have invariant lines.
(but with the axis in the x-direction; instead of z).

It occurred to me to wonder how invariant that line is – or not so how invariant but how invariable. It may be that there is other terminology for what I am describing which you can probably help me with.

It occurred to me that in our world we have left and right (as well as up/down and forward/back). However in a 2D world they don't have left or right. My answer has been to rotate our left or right into the 2Der's up and down space (as a ghosted image). In this way they can see all of the object's outlines (if not its bulk) and use a 'sideways' rotation which is just to rotate their 2D slice upwards/downwards into our available around 3D view to get the full 3D space (hopefully that makes sense).

Now, I could depict the world to the left in the up direction along with to the right in the down direction. I could alternatively depict them the opposite way around as left in the down direction with right in the up direction. It wouldn't make any difference to the 2Der as they have no sense of where left and right actually are.

If we go back to your rotating square and flipped the spinning axis 180° from left-right to right-left for the whole world it would not matter to the 2Der. To them it wouldn't change anything. So in this respect our 3D world has a perfectly flip-able extra axis (sideways to us) as far as 2Ders are concerned.

This also appears to be the case for the spinning cube in a 4D space. Except that this time there are 360° of interchangeable axis; and not just left and right. In other words you can make left be any of 360° of sideways with right being opposite to this and ana/kata being perpendicular. In the next moment you can then change left to being any other angle in that 360° of sideways with the other axis moving in correspondence.


There is no preferential left/right in 4D so it makes no difference to us. We can change left/right to being any of the 360° of opposing sideways available - with ana/kata being perpendicular - and we can do this at anytime.

I am wondering if this relates to my animation and if this supercedes invariance? Mind you I don't know that yet but hopefully?
Could I get your thoughts on that? I'll think about it more as well...

PS. Some more thoughts so far...
By changing which angle is the left-right, and their perpendicular ana-kata, it may be that we are then depicting the 3D slice as the relationship of our 3D slice to the object in 4D space with those altered base sideways angles.
The object will look different in our current slice for each perspective if we make our 3D slice's sideways angles (left/right/ana/kata) at different angles in the 4D space while maintaining our forward and vertical directions...
It would then look different in the fixed directions for each of those perspectives... Does that sound possible?
So perhaps, in our example, the one invariant would be the Mobius strip at the centre which doesn't seem to change at any change of the base sideways axis angle (although it is hidden in the bulk most the time).
How is that sounding Teragon?

Bringing it forward, here is the depiction that is in question...
[So, where is the invariant plane? Or, are we instead just rotating the base sideways axes (being left/right/ana/kata) in the 4D space?]

[gonegahgah]

Ok, the right object is flat like a sheet of paper in 4D. You have applied so much efford. Essentially I just wanted to know, how the invariant plane of the rotation is oriented and where the origin of the rotation is. If I don't understand one basic point, it's inefficient to go through all the further details, maby everything else would become clear by itself if just this point was clarified.

At this time I couldn't really point out the origin - or that's what I'm guessing - until I understand the process a little better. I foresee some distortion in the resultant image depending on where our eye is at anytime.
I'm hoping the distortion will help to depict a sense of bulk as we move but I have no idea as to whether that will be the case at the moment...

...3D plane (actually I prefer this term over volume in 4D)...

Me too. I prefer terms like 3-volume and 4-volume to distinguish between space in 3D and 4D as they will think volume has 4 dimensions when we think it has only 3.

...in the samer manner the object would keep its shape in one plane. This is my point. There is no invariant plane in the animation!

I'm tending to agree with you Teragon. I'm hoping that my previous post provides an acceptable answer...

Meanwhile I learned a lot about Moebius bands in 3D, 4D and 5D and and found a conceptional way to visualize some of their properties. I'm going to make some figures when I have time.

That will be awesome

[Teragon]

First off, thank you for your patience. I thought initially that you were asking me questions to learn but I now realise that I am more the learner in our roles.

You're welcome. It was just my own curiosity that has motivated me. As I saw something I didn't understand I couldn't tell if you had the answer.

Now, I could depict the world to the left in the up direction along with to the right in the down direction. I could alternatively depict them the opposite way around as left in the down direction with right in the up direction. It wouldn't make any difference to the 2Der as they have no sense of where left and right actually are.

This is only the case if the object is actually symmetric with respect to the plane of the 2D being, i.e. if the left side is the mirror image of the right side.
The object that's rotated through 2D space may also be asymmetric or the projection may repeat itself 3 times or more with a 360° turn. It depends on the rotational symmetry of the object with respect to the rotational axis. By rotating a higher dimensional object through a 3D cut, I can learn about its symmetries.

Fortunatly there are some nice animations on the internet that can make the concept more clear.

http://i.imgur.com/JveEJIr.webm

Here's a 3D torus rotated through a 2D plane (taken from ninedimensionalbeing.imgur.com). What you can see is, the cross section changes totally - nearly. Actually there's one thing that's unchanged through the course of the whole rotation. It's the 1D cross section from the left end to the right end in the center of the torus. So a 2D beeing living in the plane could tell that the object is being rotated around the horizontal axis (back-forward for him) and not around the vertikal axis. A 1D beeing living in this axis couldn't see any change at all because the rotation takes place exclusively in the dimensions it's not famliar with.

Here is a cyltrianglinder (same source) rotating in the left/right-ana/kata-plane. The forward/backward-up/down-plane is the invariant element of the rotation. A 2D cross section in this plane would stay the same throughout the rotation, because the dimensions involved in the rotation lie outside the invariant element.

http://i.imgur.com/6bVotNg.gif

This also appears to be the case for the spinning cube in a 4D space. Except that this time there are 360° of interchangeable axis; and not just left and right. In other words you can make left be any of 360° of sideways with right being opposite to this and ana/kata being perpendicular. In the next moment you can then change left to being any other angle in that 360° of sideways with the other axis moving in correspondence.

There is no preferential left/right in 4D so it makes no difference to us. We can change left/right to being any of the 360° of opposing sideways available - with ana/kata being perpendicular - and we can do this at anytime.

I am wondering if this relates to my animation and if this supercedes invariance? Mind you I don't know that yet but hopefully?
Could I get your thoughts on that? I'll think about it more as well...

Not sure what you mean exactly, gonegahgah. There's also a full 360° for a rotation in 2D. What's always invariant (if the cross section also lies there) is the center of the rotation... the center between left, right, ana and kata, which is 2D in this case. Any further invariance is because of the symmetries of the object.

So perhaps, in our example, the one invariant would be the Mobius strip at the centre which doesn't seem to change at any change of the base sideways axis angle (although it is hidden in the bulk most the time).
How is that sounding Teragon?

If the band was untwisted that would be what I expected. But here, no, it's always a plane, if you think about it. Otherwise on the right side x would change parts with w, in the center it would be z and on the left side it would be y.
Three possibilities come to my mind:
- The center of the rotation is not at the center of the object, but at one side
- The center of the rotation is outside the 3-plane of the cross section
- It's a double rotation

[gonegahgah]

This is a very exciting discussion. I'm not sure where I will end up but it is still invaluable for me...
I thought I might go to where we have common ground first if that is okay, Teragon. Or, to make sure I understand what you are saying.

I made the two following diagrams to depict part of two shapes that should hopefully be able to exist in 4D:

This shows multiple 3D slices of a 4D world with part of a shape shown inside spread across those slices.
The one on the left is just the front and back of several Mobius strips that form a circle cross section across the slices. If you connect them via a twisted ring they would be complete. See the following diagram.
The one on the right is similar but the front parts just connect across the 3D slices to the full circle in the single slice.

Here is a picture showing a combination of the Mobius strips. Each colour represents a different 3D slice:

I've stepped them slightly so that you can see them more clearly but just assume they are superimposed but off in the ana-kata directions along the centre of each mobius strip in each slice that is a part of the whole.

First off, can we discuss or agree on whether these are true representations of two possible shapes in 4D... Please feel free to ask me to clarify anything about them.
Remember the top two are just the front and back with the connection missing and the last picture shows the first one as it should be connected.

How does this look?

[Teragon]

Yeah, agreed, it's a possible moebius band in 4D.

[Teragon]

Now that I've learned which object you are referring to, I can see that every snap-shot of your animation makes sense. The way both distinctive images are related to each other has led me to the conclusion that the animation is showing the rotation of a toroidal vortex:



In the animation above the visible cross section would lie horizontally at the center of the vortex, extending in another dimension unaffected by the rotation. The inside/outside direction of the strip (sometimes x, sometimes z according to your labeling) is exchanged by the w direction (up/down in the animation above). What do you think about that?

[ICN5D]

Here's the cyltrianglinder animations you were looking for, Teragon,

[gonegahgah]

I love your animation Teragon. That looks fantastic!

It is my suspicion that what you are saying is occurring. I very much want to determine whether it has validity or not. That is why I am delighted with your questions because they are making me examine, and even hopefully understand, it more fundamentally.

With your last post you're jumping ahead of where I've gotten to so I hope you don't mind if I continue to take it a little more slowly while engaging your feedback so that I can fully understand it myself.

When I get home - where my computer drawing tools are - I'll do some more depictions of my last two pairs of images; to hopefully help with this process. I also wanted to discuss this orientation aspect further ie the left-right swapping orientations compared to the greater range of 4D orientations. I'll get back soon...

[gonegahgah]

Here is a filled in depiction on the right of the skeleton depiction I gave previously on the left:

The only problem is that it obscures; rather than helping to clarify; so I think I'll stick to the skeleton diagrams.
I'll just go and try to get "3D Grapher" depict what the following would look like if we rotate the object itself (and not our perspective which is what I'm heading to) into the vertical...

[gonegahgah]

Now, I could depict the world to the left in the up direction along with to the right in the down direction. I could alternatively depict them the opposite way around as left in the down direction with right in the up direction. It wouldn't make any difference to the 2Der as they have no sense of where left and right actually are.

This is only the case if the object is actually symmetric with respect to the plane of the 2D being, i.e. if the left side is the mirror image of the right side.
The object that's rotated through 2D space may also be asymmetric or the projection may repeat itself 3 times or more with a 360° turn. It depends on the rotational symmetry of the object with respect to the rotational axis. By rotating a higher dimensional object through a 3D cut, I can learn about its symmetries.

I'll wait until tomorrow morning to upload some animations when I'm on offpeak internet.
Meanwhile I thought I might look at this. Either we are talking about different things or we need to clarify this one Teragon.

Here is a diagram showing a 2Der looking at a triangle that is standing up at an angle and passing through their 2D plane.

Using 'rotational projection' I've projected the whole triangle into the 2Der's plane.

The process is to:
1. find the angle a point on the object is off into 3D from the forward direction ie. angle = arctan(x/y)
2. start at the y,z point and rotate around the common origin for angle either up or down.
3. do this for all points and join them together (all points should be rotated up if on left and down if on right; or the vice-versa of this but it should be consistent).
I'll draw the process soon.

The key thing is that the rotation can be up or down but with only one corresponding to left or right at a time. It doesn't matter. The 2Der is not able to say which way is the preferred left or which way is the preferred right.
And if need be it is easy to flip this usage...

The same would occur for us if we were able to magically transfer our whole body from left to right.
Suddenly our brain would see what was on the left as being on the right and vice-versa (this is nothing to do with VRI in case anyone is watching).

Sadly we can't do that but a 4Der can. Also, if we were in a 4D space we could do it to by rotating our 3D slice around 180°. We could flip our body 180° effectively so that left is now right and right is now left.
Things up high will still be in that direction and things further in the distance will still be further in the distance.
The only thing that will have changed is that we are now seeing everything in reverse in our sideways direction.

Please let me know if any of that needs clarification or if any of that is incorrect?

[Teragon]

Thanks, ICN5D. I haven't noticed that the link was the same both times. I changed it in my original post.

[Teragon]

The key thing is that the rotation can be up or down but with only one corresponding to left or right at a time. It doesn't matter. The 2Der is not able to say which way is the preferred left or which way is the preferred right.
And if need be it is easy to flip this usage...

The same would occur for us if we were able to magically transfer our whole body from left to right.
Suddenly our brain would see what was on the left as being on the right and vice-versa (this is nothing to do with VRI in case anyone is watching).

Sadly we can't do that but a 4Der can. Also, if we were in a 4D space we could do it to by rotating our 3D slice around 180°. We could flip our body 180° effectively so that left is now right and right is now left.
Things up high will still be in that direction and things further in the distance will still be further in the distance.
The only thing that will have changed is that we are now seeing everything in reverse in our sideways direction.

You have to keep in mind that such a turn in 4D involves an exchange of ana and kata. You can change left and right in 3D too if you turn head over heals. In this case up and down are exchanged. For us 3D beings a 180° through ana/kata would seem like a mirror operation, if that is what you mean.

Again not sure what your point is, I thought we were talking about invariant planes. Concerning your deliberations I would say:
If a 3D object is rotated through a 2D plane, a 2D beeing inside of it may not decide if it's going to the left side or to the right side, but
- it's able to tell if its sense of rotation changes if the object looks different on the left side and the right side, i.e. the 2D plane is not a mirror plane of the object.
- it's able to tell if the object has been flipped by 180° if the rotational axis is not a "mirror axis" of the object, because two directions in the plane will changes parts.

I'd like to make some words about the 3-surface of the object and it's normal vector, because there were some misconceptions about it.



One the lefhand side the surface normal vector lies fully inside our cross section. What we see as flat region is actually a cross section orthogonal to the surface, with part of the edge as a boundary on both sides. Going in any direction along the strip, the normal vector turns into the hidden w-direction. What I have shown is just its component in the (x,y,z)-direction. The directions involved in the twist here are just up/down and ana/kata. On the right side the normal vector points wholey into the w direction, so we see a tangential cut through the 3-surface now. In return we see the whole volume of the surface and the whole area of the edge now.

[gonegahgah]

You have to keep in mind that such a turn in 4D involves an exchange of ana and kata. You can change left and right in 3D too if you turn head over heals. In this case up and down are exchanged. For us 3D beings a 180° through ana/kata would seem like a mirror operation, if that is what you mean.
Again not sure what your point is, I thought we were talking about invariant planes. Concerning your deliberations I would say:
If a 3D object is rotated through a 2D plane, a 2D beeing inside of it may not decide if it's going to the left side or to the right side, but
- it's able to tell if its sense of rotation changes if the object looks different on the left side and the right side, i.e. the 2D plane is not a mirror plane of the object.
- it's able to tell if the object has been flipped by 180° if the rotational axis is not a "mirror axis" of the object, because two directions in the plane will changes parts.

There are two reasons why I'm exploring this idea Teragon. I'll just explain the first for the moment and look at the second tomorrow hopefully... I think the second may relate to our searching for the "invariant planes".
The first reason is I want to explain why the object seems to remain basically in one spot while there are obviously changes occurring. I'll try to add some more depictions tomorrow towards this.

One the lefhand side the surface normal vector lies fully inside our cross section. What we see as flat region is actually a cross section orthogonal to the surface, with part of the edge as a boundary on both sides. Going in any direction along the strip, the normal vector turns into the hidden w-direction. What I have shown is just its component in the (x,y,z)-direction. The directions involved in the twist here are just up/down and ana/kata. On the right side the normal vector points wholey into the w direction, so we see a tangential cut through the 3-surface now. In return we see the whole volume of the surface and the whole area of the edge now.

Your words sound good to me Teragon.

I'm curious to see if I can depict the following with "3D Grapher' in the same way I depicted the other one...