Binomials and n-cube cutting
22 posts
• Page 1 of 1
Binomials and n-cube cutting
Hello, Greetings and Salutations from me to this forum!
I'm brand new to this forum and thought I'd dive straight in to see what results I'd get...
I've been slowly advancing my knowledge and insight of higher-dimensional spaces and thought I'd write and take advantage of this forum as I have a question or two that I hope somebody would be able to answer or offer insight into. It relates to hypercubes and geometrical renderings of binomials.
If we take two arbitrary 1D lengths - say, 'a' for the shorter 1D length, and 'b' for the longer - add them and raise the result to the power of the dimension number - (a+b)1 - we simply get (a+b). Nothing exciting to see here (at least not for me at this time in my life.)
If we take our (a+b) and raise it to the power of 2, we get (a+b)2 = a2 + 2ab +b2. So, a2 is a small square, b2 is a big square and the two ab's are rectangles whose side lengths are a and b (thus giving us an area of a x b) - four elements in total.
When we cube our (a+b), we get a3 + 3.a2.b + 3.a.b2 + b3 wherein we see one small cube (a3), one big cube (b3), three long thin square prisms (a2.b) and three squat and fat big square prisms (a.b2) - eight elements in total.
Now, when we move on to (or in to?) R4, we have (a+b)4 = a4 + 4.a3.b + 6.a2.b2 + 4.a.b3 + b4. Therefore, we have one small tesseract (a4), one large tesseract (b4), four "long, thin" cubic hyperprisms (a3.b), four "large flat/squat" cubic hyperprisms (a.b3), and six of something else (a2.b2). Now we have sixteen elements in total.
It's clear to me that the elements raised to the 4th power are the small and large tesseracts, the following two sets of elements are hyperprisms since the "caps" are components that are raised to the power of three, which in turn are multiplied by a 1D component.
HOWEVER (!), the final set of six - the a2.b2 elements - are somewhat of a mystery to me since these objects seem to be constructed from two polygons/squares (of different sizes) - which I assume are orthogonal to each other. Do the 'a' square and 'b' square create a hyperprism or a duoprism or perhaps something else? And are we dealing with a Cartesian product as the result or perhaps Minkowski sums? I'm little stumped here and searching the Internet hasn't yielded any answers for me (yet).
In fact, in our 4D geometrical binomial, are all the other elements Cartesian products?
These questions came up for me when I was revising some high school algebra and extended it to R4.
I'd be so grateful if anyone could share with me their wisdom and insights.
Best regards:
Sobieski
[The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.]
PS: I'm in the process of slowly reading all the posts in this forum and find it all so interesting... What a great resource! Kudos to the creator and contributing members!
I'm brand new to this forum and thought I'd dive straight in to see what results I'd get...
I've been slowly advancing my knowledge and insight of higher-dimensional spaces and thought I'd write and take advantage of this forum as I have a question or two that I hope somebody would be able to answer or offer insight into. It relates to hypercubes and geometrical renderings of binomials.
If we take two arbitrary 1D lengths - say, 'a' for the shorter 1D length, and 'b' for the longer - add them and raise the result to the power of the dimension number - (a+b)1 - we simply get (a+b). Nothing exciting to see here (at least not for me at this time in my life.)
If we take our (a+b) and raise it to the power of 2, we get (a+b)2 = a2 + 2ab +b2. So, a2 is a small square, b2 is a big square and the two ab's are rectangles whose side lengths are a and b (thus giving us an area of a x b) - four elements in total.
When we cube our (a+b), we get a3 + 3.a2.b + 3.a.b2 + b3 wherein we see one small cube (a3), one big cube (b3), three long thin square prisms (a2.b) and three squat and fat big square prisms (a.b2) - eight elements in total.
Now, when we move on to (or in to?) R4, we have (a+b)4 = a4 + 4.a3.b + 6.a2.b2 + 4.a.b3 + b4. Therefore, we have one small tesseract (a4), one large tesseract (b4), four "long, thin" cubic hyperprisms (a3.b), four "large flat/squat" cubic hyperprisms (a.b3), and six of something else (a2.b2). Now we have sixteen elements in total.
It's clear to me that the elements raised to the 4th power are the small and large tesseracts, the following two sets of elements are hyperprisms since the "caps" are components that are raised to the power of three, which in turn are multiplied by a 1D component.
HOWEVER (!), the final set of six - the a2.b2 elements - are somewhat of a mystery to me since these objects seem to be constructed from two polygons/squares (of different sizes) - which I assume are orthogonal to each other. Do the 'a' square and 'b' square create a hyperprism or a duoprism or perhaps something else? And are we dealing with a Cartesian product as the result or perhaps Minkowski sums? I'm little stumped here and searching the Internet hasn't yielded any answers for me (yet).
In fact, in our 4D geometrical binomial, are all the other elements Cartesian products?
These questions came up for me when I was revising some high school algebra and extended it to R4.
I'd be so grateful if anyone could share with me their wisdom and insights.
Best regards:
Sobieski
[The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.]
PS: I'm in the process of slowly reading all the posts in this forum and find it all so interesting... What a great resource! Kudos to the creator and contributing members!
-

Sobieski - Mononian
- Posts: 12
- Joined: Sat Oct 12, 2013 9:24 pm
- Location: Ankara, Turkey
Re: Binomials and n-cube cutting
a2.b2 will be a duoprism, it is the Cartesian product of a small square and a large square. Welcome to the forum!
Whale Kumtu Dedge Ungol.
- Polyhedron Dude
- Trionian
- Posts: 196
- Joined: Sat Nov 08, 2003 7:02 am
- Location: Texas
Re: Binomials and n-cube cutting
Many thanks, Polyhedron Dude. 

I assumed correctly then about the duoprisms.
Right now I'm on a learning curve with regard to duoprisms and duo-extruded 4-polytopes. It's an interesting area of knowledge. After consulting a Wiki article on duoprisms (http://en.wikipedia.org/wiki/Duoprism), I found that the good ol' tesseract is a 4-4 duoprism - so I assume that hypercubes/n-cubes form a subset of a larger superset of duoprisms and/or "prismic hyperprisms" (not sure of where everything lies in the taxonomical structures and hierarchies yet).
Since the a2.b2 objects are established to be duoprisms, can we give them a useful name? Such as 4-4 duoprism?
Moreover, just out of curiosity, if a = b, then a2 would equal b2. Would a Cartesian product of these two objects (a2 and b2) produce a tesseract in the same way an extrusion along the w-axis of a 3-cube (either a3 or b3)?
Are there any visual representations (especially as Schlegel diagrams) of a square duoprism wherein the two squares differ in size? Perhaps it's just a stretched out or squashed tesseract... I tried scouring the Net and couldn't find what I was looking for, but I'm not sure if I'm lookng hard enough...
So many questions!! So little time!!! The quest continues...


I assumed correctly then about the duoprisms.
Right now I'm on a learning curve with regard to duoprisms and duo-extruded 4-polytopes. It's an interesting area of knowledge. After consulting a Wiki article on duoprisms (http://en.wikipedia.org/wiki/Duoprism), I found that the good ol' tesseract is a 4-4 duoprism - so I assume that hypercubes/n-cubes form a subset of a larger superset of duoprisms and/or "prismic hyperprisms" (not sure of where everything lies in the taxonomical structures and hierarchies yet).
Since the a2.b2 objects are established to be duoprisms, can we give them a useful name? Such as 4-4 duoprism?
Moreover, just out of curiosity, if a = b, then a2 would equal b2. Would a Cartesian product of these two objects (a2 and b2) produce a tesseract in the same way an extrusion along the w-axis of a 3-cube (either a3 or b3)?
Are there any visual representations (especially as Schlegel diagrams) of a square duoprism wherein the two squares differ in size? Perhaps it's just a stretched out or squashed tesseract... I tried scouring the Net and couldn't find what I was looking for, but I'm not sure if I'm lookng hard enough...

So many questions!! So little time!!! The quest continues...

- Sobieski
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
-

Sobieski - Mononian
- Posts: 12
- Joined: Sat Oct 12, 2013 9:24 pm
- Location: Ankara, Turkey
Re: Binomials and n-cube cutting
duoprisms are usually named like that - aka 3,5 - duoprism, or 4,4 - duoprism. for P2 cases, we usually say P - duoprism. Sometimes we use the name of the polygons, like triangular duoprism, or pentagon, hexagon - duoprism. I even have a few short names for them:
3 - duoprism - triddip
3,4 - duoprism - tisdip
3,6 - duoprism - thiddip
The 4,4 - duoprism would make a tesseract if the squares are the same size. I sometimes use large and small font to denote different edge lengths in duoprisms, like 3,3 - duoprism.
It should be easy to draw a projection of the 4,4 - duoprism. Start by drawing 4 small diamonds (squares standing on corner) in the pattern of a large square, like this:
Then connect them together like a tesseract projection.
The 4,4 - duoprism can be thought of as a tesseract with two of its dimensions squeezed or expanded.
3 - duoprism - triddip
3,4 - duoprism - tisdip
3,6 - duoprism - thiddip
The 4,4 - duoprism would make a tesseract if the squares are the same size. I sometimes use large and small font to denote different edge lengths in duoprisms, like 3,3 - duoprism.
It should be easy to draw a projection of the 4,4 - duoprism. Start by drawing 4 small diamonds (squares standing on corner) in the pattern of a large square, like this:
- Code: Select all
<> <>
<> <>
Then connect them together like a tesseract projection.
The 4,4 - duoprism can be thought of as a tesseract with two of its dimensions squeezed or expanded.
Whale Kumtu Dedge Ungol.
- Polyhedron Dude
- Trionian
- Posts: 196
- Joined: Sat Nov 08, 2003 7:02 am
- Location: Texas
Re: Binomials and n-cube cutting
Many thanks again, PD. Your insights are helping me a lot.
So, from the four diamands we get something that looks like a square ring-ling structure in the projection? (Connecting nodes to make a tesseract projection isn't exactly instinctual for me just yet...) What I managed to draw (by making the measurements of the a-square and b-square as large as necessary) has four rays/edges per vertex, so I'm certain it's correct. It seems that such a 4-4 duoprism is a type of distorted tesseract not too dissimlar to the cubic hyperprism? I consulted a page (http://eusebeia.dyndns.org/4d/duoprism) on the Net, but it didn't yield the answers I was seeking. Moreover, has anyone ever made an animation of the 4-4?
What would yield more insights would be looking at the nets of these objects after the 4-cube is cut as per our binomial expansion.
I've seen these strange names for duoprisms and other exotic polytopes. For me they don't get the juices flowing. I prefer a nomenclature with numbers and descriptive wording that following the rules of a naming paradigm.
So, from the four diamands we get something that looks like a square ring-ling structure in the projection? (Connecting nodes to make a tesseract projection isn't exactly instinctual for me just yet...) What I managed to draw (by making the measurements of the a-square and b-square as large as necessary) has four rays/edges per vertex, so I'm certain it's correct. It seems that such a 4-4 duoprism is a type of distorted tesseract not too dissimlar to the cubic hyperprism? I consulted a page (http://eusebeia.dyndns.org/4d/duoprism) on the Net, but it didn't yield the answers I was seeking. Moreover, has anyone ever made an animation of the 4-4?
What would yield more insights would be looking at the nets of these objects after the 4-cube is cut as per our binomial expansion.
I've seen these strange names for duoprisms and other exotic polytopes. For me they don't get the juices flowing. I prefer a nomenclature with numbers and descriptive wording that following the rules of a naming paradigm.
- Sobieski
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
-

Sobieski - Mononian
- Posts: 12
- Joined: Sat Oct 12, 2013 9:24 pm
- Location: Ankara, Turkey
Re: Binomials and n-cube cutting
Here is a net of the 4,4 - duoprism that I rendered with Stella4D, hope this helps.
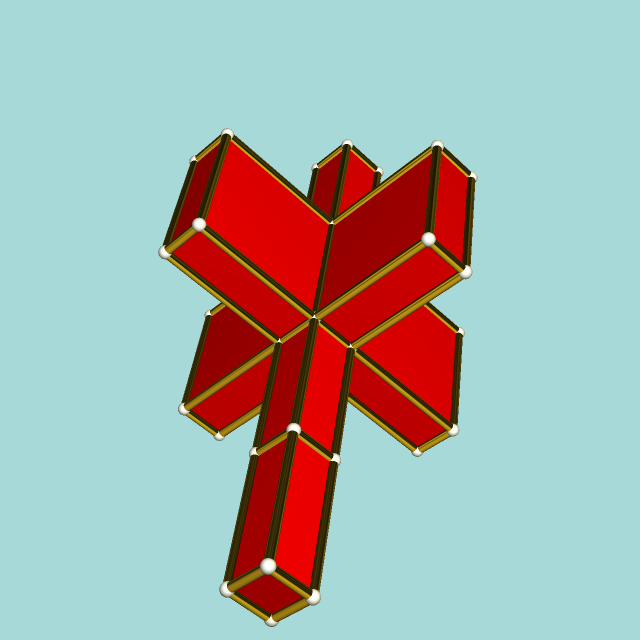

Whale Kumtu Dedge Ungol.
- Polyhedron Dude
- Trionian
- Posts: 196
- Joined: Sat Nov 08, 2003 7:02 am
- Location: Texas
Re: Binomials and n-cube cutting
Oh yes indeed. It DOES help. Thank you again. As they say: a picture speaks a thousand words. I can't imagine how many words it would speak if we actually had access to that extra degree of freedom and saw quadric pictures drawn onto (into?) 3D hypersurfaces... Would a hyperpicture in R4 speak (sqrt(1000))3 words?? (Just some frivolous speculation there...) 
Just how easy is it to create these visualizations with Stella? I've heard a lot about it and seen it in use on YouTube. I've even tried looking for a pirated/cracked version to download but we won't go there... Nevertheless, I would really like to get into rendering higher-dimensional objects with whatever programs are available as others have done. It'll be yet another learning curve...
but we won't go there... Nevertheless, I would really like to get into rendering higher-dimensional objects with whatever programs are available as others have done. It'll be yet another learning curve... 
I was wondering if it's not too much trouble whether you could make a visual rendering of a net or nets of the hyperprisms (i.e., the a3.b and a.b3 components of the binomial expansion) bearing in mind that the 'a' linear measurement is the shorter and 'b' the longer. I can visualize it more or less mentally but I would love to see renderings of it/them in physical reality. Of interest would be to see the qualitative differences between the cubic hyperprisms and the duoprisms.
BTW, thanks for following this thread

Just how easy is it to create these visualizations with Stella? I've heard a lot about it and seen it in use on YouTube. I've even tried looking for a pirated/cracked version to download
 but we won't go there... Nevertheless, I would really like to get into rendering higher-dimensional objects with whatever programs are available as others have done. It'll be yet another learning curve...
but we won't go there... Nevertheless, I would really like to get into rendering higher-dimensional objects with whatever programs are available as others have done. It'll be yet another learning curve... 
I was wondering if it's not too much trouble whether you could make a visual rendering of a net or nets of the hyperprisms (i.e., the a3.b and a.b3 components of the binomial expansion) bearing in mind that the 'a' linear measurement is the shorter and 'b' the longer. I can visualize it more or less mentally but I would love to see renderings of it/them in physical reality. Of interest would be to see the qualitative differences between the cubic hyperprisms and the duoprisms.
BTW, thanks for following this thread

- Sobieski
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
-

Sobieski - Mononian
- Posts: 12
- Joined: Sat Oct 12, 2013 9:24 pm
- Location: Ankara, Turkey
Re: Binomials and n-cube cutting
a3b and ab3 should be easy to do, I'll do the renders of those also. Stella is quite easy to use and fun to play around with. All of the uniform polychora can be explored with it. BTW. I was the one who gave Robert Webb (the designer of Stella4D) the list of these polychora  . The 4,4 - duoprism was done by writing an OFF file with a list of its 16 vertices (with four number representing the x,y,z,w coordinates) and Stella provided the convex hull of these. The file looked like this:
. The 4,4 - duoprism was done by writing an OFF file with a list of its 16 vertices (with four number representing the x,y,z,w coordinates) and Stella provided the convex hull of these. The file looked like this:
4OFF
16 0 0 0
1 1 3 3
-1 1 3 3
1 -1 3 3
-1 -1 3 3
1 1 -3 3
-1 1 -3 3
1 -1 -3 3
-1 -1 -3 3
1 1 3 -3
-1 1 3 -3
1 -1 3 -3
-1 -1 3 -3
1 1 -3 -3
-1 1 -3 -3
1 -1 -3 -3
-1 -1 -3 -3
 . The 4,4 - duoprism was done by writing an OFF file with a list of its 16 vertices (with four number representing the x,y,z,w coordinates) and Stella provided the convex hull of these. The file looked like this:
. The 4,4 - duoprism was done by writing an OFF file with a list of its 16 vertices (with four number representing the x,y,z,w coordinates) and Stella provided the convex hull of these. The file looked like this:4OFF
16 0 0 0
1 1 3 3
-1 1 3 3
1 -1 3 3
-1 -1 3 3
1 1 -3 3
-1 1 -3 3
1 -1 -3 3
-1 -1 -3 3
1 1 3 -3
-1 1 3 -3
1 -1 3 -3
-1 -1 3 -3
1 1 -3 -3
-1 1 -3 -3
1 -1 -3 -3
-1 -1 -3 -3
Whale Kumtu Dedge Ungol.
- Polyhedron Dude
- Trionian
- Posts: 196
- Joined: Sat Nov 08, 2003 7:02 am
- Location: Texas
Re: Binomials and n-cube cutting
Does Stella do animations?
- Sobieski
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
-

Sobieski - Mononian
- Posts: 12
- Joined: Sat Oct 12, 2013 9:24 pm
- Location: Ankara, Turkey
Re: Binomials and n-cube cutting
Yes it does. When in use you can watch any of the polychora go through their sections, or watch projections spin in all sorts of ways. Here are the a3b and ab3:

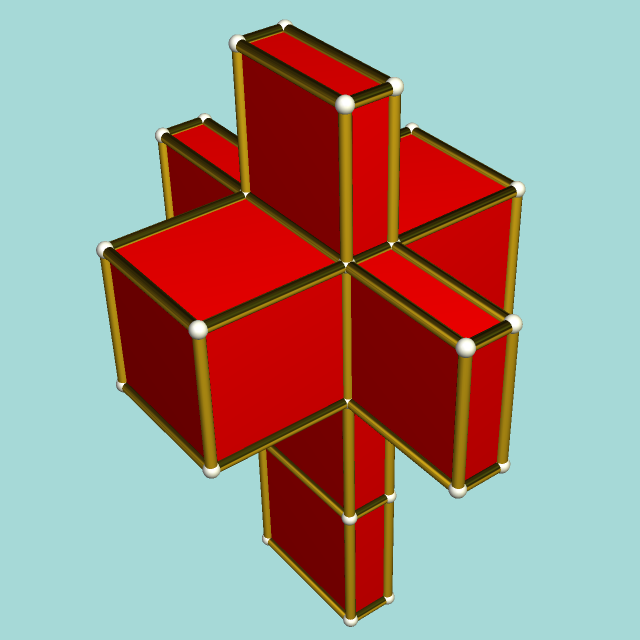


Whale Kumtu Dedge Ungol.
- Polyhedron Dude
- Trionian
- Posts: 196
- Joined: Sat Nov 08, 2003 7:02 am
- Location: Texas
Re: Binomials and n-cube cutting
Oh my!! That was quick. 
Just as I imagined and (logically surmised) they would look.
OK then. Let's cut to the chase and expand a trinomial. So, the tesseract is cut not in two along each axis, but three.
Given that a < b < c in terms of linear measurement, we have a trinomial (a+b+c)4 = a4 + b4 +c4 + 4(a3b + a3c +b3c) + 4(ab3 + ac3 + bc3) + 6(a2b2 + a2c2 + b2c2) + 12(a2bc + ab2c + abc2)
In the result, the terms containing 4th, 3rd by 1st and 2nd by 2nd powers are familiar to us and we know exactly what they represent and how they render/visualize physically. Now, in the purple at the end of the expansion we have 36 exotic objects - squares (a2, b2, c2) cross multiplied with rectangles (bc, ac, ab). I suppose we assume the linear (degree 1) elements will render rectangles that'll be part of a cross product operation with the squares (degree 2 elements)? Or is there some other more exotic mathematical operation at work here

Just as I imagined and (logically surmised) they would look.
OK then. Let's cut to the chase and expand a trinomial. So, the tesseract is cut not in two along each axis, but three.
Given that a < b < c in terms of linear measurement, we have a trinomial (a+b+c)4 = a4 + b4 +c4 + 4(a3b + a3c +b3c) + 4(ab3 + ac3 + bc3) + 6(a2b2 + a2c2 + b2c2) + 12(a2bc + ab2c + abc2)
In the result, the terms containing 4th, 3rd by 1st and 2nd by 2nd powers are familiar to us and we know exactly what they represent and how they render/visualize physically. Now, in the purple at the end of the expansion we have 36 exotic objects - squares (a2, b2, c2) cross multiplied with rectangles (bc, ac, ab). I suppose we assume the linear (degree 1) elements will render rectangles that'll be part of a cross product operation with the squares (degree 2 elements)? Or is there some other more exotic mathematical operation at work here

- Sobieski
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
-

Sobieski - Mononian
- Posts: 12
- Joined: Sat Oct 12, 2013 9:24 pm
- Location: Ankara, Turkey
Re: Binomials and n-cube cutting
I'll be leaving the cutting the tesseract into four for a later time... 

- Sobieski
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
-

Sobieski - Mononian
- Posts: 12
- Joined: Sat Oct 12, 2013 9:24 pm
- Location: Ankara, Turkey
Re: Binomials and n-cube cutting
One way to think of these tesseractic blocks is to consider them as A x B x C x D blocks, where A,B,C,D are various measures. For the A2BC, we would have an A x A x B x C tesseractic block, this would be the same as a square times a larger rectangle. For the ABC2, we have an A x B x C x C block which is a large square times a small rectangle. Finally the AB2C block would be an A x B x B x C, which would be a square times a rectangle with a longer edge and a smaller edge.
Whale Kumtu Dedge Ungol.
- Polyhedron Dude
- Trionian
- Posts: 196
- Joined: Sat Nov 08, 2003 7:02 am
- Location: Texas
Re: Binomials and n-cube cutting
So in essence, when the Tesseract is cut into three (or into any number for that matter), we tend to end up with smaller duoprismic objects which comprise the superset containing tesseracts and cubic hyperprisms. Am I correct in my assumption about measure polytope taxonomy? Judging by what I see from the Stella pics you posted, all I can assume is that cutting a tesseract would produce mostly a cornucopia of streched out and/or squished tesseracts (4-4 duoprisms).
Nevertheless I am curious at to what the cross product of a sqaure and rectangle would look like since we have hitherto only been dealing with regular squares and not rectangles. Could we see nets of these abc objects? (If it's not too much trouble, of course )
)
(I'll be getting a copy of my own full version of Stella 4D as soon as I pay off my credit card debt )
)
Nevertheless I am curious at to what the cross product of a sqaure and rectangle would look like since we have hitherto only been dealing with regular squares and not rectangles. Could we see nets of these abc objects? (If it's not too much trouble, of course
 )
)(I'll be getting a copy of my own full version of Stella 4D as soon as I pay off my credit card debt
 )
)- Sobieski
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
-

Sobieski - Mononian
- Posts: 12
- Joined: Sat Oct 12, 2013 9:24 pm
- Location: Ankara, Turkey
Re: Binomials and n-cube cutting
Yep, right you are.
--- rk
- Just as the square is nothing but a special rectangle (in fact a line-duoprism),
- and the cube is nothing but a special brick (in fact a line-triprism),
- the tesseract likewise will be nothing but a special line-tetraprism.
--- rk
- Klitzing
- Pentonian
- Posts: 1649
- Joined: Sun Aug 19, 2012 11:16 am
- Location: Heidenheim, Germany
Re: Binomials and n-cube cutting
Ah yes! It makes sense.
So, keeping things orthogonal and regular as possible, we see that
- line intervals are point-prisms (x) (only one possibility);
- a square (x2) /rectangle (xy) are line prisms (two possibilities);
- a cube (x3)/square prism (x2y)/rectangular prism (xyz) are square/rectangle extrusions (three possiblities).
It would, therefore, follow that there would be four varieties/subspecies of animals in our geometric zoo of the genus/class/family of n-prisms:
But I find FIVE:
- totally regular tesseracts (x4) - all edges equal;
- cubic hyperprisms (x3y) - 3 edges equal, 1 different;
- duoprisms (x2y2) - 2 edges equal, the other 2 equal but different from former set;
- a square-rectangle Cartesian product thingy (x2yz) (at least that's how I interpret it for now) - 2 edges equal, other 2 different; and
- an object whose side lengths are all unequal (xyzw) - a run-of-the-mill "line-tetraprism"/Cartesian product of two differently-sized rectangles.
My logic appears to be breaking down at this point. Given that if we had a 4D measure polytope, we could discount the non-commutativity of the Cartesian product since our 4D object is rigid in R4. But why FIVE subspecies that are topologically identical but qualitatively distinct in their metrics and not four? What am I missing?
A point may be extruded any length - say x, y, z or w linear units as above. There is only one distinct polytope that arises from this operation, namely {} (in Schlaefli notation). When the line interval is extruded, there are two possibilities: extrude it as far as exactly the length of the line interval to produce a square ({4}) or fall short or overshoot the interval length to produce a rectangle. So here we have two metrically distinct types of entities in R2. We can then take these two toplogically identical but metrically distinct 2D objects and extrude again. The square extruded to exactly the length of the square will of course render us a cube; undershoot or overshoot the extrusion and we end up with a square prism. If we extrude the rectangle, we can extrude it to three possible lengths, namely to one of the side lengths of the rectangle or to neither side length; in the cases of extruding exactly to either side length, the operation will produce square prisms that are metrically identical in a qualitative sense to the overshooting or undershooting of the extrusion of the square. If we undershoot or overshoot the extrusion and avoid either side length measurement, then the object will have 3 distinct orthogonal measurements. Nothing more can be done here and as a result we have produced 3 distinct subpecies in R3: a cube, a square prism and a rectangular prism. I see a pattern and a correlation here: in R1 - only 1 possibility; R2 - 2 possibilities; and R3 - 3 possibilities.
I do believe I've discovered a hitherto unknown hole in my knowledge. Or have I nesciently erred somewhere?
So, keeping things orthogonal and regular as possible, we see that
- line intervals are point-prisms (x) (only one possibility);
- a square (x2) /rectangle (xy) are line prisms (two possibilities);
- a cube (x3)/square prism (x2y)/rectangular prism (xyz) are square/rectangle extrusions (three possiblities).
It would, therefore, follow that there would be four varieties/subspecies of animals in our geometric zoo of the genus/class/family of n-prisms:
But I find FIVE:

- totally regular tesseracts (x4) - all edges equal;
- cubic hyperprisms (x3y) - 3 edges equal, 1 different;
- duoprisms (x2y2) - 2 edges equal, the other 2 equal but different from former set;
- a square-rectangle Cartesian product thingy (x2yz) (at least that's how I interpret it for now) - 2 edges equal, other 2 different; and
- an object whose side lengths are all unequal (xyzw) - a run-of-the-mill "line-tetraprism"/Cartesian product of two differently-sized rectangles.
My logic appears to be breaking down at this point. Given that if we had a 4D measure polytope, we could discount the non-commutativity of the Cartesian product since our 4D object is rigid in R4. But why FIVE subspecies that are topologically identical but qualitatively distinct in their metrics and not four? What am I missing?
A point may be extruded any length - say x, y, z or w linear units as above. There is only one distinct polytope that arises from this operation, namely {} (in Schlaefli notation). When the line interval is extruded, there are two possibilities: extrude it as far as exactly the length of the line interval to produce a square ({4}) or fall short or overshoot the interval length to produce a rectangle. So here we have two metrically distinct types of entities in R2. We can then take these two toplogically identical but metrically distinct 2D objects and extrude again. The square extruded to exactly the length of the square will of course render us a cube; undershoot or overshoot the extrusion and we end up with a square prism. If we extrude the rectangle, we can extrude it to three possible lengths, namely to one of the side lengths of the rectangle or to neither side length; in the cases of extruding exactly to either side length, the operation will produce square prisms that are metrically identical in a qualitative sense to the overshooting or undershooting of the extrusion of the square. If we undershoot or overshoot the extrusion and avoid either side length measurement, then the object will have 3 distinct orthogonal measurements. Nothing more can be done here and as a result we have produced 3 distinct subpecies in R3: a cube, a square prism and a rectangular prism. I see a pattern and a correlation here: in R1 - only 1 possibility; R2 - 2 possibilities; and R3 - 3 possibilities.
I do believe I've discovered a hitherto unknown hole in my knowledge. Or have I nesciently erred somewhere?
- Sobieski
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
-

Sobieski - Mononian
- Posts: 12
- Joined: Sat Oct 12, 2013 9:24 pm
- Location: Ankara, Turkey
Re: Binomials and n-cube cutting
This recent question of yours just refers to the partition problem of number theory. Cf. e.g. http://en.wikipedia.org/wiki/Partition_%28number_theory%29 and the related Young diagrams.
(You might also like to cf. the respective german wiki page, providing not exactly the same view, so this might complement your vision.)
P(n) = number of possibilities to write n as a sum of positive integers
(corresponding to your exponents)
P(1) = 1
P(2) = 2
P(3) = 3
P(4) = 5
P(5) = 7
P(6) = 11
P(7) = 15
P(8) = 22
P(9) = 30
--- rk
(You might also like to cf. the respective german wiki page, providing not exactly the same view, so this might complement your vision.)
P(n) = number of possibilities to write n as a sum of positive integers
(corresponding to your exponents)
P(1) = 1
P(2) = 2
P(3) = 3
P(4) = 5
P(5) = 7
P(6) = 11
P(7) = 15
P(8) = 22
P(9) = 30
--- rk
- Klitzing
- Pentonian
- Posts: 1649
- Joined: Sun Aug 19, 2012 11:16 am
- Location: Heidenheim, Germany
Re: Binomials and n-cube cutting
Oh my goodness! This is so wonderful!!!! 
Thank you for sharing.
I'll get into the Number Theory Young diagrams as soon as I can. I see that a bit of this knowledge will help us to make safe generalizations for higher-dimensional spaces.
But I have another question: between cubic hyperprisms and duoprisms, which is the more generic and which more specific. In other words, is one a superset/subset of the other? I know that the tesseract is a very special totally regular 4-brick and would lie at the end of a taxonomical tree.
More to ponder....![Pleased :]](./images/smilies/pleased.gif)

Thank you for sharing.
I'll get into the Number Theory Young diagrams as soon as I can. I see that a bit of this knowledge will help us to make safe generalizations for higher-dimensional spaces.
But I have another question: between cubic hyperprisms and duoprisms, which is the more generic and which more specific. In other words, is one a superset/subset of the other? I know that the tesseract is a very special totally regular 4-brick and would lie at the end of a taxonomical tree.
More to ponder....
![Pleased :]](./images/smilies/pleased.gif)
- Sobieski
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
-

Sobieski - Mononian
- Posts: 12
- Joined: Sat Oct 12, 2013 9:24 pm
- Location: Ankara, Turkey
Re: Binomials and n-cube cutting
All of the regular solids that exist in five and higher dimensions, are the direct powers of primitive forms.
The five regular solids are: the simplex, the orthotope (square, octahedron, 16choron, &c) gives polytegmids, the circles and spheres, the measure polytopes (squares, cubes, tesseracts), and the cubics. There are a vast collection of 'lace prisms' and 'lace tegums', of my discovery, that are much discussed here.
But in general, there is a thing called the 'surtope equation', which is an algebraic form, whose term (x_n a^n) is a count of x_n separate surtopes of n dimensions. The cube is for example, 6 a^2 + 12 a^1 + 8 a^0. The products are operations P(ab) = kP(a)P(b), where P(x) is a property of x (eg volume in some unit). For the surtope products P(x) is the surtope equation, with various additions of terms at either end: for the prisms and pyramids, one adds a 'content' element, which is 1 a^n (eg where n=solid space). For the pyramids and tegums, one sets k=a, and adds a^{-1}.
So the P(cube) = a^3 + 6a^2 + 12a + 8, = (a+2)^3, being the cube of P(line) since the surface of a line is '2 points'. A cube is a square prism, a square is a line prism, a line is a point prism. But a point is not a prism of anything.
A Tetrahedron, at 4a^2 + 6a + 4, gives T(tetra) = a^3 + T + a^-1, is the biquadrate of (1+1/a) . a^3, is the fourth power of its vertex. A tetrahedron is a triangle pyramid, a triangle is a line pyramid, a pyramid is a point pyramid, a point is a null-pyramid.
The five regular solids are: the simplex, the orthotope (square, octahedron, 16choron, &c) gives polytegmids, the circles and spheres, the measure polytopes (squares, cubes, tesseracts), and the cubics. There are a vast collection of 'lace prisms' and 'lace tegums', of my discovery, that are much discussed here.
But in general, there is a thing called the 'surtope equation', which is an algebraic form, whose term (x_n a^n) is a count of x_n separate surtopes of n dimensions. The cube is for example, 6 a^2 + 12 a^1 + 8 a^0. The products are operations P(ab) = kP(a)P(b), where P(x) is a property of x (eg volume in some unit). For the surtope products P(x) is the surtope equation, with various additions of terms at either end: for the prisms and pyramids, one adds a 'content' element, which is 1 a^n (eg where n=solid space). For the pyramids and tegums, one sets k=a, and adds a^{-1}.
So the P(cube) = a^3 + 6a^2 + 12a + 8, = (a+2)^3, being the cube of P(line) since the surface of a line is '2 points'. A cube is a square prism, a square is a line prism, a line is a point prism. But a point is not a prism of anything.
A Tetrahedron, at 4a^2 + 6a + 4, gives T(tetra) = a^3 + T + a^-1, is the biquadrate of (1+1/a) . a^3, is the fourth power of its vertex. A tetrahedron is a triangle pyramid, a triangle is a line pyramid, a pyramid is a point pyramid, a point is a null-pyramid.
The dream you dream alone is only a dream
the dream we dream together is reality.
\ ( \(\LaTeX\ \) \ ) [no spaces] at https://greasyfork.org/en/users/188714-wendy-krieger
the dream we dream together is reality.
\ ( \(\LaTeX\ \) \ ) [no spaces] at https://greasyfork.org/en/users/188714-wendy-krieger
-

wendy - Pentonian
- Posts: 2031
- Joined: Tue Jan 18, 2005 12:42 pm
- Location: Brisbane, Australia
Re: Binomials and n-cube cutting
Whoah! That last post was a bit abstruse for me at this stage, interesting nonetheless. There are many terms and nomenclatures that I have to assimilate.
I'll need some time to ponder these things. I am, after all, exploring a lot of new territory thanks to all you good people.
BTW, Wendy, how is Brisbane? It myust be warming up there. I used to live in Carindale and when I left Brisbane all those years ago, I was living in the 'Gabba.
I'll need some time to ponder these things. I am, after all, exploring a lot of new territory thanks to all you good people.

BTW, Wendy, how is Brisbane? It myust be warming up there. I used to live in Carindale and when I left Brisbane all those years ago, I was living in the 'Gabba.

- Sobieski
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
-

Sobieski - Mononian
- Posts: 12
- Joined: Sat Oct 12, 2013 9:24 pm
- Location: Ankara, Turkey
Re: Binomials and n-cube cutting
Brisbane is mostly fine. I live in the inner west, and rarely venture south of the river. I was a bit miffed to find that they closed down the railway goods yard at the 'gabba, it was there the last time i ventured over the river.
It's currently moving from the 'dry' seasons into the 'early storms' season. We had a cyclone (Oswald) here last year, it dropped 10 inches of rain at my place, but apart from the odd tree fallen over, and a severely flooded river, nothing much happened. Oswald crossed the cost a few miles south of weipa, and more or less tracked down inside the coastline about 60 miles on-shore, generally causing a nuisance. It petered out at tenterfield.
Apart from that we have had a very long dry spell where the dams fell to 20% of capacity, and there were severe water restrictions. A little later on, the dam was at 200% capacity, and people were annoyed that they let water out at the height of the floods.
Apart from that, life goes on.
It's currently moving from the 'dry' seasons into the 'early storms' season. We had a cyclone (Oswald) here last year, it dropped 10 inches of rain at my place, but apart from the odd tree fallen over, and a severely flooded river, nothing much happened. Oswald crossed the cost a few miles south of weipa, and more or less tracked down inside the coastline about 60 miles on-shore, generally causing a nuisance. It petered out at tenterfield.
Apart from that we have had a very long dry spell where the dams fell to 20% of capacity, and there were severe water restrictions. A little later on, the dam was at 200% capacity, and people were annoyed that they let water out at the height of the floods.
Apart from that, life goes on.
The dream you dream alone is only a dream
the dream we dream together is reality.
\ ( \(\LaTeX\ \) \ ) [no spaces] at https://greasyfork.org/en/users/188714-wendy-krieger
the dream we dream together is reality.
\ ( \(\LaTeX\ \) \ ) [no spaces] at https://greasyfork.org/en/users/188714-wendy-krieger
-

wendy - Pentonian
- Posts: 2031
- Joined: Tue Jan 18, 2005 12:42 pm
- Location: Brisbane, Australia
Re: Binomials and n-cube cutting
Hello again after a little hiatus...
Just thought that anyone following would like to know that I've been able to acquire Stella4D now that my credit card debt has been discharged.. All I can say is WOW!!! What a magnificent piece of software! Three thumbs up (at least)...
This'll add a new dimension (pardon the pun) to my explorations...
Just thought that anyone following would like to know that I've been able to acquire Stella4D now that my credit card debt has been discharged.. All I can say is WOW!!! What a magnificent piece of software! Three thumbs up (at least)...
This'll add a new dimension (pardon the pun) to my explorations...
- Sobieski
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
The message is very simple: THINK FOR YOURSELF and QUESTION AUTHORITY.
-

Sobieski - Mononian
- Posts: 12
- Joined: Sat Oct 12, 2013 9:24 pm
- Location: Ankara, Turkey
22 posts
• Page 1 of 1
Return to Higher Spatial Dimensions
Who is online
Users browsing this forum: No registered users and 52 guests
