Thanks Hugh, QuickFur & AC2000.
quickfur wrote:There are other variants as well. One of my least favorite (I admit I'm biased here) is the so-called Schlegel diagram, where you do a perspective projection to a point not outside the object proper, but on its surface. Of course, you can't really do this in real-life (if your eye is right on the surface you can't really see much), but mathematically you can compute the coordinates of the result. It turns out that when you do this, the nearest face of the object will expand such that its projection image covers the image of the rest of the object. The rest of the object then appears, highly-distorted, inside this nearest face.
Cool, thanks for that QuickFur. I was curious and had a look at Wikipedia for Spatial 4D. I couldn't see that they mentioned the different modelling techniques. That might be useful for someone to add or suggest one day.
quickfur wrote:Aha! I see where this is going, based on what you wrote before in other threads. This is a nice alternative approach to the multi-frames method, except that instead of doing multiple parallel frames, you're doing rotated frames around the vertical. Let's see how you develop this idea.

That's it exactly. I'm hoping that some computer games can be developed using this technique to give us a new expanded 4D experience...
...and I'm thinking a bit about how I would like the story, for the first game, to unfold which I'll write about later...
Just as a slight diversion for the moment I had one of those moments of clarity last night as I was going to sleep. Well, two moments actually.
I'll explain the 2nd moment first and I'll post the 1st moment later today because it requires several diagrams.
The 2nd could use diagrams too but I'll just explain it here.
Both moments had to do with how a 4Der pictures a cube. The 2nd moment brought time into things temporarily (or maybe that should be temporally) to help us understand.
This is probably something that has already been written about but it just occurred to me last night.
Another separate point that occurred to me (maybe we should call it moment of clarity 3

) was that ana and kata need to be separated from viewing with a 4th directional ability.
A 4Der can, for example, view a cube from one of our 3 dimensional directions and still see a cube how they would see it...
The pre-requisite to this is that they need to hold and rotate the cube with them so that they are still not looking at it edge on.
(The result of the 4Der turning it like this - as QuickFur has mentioned - is that we would only be left seeing a square).
So, I'm feeling their needs to be another two terms to indicate whether a 4Der is looking at an object from one direction or it's opposite.
I guess front and back could remain useful. ie. We look at the front of a square and then we turn it around and look at the back.
The same terminology then needs to be expanded when we talk about cubes. We 3Ders can look at the front of a cube, turn it around and look at the back. We can also turn it quarter around and look at it from either side, or turn it up or down to look at the top or bottom. To us these are all equivalent to looking at the cube from its prominent views.
A 4Der, however, instead thinks in terms of looking at a cube's front or turning it around and looking at its back.
For them to look at it any other way, they would conceive as themselves looking at the cube edge on.
The equivalent for us would be to look at a square edge on which is not our usual thing.
The same goes for the 4Der. They will generally only look at a cube front on or turn it around and look at its back. They generally won't look at a cube edge on.
And as I mentioned, when a 4Der turns a cube edge on they see it as we would see a cube; just as we also see a square how a 2Der does when we look at that square edge on.
Now back to the moments...
Moment 2:
To help explain how a 4Der sees things I am replacing the 4th direction with time.
If we do this we can throw ourselves into the picture as well with a little bit of modification to ourselves.
The modification is that we have to be able to see past, present and future all at once.
If you look at a cube over time the cube remains unchanged. It looks exactly the same.
Your retinas also travel through time, like the cube, otherwise you would only see the cube while your retinas existed.
ie. We don't see the cube before we were born and we can't see it after our death.
Now, if instead of travelling through time our retinas were able to connect all those moments of time, and our brain could process all these images as if at one time, then this is how a 4Der would see a cube. If they look at - or we look at all at once through time - a particular square face then they/we would see the same square face connected through time to form a time cube. So basically through this process they are seeing one of the cube faces of a tesseract.
Of course they can do this with any face of the resultant tesseract so that they see six cube faces by turning the tessearact around.
Like we can only see 3 cube faces at at one time they can only see 4 tesseract 'faces' at any one time.
To be a tesseract it has to have all equal sides so at some point of time the tesseract would have to pop into existance and then pop out of existance.
I'm not sure how you would measure centimetres into the timeline but the tesseract would have to be n centimetres long, wide, high and through time.
Our eyes and brain would have to be able to see through time with the left of time and right of time (ana/kata) disappearing off into the perpendicular time horizon.
Now, how about a cube cube; and not a tesseract?
Well, for us to see a cube with a 4Der's eyes the cube would have exist at only one point in time... or does it?
The trick is that this view is the same view a 4Der sees when they are looking at the cube edge on.
If the cube existed for only one moment and our eyes were able to see all moments at once then we would basically be looking at the cube edge on as a 4Der does.
So how does the 4Der - and us with our retinas seeing through time - see a cube front on then?
This is the part that really explains how a 4Der normally looks at our cube front on or via its back.
What we do is take a single square face and put that square face through multiple adjacent time moments for a distance equal to the side of the square.
If our eyes can see all those moments at once then we are effectively looking at a cube front on as a 4Der sees it.
Except that you have to remember that all those time frames are connected to our up-down and left-right views and this forms a cube that we can see in its entirety all at once.
We don't see a series of individual time frames with eyes in each time frame. We see all those time frames at once to form a time cube.
Just as a square is a series of lines but we don't think of them as lines; the 4Der sees a cube from front not as a series of squares but as a cube that they can see all at once.
You have to remember that the first time frame is not the front and the last time frame is not the back; or vice versa.
ie. that equally means the last time frame is not the front and the first time frame is not the back. To the 4Der all these time frames would be equal.
So instead, if our eyes could see across time then the first square face would just be the time-left of the cube and the last square face would just be the time-right of the cube.
Just as for us the first line of a square is just the left of the square and the last line of the square is just the right of the square.




 . I guess I should also include the multi-coloured cube method by CH Hinton provided to us courtesy of AC2000. If I have missed any others please add them for me.
. I guess I should also include the multi-coloured cube method by CH Hinton provided to us courtesy of AC2000. If I have missed any others please add them for me.

 I just read through all your previous steps again, and I like where this is going. Now I'm waiting to find out what's coming next! I'm especially eager to find out what your new method is. Judging from what you've presented so far, it promises to be very interesting indeed.
I just read through all your previous steps again, and I like where this is going. Now I'm waiting to find out what's coming next! I'm especially eager to find out what your new method is. Judging from what you've presented so far, it promises to be very interesting indeed. .
.


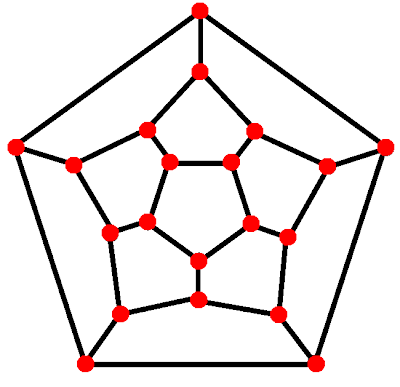
 ) was that ana and kata need to be separated from viewing with a 4th directional ability.
) was that ana and kata need to be separated from viewing with a 4th directional ability.




