Quickfur's renders

[quickfur]

Here's the next 4D Catalan, as promised:



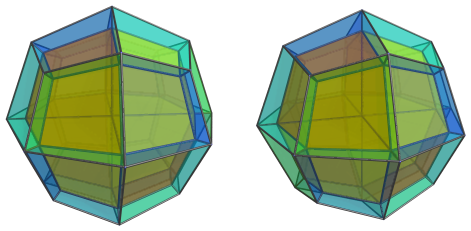
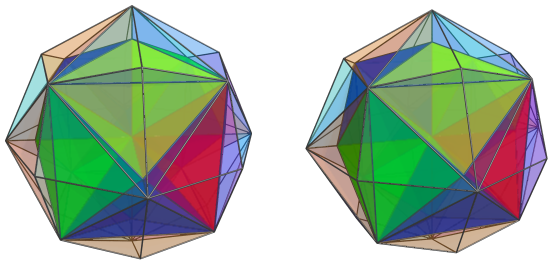
This is what the Polytope Wiki calls the Triangular Antitegmatic Hexacontatetrachoron, the dual of the runcinated tesseract. It's obviously the direct analogue of the deltoidal icositetrahedron, the dual of the rhombicuboctahedron.

Interestingly enough, the projection envelope of this 4D baby is exactly the deltoidal icositetrahedron, having exactly the same proportions. This leads to a conjecture that all duals of x4o...o3x have this projective relationship with each other. I haven't worked out the proof yet, nor have I checked the 5D analogue yet, but I'm fairly confident this is the case. I find this remarkable, because most of the 4D Catalans (that I've checked so far) don't have such direct proportions with their 3D analogues. It seems that x4o...o3x is one of those cases where there's a direct analogy across dimensions -- if this conjecture is true. It's also remarkable that this polychoron has 3 different edge lengths, whereas the deltoidal icositetrahedron has only 2 different edge lengths, yet in the projection the edge length proportions line up nicely. So this projective relationship isn't as trivial as it may seem. In any case, it's an interesting observation.

[wendy]

that's right. with the runcinate xP..Qx, the dual is an antigum along the line between the vertices of xP...Qo and its dual. so the antitegum is based on what is the ...
I use the Conway term strombiate.

[mr_e_man]

Next Catalan?

[quickfur]

Oops, I actually had it ready 2 weeks ago, but got busy and totally forgot to post it on the website. I'll get around to it sometime today, hopefully.

[quickfur]

OK, got it set up faster than expected. Here it is:



This is the square antitegmatic hecatontetracontatetrachoron (what a mouthful!), the dual of the runcinated 24-cell. This time round I actually have two series of structural renders, one the traditional layer-by-layer cell breakdown, and a bonus one showing the Hopf fibration structure of a subset of the cells. Very interesting, check it out!

[quickfur]

This month's POM (polytope of the month) is the dual of the uniform pentagonal prism, the (non-Johnson) pentagonal bipyramid:



Nothing special in itself, but it does feature as a cell in a 4D Catalan which will be posted next month. So stay tuned!

[Klitzing]

That one then ought be the dual of the rectified 600-cell, cf. https://bendwavy.org/klitzing/incmats/o3m3o5o.htm, which also is known as "pentagonal-bipyramidal heptacosiicosachoron".
In fact yourself provided a first render in here already, cf. http://hi.gher.space/forum/viewtopic.php?p=16141#p16141, however the pic itself seems lost since (but then was saved and still is shown on my page, hehe).

--- rk

[quickfur]

Very nice! So you already know what's coming.

[quickfur]

And here it is:



This is the joined 120-cell, sporting 720 pentagonal bipyramids. It is the dual of the rectified 600-cell, one of the rare semiregular polychora.

[quickfur]

Hmm, apparently I neglected to post this month's POM:



This is, of course, the famous rhombic triacontahedron, which is one of the projection images of the castellated rhombicuboctahedral prism, the first CRF we discovered that sported bilunabirotunda cells in a non-trivial manner. The order-5 vertices correspond to the apices of the castellated prism's pentagonal pyramid cells, and the order-3 vertices correspond with the tetrahedral cells.

[Keiji]

Thank you for going to the effort to continue to post these after all these years!

I'm hoping to spend a little more time reading up on these topics again. What would you say were the most interesting discoveries of the last 5 years or so?

[quickfur]

Hey Keiji, good to have you active here again, it's been a while!

Sad to say, I have hardly been keeping up with discoveries lately. Mostly just doing what I can to keep my website updating in a semi-regular fashion (has been better since last year when I made a large enough buffer of Catalan solid entries that I could continue posting monthly in spite of often having no time at all to do anything 4D or 3D related). I'm dreading the day my buffer runs out, then I might have to go on hiatus again.

//

Anyway, since I'm at it, might as well include the latest entry on my website, that I haven't gotten around to posting here until now:



This is the so-called "bidecachoron", the dual of the bitruncated 5-cell. (Not perfectly happy with the name, I got the name from the Polytope Wiki, apparently coined by Bowers. But for lack of a better name, it will have to suffice for now.) Interestingly enough, the projection envelope is a cube, and the layout of cells in it embodies the two ways one could inscribe a tetrahedron in a cube. I'm sure there's a rational reason behind it all, of course, but it's just an interesting coincidence that struck me when I was doing these renders.

[username5243]

Just to provide some context: That name was chosen more out of analogy with a different family than the Catalans - the family of convex vertex-transitive polychora formed from the convex hull of two inversely oriented 5-cell truncates, so you have names like the bitruncatodecachoon rfo the hull of two opposite truncated 5-cells, etc. As you can tell from the coordinates listed on your site, the dual of the bitruncated 5-cell is just the convex hull of the compound of two dual 5-cells.

A full investigation into these (along with the 24-cell family analogs of them) was done by those of us on the Discord/wiki a few years back. Interestingly, for some there are several related forms based on taking the hull of two similar polychora, but with the edge lengths varied - any Wythoffian uniform polychoron with more than one ringed node in the CD has infinite non-uniform (but still isogonal) variations formed by adjusting edge lengths, and in some cases (such as that of the cantellated 5-cell) taking the hull of two of these variants will give results with different types of cells, rather than just similar edge-length variations. There are in fact a total of 24 of these isogonal polychora with doubled 5-cell symmetry and up to 240 vertices, and each has a corresponding one with doubled 24-cell symmetry. The 5-cell ones actually have a page on the Bowers polychoron site now if you are at all interested - he also gets into even stranger chiral varations, because you can do this with variations of the (non-uniform but still isogonal) snub 5-cell as well.

[quickfur]

I've noticed that even among the 3D Catalans, the coordinates are essentially a union of the coordinates of 2 or more of xNo3o, oNx3o, and oNo3x, variously scaled. I.e. the Catalans are the convex hull of various compounds of the regular and quasi-regular polyhedra of their respective families.

Apparently in 4D this is also the case. I surmise it's true in all dimensions.

[username5243]

I've noticed that even among the 3D Catalans, the coordinates are essentially a union of the coordinates of 2 or more of xNo3o, oNx3o, and oNo3x, variously scaled. I.e. the Catalans are the convex hull of various compounds of the regular and quasi-regular polyhedra of their respective families.

Apparently in 4D this is also the case. I surmise it's true in all dimensions.

Correct, at least if the original polytope is Wythoffian. And it is easy to figure out which specific vertex sets are involved, based on the facets of the original. As you know the facets of a Wythoffian uniform polytope can be fonud from the CD by removing one of the nodes and all adjacent edges. However, sometimes this will yield a lower-dimensional element instead - take x4x3x3o, where removing the third node gives x4x o, representing only an octagonal face between two great rhombicuboctahedra. Its dual then does not use the o4o3o3o rectate with that node (that is o4o3x3o) but does use the others.

For non-Wythoffian cases of course things get more complicated, so this will not work for stuff like the snub 24-cell if you get around to its dual.

[quickfur]

Forgot to post this here, this is the latest 4D Catalan:



This is the joined 16-cell, the dual of the rectified tesseract. Interestingly enough, the projection envelope is a regular octahedron.

[quickfur]

Hmm, looks like I forgot to post last month's polytope of the month:



And yesterday I just posted this month's polytope:



(Both images are linked to the respective pages.)

[quickfur]

Looks like again I forgot to post last month's POM:



This is the dual of the rhombicosidodecahedron, as yall probably already know.

//

Meanwhile, this month's POM is already up:



This looks like just the vertex-first projection of the tesseract... but actually, it's the triangular antitegmatic icosachoron, the dual of the runcinated 5-cell. It's analogous to the rhombic dodecahedron (as the dual of the face-expanded tetrahedron aka cuboctahedron), which also projects to a hexagon with 3 rhombus faces that looks like a vertex-first projection of a cube. Both are n-cube lookalikes in this angle, although of course, from other POVs their difference becomes obvious.

[mr_e_man]

What happened (or, what didn't happen) with the 3:1 double-rotating cantellated tesseract? I still would like to see it.

[quickfur]

Sorry, I just couldn't figure out how to orient the rotation properly, so I put it aside for some time and then forgot about it.

Basically, my polytope viewer is currently somewhat limited in how it expresses rotations; it does not accept rotations in matrix form, but only handles axis-aligned rotations. So to get the correct rotation, I need to know how to orient the polytope so that the plane of rotation lines up with one of the cardinal planes. Unfortunately, orienting the polytope is also limited to cardinal rotations (eventually I plan to improve this, but that will require some time to design and implement). So I need to figure out a sequence of cardinal rotations that will bring the polytope into the required orientation so that the desired rotation happens along one of the cardinal planes. I haven't been able to figure this out from your description, sorry.

Alternatively, if you could supply pre-transformed vertex coordinates of the polytope, then I could run the convex hull algorithm to create the model already in the right orientation and do the rotation that way.

[mr_e_man]

Basically, my polytope viewer is currently somewhat limited in how it expresses rotations; it does not accept rotations in matrix form, but only handles axis-aligned rotations.
[...]

Alternatively, if you could supply pre-transformed vertex coordinates of the polytope, then I could run the convex hull algorithm to create the model already in the right orientation and do the rotation that way.

Okay, I took an hour or two to automate the transformation. Here's my Python code.

Code: Select all

class Sqrt2Number:

    def __init__(self, unit_coefficient, sqrt2_coefficient):
        self.coef0 = unit_coefficient
        self.coef1 = sqrt2_coefficient

    def __repr__(self):
        (s0, s1) = (self.coef0, self.coef1)
        if s1 == 0:
            return repr(s0)
        if s0 == 0:
            return repr(s1) + "*q"
        if s1 < 0:
            return repr(s0) + "-" + repr(-s1) + "*q"
        return repr(s0) + "+" + repr(s1) + "*q"

    def __add__(self, value):
        if not isinstance(value, Sqrt2Number):
            value = Sqrt2Number(value, 0)
        return Sqrt2Number(self.coef0 + value.coef0, self.coef1 + value.coef1)

    __radd__ = __add__

    def __pos__(self):
        return self

    def __neg__(self):
        return Sqrt2Number(-self.coef0, -self.coef1)

    def __sub__(self, value):
        return self + -value

    def __rsub__(self, value):
        return -self + value

    def __mul__(self, value):
        if not isinstance(value, Sqrt2Number):
            value = Sqrt2Number(value, 0)
        (s0, s1) = (self.coef0, self.coef1)
        (v0, v1) = (value.coef0, value.coef1)
        return Sqrt2Number(s0*v0 + 2*s1*v1, s0*v1 + s1*v0)

    __rmul__ = __mul__

x = Sqrt2Number(1, 0)
q = Sqrt2Number(0, 1)
w = Sqrt2Number(1, 1)
m = Sqrt2Number(-1, 1)  # Reciprocal of w


Code: Select all

unsigned_vertices = [
    [x, x, w, w],
    [x, w, x, w],
    [x, w, w, x],
    [w, x, x, w],
    [w, x, w, x],
    [w, w, x, x],
]

vertices = []
for v in unsigned_vertices:
    for s0 in [1, -1]:
        for s1 in [1, -1]:
            for s2 in [1, -1]:
                for s3 in [1, -1]:
                    vertices.append(
                        [s0*v[0], s1*v[1], s2*v[2], s3*v[3]]
                    )

matrix = [
    [+x, -w, -x, -w],
    [+x, +w, +x, -w],
    [+w, +x, -w, +x],
    [+w, -x, +w, +x],
]

transformed_vertices = []
for v in vertices:
    transformed_vertices.append(
        [sum(v[i]*matrix[i][j] for i in range(4)) for j in range(4)]
    )

# Everything has a factor of 2.  Divide by 2, and by w.
# (This just changes the overall size of the polytope.)
for v in transformed_vertices:
    for i in range(4):
        vi = v[i]
        (vi0, vi1) = (vi.coef0, vi.coef1)
        assert vi0 % 2 == vi1 % 2 == 0
        v[i] = Sqrt2Number(vi0 // 2, vi1 // 2) * m

for v in transformed_vertices:
    print(v)


The resulting vertices:

Code: Select all

[2*q, 0, 0, 0]
[-1+1*q, 1, -1-1*q, -1]
[-1+1*q, -1, 1+1*q, -1]
[-2, 0, 0, -2]
[1+1*q, -1, 1-1*q, 1]
[0, 0, -2*q, 0]
[0, -2, 2, 0]
[-1-1*q, -1, 1-1*q, -1]
[1+1*q, 1, -1+1*q, 1]
[0, 2, -2, 0]
[0, 0, 2*q, 0]
[-1-1*q, 1, -1+1*q, -1]
[2, 0, 0, 2]
[1-1*q, 1, -1-1*q, 1]
[1-1*q, -1, 1+1*q, 1]
[-2*q, 0, 0, 0]
[1+1*q, -1+1*q, 1, -1]
[0, 1*q, -1*q, -2]
[1*q, 0, 2, -1*q]
[-1, 1, 1-1*q, -1-1*q]
[1*q, -2, 0, 1*q]
[-1, -1, -1-1*q, -1+1*q]
[-1+1*q, -1-1*q, 1, 1]
[-2, -1*q, -1*q, 0]
[2, 1*q, 1*q, 0]
[1-1*q, 1+1*q, -1, -1]
[1, 1, 1+1*q, 1-1*q]
[-1*q, 2, 0, -1*q]
[1, -1, -1+1*q, 1+1*q]
[-1*q, 0, -2, 1*q]
[0, -1*q, 1*q, 2]
[-1-1*q, 1-1*q, -1, 1]
[1+1*q, 1, 1-1*q, -1]
[1*q, 1*q, -1*q, -1*q]
[0, 0, 2, -2]
[-1, -1+1*q, 1, -1-1*q]
[1*q, -1*q, -1*q, 1*q]
[-1+1*q, -1, -1-1*q, 1]
[-1, -1-1*q, 1, -1+1*q]
[-2, -2, 0, 0]
[2, 2, 0, 0]
[1, 1+1*q, -1, 1-1*q]
[1-1*q, 1, 1+1*q, -1]
[-1*q, 1*q, 1*q, -1*q]
[1, 1-1*q, -1, 1+1*q]
[0, 0, -2, 2]
[-1*q, -1*q, 1*q, 1*q]
[-1-1*q, -1, -1+1*q, 1]
[1+1*q, -1, -1+1*q, -1]
[0, 0, -2, -2]
[1*q, -1*q, 1*q, -1*q]
[-1, 1-1*q, -1, -1-1*q]
[2, -2, 0, 0]
[1-1*q, -1, -1-1*q, -1]
[1, -1-1*q, 1, 1-1*q]
[-1*q, -1*q, -1*q, -1*q]
[1*q, 1*q, 1*q, 1*q]
[-1, 1+1*q, -1, -1+1*q]
[-1+1*q, 1, 1+1*q, 1]
[-2, 2, 0, 0]
[1, -1+1*q, 1, 1+1*q]
[-1*q, 1*q, -1*q, 1*q]
[0, 0, 2, 2]
[-1-1*q, 1, 1-1*q, 1]
[1+1*q, 1-1*q, -1, -1]
[1*q, 0, -2, -1*q]
[0, -1*q, 1*q, -2]
[-1, -1, -1+1*q, -1-1*q]
[2, -1*q, -1*q, 0]
[1, -1, -1-1*q, 1-1*q]
[1-1*q, -1-1*q, 1, -1]
[-1*q, -2, 0, -1*q]
[1*q, 2, 0, 1*q]
[-1+1*q, 1+1*q, -1, 1]
[-1, 1, 1+1*q, -1+1*q]
[-2, 1*q, 1*q, 0]
[1, 1, 1-1*q, 1+1*q]
[0, 1*q, -1*q, 2]
[-1*q, 0, 2, 1*q]
[-1-1*q, -1+1*q, 1, 1]
[2, 0, 0, -2]
[1, -1+1*q, -1, -1-1*q]
[1, 1-1*q, 1, -1-1*q]
[0, 0, 0, -2*q]
[1, -1-1*q, -1, -1+1*q]
[0, -2, -2, 0]
[0, -2*q, 0, 0]
[-1, -1-1*q, -1, 1-1*q]
[1, 1+1*q, 1, -1+1*q]
[0, 2*q, 0, 0]
[0, 2, 2, 0]
[-1, 1+1*q, 1, 1-1*q]
[0, 0, 0, 2*q]
[-1, -1+1*q, -1, 1+1*q]
[-1, 1-1*q, 1, 1+1*q]
[-2, 0, 0, 2]


(Of course q = √2.)

Apply the fast rotation to the first two coordinates, and the slow rotation to the last two coordinates.

[quickfur]

Thanks for the pre-transformed coordinates, now I'm finally getting something with 45° symmetry. (I.e., a rotation over 360° produces a 4-fold repetition of the same configuration.)

I'm afraid I don't understand what I'm seeing, though. The initial render showed 4-fold symmetry but I couldn't figure out what's going on. So I decided to color the octahedra to see if that was better. I still don't understand what I'm seeing, though. Maybe you can figure it out:



The yellow cells are on the near side of the polytope, the green cells are on the far side. Not that it helps understand what's going on though.

[mr_e_man]

Maybe, instead of highlighting octahedra, just highlight two opposite sircoes, and the eight vertices (0, 0, ±2√2, 0) and (0, 0, 0, ±2√2) and (0, 0, ±2, ±2).
And I'd rather not see the far side of the polytope.

There seem to be 3 groups of triangular prisms (though it's not evident visually): 8 touching the "axis" formed by those eight vertices, 8 touching the orthogonal "axis", and 16 in between. The group of 8 prisms makes me think of some kind of wire-saw, with each prism being a tooth.

[Hugh]

Gosh those rotations look amazing!

[quickfur]

:)

Maybe, instead of highlighting octahedra, just highlight two opposite sircoes, and the eight vertices (0, 0, ±2√2, 0) and (0, 0, 0, ±2√2) and (0, 0, ±2, ±2).
And I'd rather not see the far side of the polytope.
[...]

The problem is, highlighting only two antipodal sircoes breaks the 4-fold rotation symmetry. So the animation to be several times longer before the cells return to their original positions. In fact, I've tried up to 4 times as long and still haven't found the cells returning to the original positions yet. It may turn out to be something as large as 12 times as long.

If you look at the above animation and trace a single octahedron, you'll see that it makes a pretty long spiralling orbit around the polytope before returning to its original position.

Also, clipping the far side of the polytope may make it even more confusing, since you'll have cells popping in and out when they move across the limb of the projection. This is why I chose to color the far side cells in green instead of clipping them completely. But since you asked:

[mr_e_man]

In fact, I've tried up to 4 times as long and still haven't found the cells returning to the original positions yet. It may turn out to be something as large as 12 times as long.

If you look at the above animation and trace a single octahedron, you'll see that it makes a pretty long spiralling orbit around the polytope before returning to its original position.

The symmetry is (135°, 45°), so a cell returns to its starting position after 8 cycles of the animation. But if antipodal cells look the same, it should be only 4 times as long.

Whether you highlight some or all sircoes, or some or all trips, I think it would help you (or anyone) figure out what's going on. The octahedra are the least helpful, because they don't touch the axes of rotation (except at vertices).

About highlighting vertices, I had in mind your 600-cell images.

Also, clipping the far side of the polytope [...]

I notice some triangles are still appearing green at the limb, then turning yellow as the whole octahedron appears. Is that supposed to happen?

[quickfur]

[...]
The symmetry is (135°, 45°), so a cell returns to its starting position after 8 cycles of the animation. But if antipodal cells look the same, it should be only 4 times as long.

I tried running the animation for 4x its length, there's still a skip between the end and the start. I've no idea why. I'll have to play around with it some more to figure out what's going on.

[...]

Also, clipping the far side of the polytope [...]

I notice some triangles are still appearing green at the limb, then turning yellow as the whole octahedron appears. Is that supposed to happen?

This is because the while the octahedron is on the far side of the polytope, one face still lies on the limb, so that still shows up. I could get rid of them, of course, but then you'll just have octahedra popping in and out of view suddenly as the rotation happens.

[mr_e_man]

[...]
The symmetry is (135°, 45°), so a cell returns to its starting position after 8 cycles of the animation. But if antipodal cells look the same, it should be only 4 times as long.

I tried running the animation for 4x its length, there's still a skip between the end and the start. I've no idea why.

That is strange....

[...]

Also, clipping the far side of the polytope [...]

I notice some triangles are still appearing green at the limb, then turning yellow as the whole octahedron appears. Is that supposed to happen?

This is because the while the octahedron is on the far side of the polytope, one face still lies on the limb, so that still shows up.

But what does it mean for a face to be on the limb?

A choron (i.e. 3D cell) is on the limb when its normal vector is perpendicular to the line of sight in 4D. It's on the far side of the polytope when the outward normal vector has a positive component in the direction of the line of sight, i.e. a positive dot product (or negative, depending on whether the line goes from the viewer or to the viewer).
A hedron (i.e. 2D face) doesn't have a unique normal vector. How do you determine whether it's on the near side or the far side?

Well, I suppose the hedron should be visible when any one of the incident chora is visible.

[quickfur]

A hedrix is considered to be on the limb when either (1) one of its incident chora is on the far side, and the other incident chora is on the near side; or (2) one or more of its incident chora lies on the limb (i.e., the normal vector is perpendicular to the line-of-sight).

[mr_e_man]

Right.

And how do you determine the colour of a hedron?
Is it specified independently?
Or is it a "sum" of colours of the two incident chora? If one choron is invisible, on the far side, then it shouldn't contribute its colour to the hedron.

From your rectified 24-cell images (very pretty ), it looks like you're using just one of the colours, not combining them. Specifically, if both chora are visible, then the hedron gets the colour of the one farther from the 3D viewpoint. I think I used this method for some of my images.