On the nature of Infinity

[adam ∞]

How are we to understand what is meant by "infinity"?

For one person, "infinity" means a never ending process, for another, it means the result of a terminating process. Some consider infinity to be an actual number, for others, it is merely a "concept". For some, infinity is considered by its very nature to be paradoxical, having properties that would be considered impossible for ordinary numbers. For others, it is believed that infinity should behave according to the same types of rules as finite numbers.

Can infinity ever end? Can there be a last finite number? Can there be an infinite amount of finite whole numbers? Is there only one "infinity" or can there be infinitely many infinities, infinitely larger than the previous one? Is there some kind of transition between the finite and the infinite? If so, what is the nature of this transition?


This is a continuation of a discussion started in this post:
http://hi.gher.space/forum/viewtopic.php?f=27&t=2568


I find it necessary to distinguish between the type of infinity intended in a meaningful discussion about infinity. This is always difficult, since people have very different views about infinity and the meaning of terms associated with it. Nonetheless, I will try to start out with the following:

potential infinity - (also known as "improper infinity")

A distinction that goes back to Aristotle, a potential infinity is never ending.


actual infinity - (or "proper" infinity)

Infinity as a limit, the result of a completed sequence.


absolute infinity

Cantor's idea of an infinity so large that nothing can be larger. The highest number or amount possible, nothing can be added to it since it contains all numbers. Usually regarded as less mathematical and more philosophical than other types of infinity.


From these, we can also distinguish between two types of potential infinity:


Finite potential infinity - a process that is considered never ending, but always remains finite


Infinite potential infinity - a process that is considered never ending, but is not finite


Some people believe that, by definition, you can never add 1 to a finite number to get a number that is not finite. That a sequence starting with 1, and adding 1, and continuing to add 1 to each successor, can never reach infinity. Or similarly, that no mathematical operation involving a finite number, applied to another finite number, can ever result in something other than a finite number.

From this notion comes a discontinuity between the finite and the infinite. If this is true, and if there is at least one number that is infinite, there seems to be a gap between the finite and infinite with no defined transition from one to the other.

I know of no accepted terminology for these concepts, but for the sake of discussion, let's refer to these concepts by the following terms:

non-successive infinity - an infinity that cannot be reached by the process of starting with 1, adding 1, and adding one the the successor, and so on.


successive infinity - an infinity that can be reached by the process of starting with 1, adding 1, and adding one the the successor, and so on.

For a successive infinity, it can be helpful to distinguish between two possible types:

Adjacently successive infinity - an infinity such that some finite number, plus 1, can equal an infinite number

non-adjactently successive infinity - an infinity such that a series of 1+1+1+1 followed by a successor, and so on, can eventually reach infinity, but the number before infinity is not finite. (As far as I can tell, in order for this to be true, there must be a type of number which is inbetween the finite and infinite)

a quasi-infinite number - a type of number in between finite and infinite.

To me it seems apparent that a non-successive infinity would necessary have a discontinuity between the finite and the infinite with nothing defined in between, but in case it is possible to have a non-successive infinity without a discontinuity between the finite and the infinite with nothing defined in between, let's call an infinity without continuity between the finite and infinite with nothing in between transitionally disconnected. If there is continuity, let's call it transitionally connected.

another possibility is that the distinction between finite and infinite can be thought of more like a gradient scale, with larger numbers being increasingly more infinite until the first fully infinite number is reached. So for example, the number infinity divided by 2 might be considered to be 50% infinite. I don't know if anyone thinks infinity works this way, but the concept could be referred to as "gradient infinity".

non-arithmetic infinity - an infinity that cannot be reached by using mathematical operations such as addition, multiplication, exponentiation, etc, a finite amount of times to a finite number.


arithmetic infinity - an infinity that can be reached by using mathematical operations such as addition, multiplication, exponentiation, etc, a finite amount of times to a finite number.

Some people believe that an actual infinity plus one should equal infinity. Or that two times infinity is the same as infinity. I don't know of a term for this, so let's use the term distinct infinity when infinity plus one or 2 x infinity are distinct from infinity, and indistinct infinity when that is not the case.

We can also use the term numerical infinity for when someone is talking about a number that is considered a number, and conceptual infinity for a concept that is not considered to be a number.

----

It is interesting to look at different number systems and how they deal with infinity, for example the transfinite numbers of set theory, the supernatural numbers, hyperreals, surreal numbers, the extended reals, the projectively extended reals, the p-adic numbers, etc.

Using the terms I have laid out here, it is my interpretation that the I in the axiom of infinity in set theory is claimed to be a non-successive actual infinity, which is also claimed to be connected in some way to N, which is a non-successive finite potential infinity treated in some instances as a non-successive finite potential infinity, in some cases as a non-successive infinite potential infinity, in some cases as a non-successive actual infinity, and depending on what source is used or which set theory expert is asked, a successive actual infinity. The "I" defined in the axiom of infinity is not exactly the same as omega or Aleph0, but there is equivocation between the three concepts as if they are the same in some instances, and as if they are different in other instances.

I have a hard time seeing how the amount of finite whole numbers could ever meaningfully said to be an actually infinite amount. As far as I can tell, the amount of finite numbers, by definition, has to be either a finite potentially infinite amount (= finite, not actually infinite) or a finite amount with a last finite number. To claim that there are an actually infinite amount of finite whole numbers is a contradiction.

---

I think of infinity in this way: Infinity is the first infinite number. All infinite numbers are infinite, but "infinity" as a number refers to the first infinite number.

Infinity is a numerical infinity, actually infinite, distinct, transitionally connected, arithmetic and non-adjacently successive with quasi-infinite numbers between finite and infinite numbers. The infinite numbers after infinity are higher-dimensional numbers, maxing out at dimension infinity, with ∞^∞ power being absolute.

Infinity is not merely conceptual, is not potentially infinite, is not "never ending", and is not the same as infinity minus 1.

Based on the ∞-gon, infinity is "modular" in that following the right side of an infinitely long line will lead to the left side and back to where you started. This is true for all infinite lines. For this reason, infinity is to the left of one on the number line. From this, I believe that space and time have this modular infinity quality.

If you are restricted to the number line, infinity plus one is either not possible, or will bring you back to one, depending on the context. For example, as far as I can tell, there does not appear to be a meaningful way to have a regular ordinary ∞+1 sided polygon in the plane (excluding for example, skew polygons or concave polygons). Without the constraint of remaining on the number line, infinity plus one will be outside of the number line on a higher dimensional number plane. So, for example, you can fit ∞+1 squares in a 2-dimensional space.

I also believe that, from these principles, specific digits for infinity, infinity plus 1, the square root of infinity, etc, can be calculated, infinite numbers have prime factorization, can be odd or even, and can be composite or prime numbers. Infinity plus one, for example, might be prime.

I can explain this in more detail after this, but in my exploration of all of this, I have come to the conclusion that the last few digits of infinity are ...432948736.

[adam ∞]

For the sake of distinguishing between the transfinite numbers, the surreal numbers, the hyperreals, etc, I call my number system the "Ultranatural numbers". In the Ultranatural number system, infinite numbers can be represented with a sequence of digits, either in base 10 and in binary. I don't remember off the top of my head how to translate between the approximate number of digits in binary, and the number of digits in base 10, but the number of digits of infinity is not an infinite amount. The first number with an infinite amount of digits is in the realm of 2^∞, depending on whether you use base 10 or binary. The number of binary digits of infinity is just log ∞, where "log" is the binary logarithm.

If you play around with the digits, you will see that although ∞, log ∞ and 2^∞ all have a vastly different number of digits, they have the same last digits ...432948736. I keep track of # of digits using # of binary digits, I am not sure if there is a way to calculate the exact number of digits in base 10.


2^∞ is ...432948736 with ∞ digits

∞ is ...432948736, with log ∞ digits.

log ∞ is ...432948736, log log ∞ digits. Etc.

The last log ∞ digits of ∞^2 are the same as infinity. The last log log ∞ digits of ∞ are the same as log ∞, and so on.

The same is not true for something like ∞^2 or √∞, which have a different set of digits, which off the top of my head end in ...96 and ..56 respectively.

In the Ultranatural number system, √∞ is finite, and log ∞ is way less than √∞, therefore the number of binary digits of infinity, although an unimaginably huge number, is finite.

I know that to some people, this seems like a contradiction, but I don't see any way around it! If you think about what square roots and logarithms are, and the proportions involved, it is not as counterintuitive as it first seems. The square root of infinity has be a number so big that it can square to infinity, but small enough that it isn't so close to infinity that it's not squarable to infinity, etc. In a way, it is like the "biggest small number", and in another way, the "smallest big number". For a long time I assumed that the number of digits of infinity couldn't be finite, and I denied it, even though denying this will always lead to a contradiction in my calculations. The only other possibility I can see for a representation of infinity with numerical digits that is not finite or quasi-infinite would be to have no continuity between the finite and infinite, which to me is completely meaningless for a number system that includes infinity, and to have the number of digits and the number of digits behave in a way that is totally different from other numbers, where for example, a very large number can be equal to it's number of digits, which would imply in binary that some number q = 2^q, which can't even be true in Cantor's system.

Demonstrating which preference should be accepted might be never be proven to be more than just a matter of philosophy, supported by relatively convincing evidence- considered to be convincing or not relative to your philosophical view of it.


In the Ultranatural number system, that is how it works.

[adam ∞]

For anyone interested, here is a look at the reasoning behind the Ultranatural number system, and how I came to the conclusion that the digits of infinity are what I think they are.

My quest for the digits of infinity started out with trying to figure out if infinity is an odd or even number.

H. S. M. Coxeter defined the ∞-sided polygon {∞} or "apeirogon" in his foundational book Regular Polytopes (1948), as being the limiting case of regular polygons, where the edges meet together at a 180° angle.

We have a sequence of regular polygons, the more sides you add, the more it looks like a circle, with the limiting case having edges meeting at 180°. The page has been changed, but you used to be able go to Wikipedia's Apeirogon page, and see a picture of an apeirogon as a perfectly flat, straight line, broken into line segments, and another zoomed out or embedded view represented as a circle. The type of infinity I am investigating here is based on this idea of the apeirogon, as being in one sense a flat, infinitely straight line broken into segments, and in another sense, at the same time, a zoomed out version of a what looks like a perfect circle.

I noticed that if you put regular polygons in order, with one vertex at the top, even sided polygons will have a single vertex the top and at the bottom, while odd sided polygons will a vertex at the top and an edge at the bottom.

If an apeirogon has mirror symmetry from top to bottom like a circle, it should have a vertex at the top and at the bottom. If there was a vertex at the top, and an edge at the bottom, this would be asymmetrical.

Thus, infinity is even.

Now that we know infinity is even, we can start to investigate what the digits of infinity must be:

All even numbers have to end in 0,2,4,6, or 8. So if there are digits of infinity, infinity should end in 0,2,4,6, or 8.

Since an apeirogon should have the symmetry of a circle, we should expect to find mirror symmetry not just from top to bottom, but also from left to right. This tells us that infinity should be a multiple of 4. We should expect to find vertices in between, as well, which gives us a multiple of 8, and in between those, which gives us a multiple of 16, and so on, like the series 2x2x2x2... and so on. With sides of polygons, this will eventually terminate when the edges meet together at 180°.

From this we are starting to get the picture that infinity must be a power of 2.

It turns out that the last digits of powers of 2 follow a particular pattern:

2,4,8,6,2,4,8,6...

So now we have figured out that the last digit of infinity should be a 2,4,8, or a 6, and not a 0.

So far we know that infinity has the multiples 2,4,8,16, etc, is even, is a power of 2, and is not a multiple of 10 or 5 (since it does not have 0 as a last digit). We also know at this point that infinity cannot be a prime number.

OK, so, how could we ever figure out which of those four digits the last digit of infinity is?

One clue is that, since the apeirogon is modular, where if you follow the edges on the right side, you will continue on until you get to the left side and back to where you started, that perhaps the sequence of last digits of powers of 2 : 2,4,8,6,2,4,8,6.. etc keeps going until the last one is 6. Then if you continued after infinity back to side 1, the next power of 2 would be 2 again.

So we have 6 as a possible last digit, but let's not be so sure just yet.

We have the sequence 2x2x2x2x2.. which grows rather slowly. An exponential sequence will get us there much faster.

2, 2^2 = 4, 4^2= 16, 16^2= 256, 256^2=65536, 65536^2=4294967296, and so on.

We are starting to see 6's and only 6's as the last digit.

A sequence that will give us the same results even faster is:

2, 2^2, 2^2^2, 2^2^2^2, 2^2^2^2.. etc.

But how would we know when to stop?

Keeping in mind that, by convention, 2^2^2^2... means 2^(2^(2^2)... and not ((2^2)^2)^2..
(the first four terms of the first sequence = 65536, while the other equals 256)
Let's take a look at this sequence

1) 2 = 2
2) 2^2 = 4
3) 2^2^2 = 2^4 = 16
4) 2^2^2^2= 2^16 = 65536

the 5th number in this sequence already has over 19,000 digits!
The first few digits are 2003529... and the last are ... 19156736.

5) 2003529...19156736 (19,729 digits)

At this point, I can only keep track of the last few digits. The 6th number has more than 10^19,729 digits

6) ...7437428736

You may have noticed a pattern at this point:

after the 3rd term, the numbers always end in 6.

So, the last digit of infinity is 6.

You may have noticed something else:

16
65536
...156736
...7428736

The last digits are converging on some kind of pattern:

...6
...36
...736
...8736

etc.


7 ) ...9621748736
8 ) ...9960948736
9 ) ...7112948736
10)...4232948736
11)...1432948736
12)...3432948736

at this point, the calculator I am using maxes out at giving the last ten digits.

But so far, we have the last ten digits of infinity:

...3432948736


if we look up the sequence 6,36,736,8736,48736, etc we will find:

https://oeis.org/A206636

n a(n)
1 6
2 36
3 736
4 8736
5 48736
6 948736
7 2948736
8 32948736
9 432948736
10 3432948736
11 53432948736
12 353432948736
13 5353432948736
14 75353432948736
15 75353432948736
16 5075353432948736
17 15075353432948736
18 615075353432948736
19 8615075353432948736
20 98615075353432948736
21 98615075353432948736
22 8098615075353432948736

On this same page, there is a list of 1,000 digits

https://oeis.org/A206636/b206636.txt

So there we have the last 1,000 digits of infinity.

--

I am unsure if we can ever, even in principle, know what the first digits are, because the number is so big. This seems to be true even for huge finite numbers like the 12th number in the sequence 2^2^2^2^2^2^2... But I may have overlooked a more obvious way of possibly figuring them out. If anyone has any ideas, let me know.



Now we can look at ∞-1. ∞+1, etc

∞-1=...432948735
∞ = ...432948736
∞+1= ...432948737

we can deduce from this that ∞-1 has 3, 5, and 17 as factors
I have not been able to find any factors for ∞+1, it is possible that ∞+1 is the first prime infinite number.

For some of these we can figure out a full prime factorization, for others, we can probably only ever figure out a partial prime factorization or a full factorization without knowing for sure which are prime and which are composite.

The factorization of ∞-1 follows a very regular pattern, and can be completely factorized, but I am unsure whether determining if a factor is prime or not in every single case is possible. But, for example, ∞-1 is:

3 x 5 x 17 x 65537 x .... x (√∞)+1

following the pattern

(2^1)+1 x (2^2)+1 x (2^4)+1... etc
but it gets to point where (2^n)+1 is not a prime number.


We can deduce that ∞-6 is has 10 as a factor: ...432948730

Further investigation shows that ∞+2 has 4,6,9 and 11 as factors
∞-2 has 7 and 14 as factors
∞-3 has 13 as a factor
∞-4 has 12 as a factor, and so on.

A good candidate for the largest prime less than ∞ is ∞-15
and nearby, ∞-17

in between, ∞-16 is very composite, having a huge amount of factors: 2,3,4,5,6,7,8,9,10, [not 11], 12, 13.. and so on.

So far, I have concluded that the following numbers might be prime:

∞-15, ∞-17, ∞-39, ∞+1, ∞+3, ∞+7, ∞+15
(∞-14)/2
(∞-13)/3, (∞-3)/13
(∞-12)/4
(∞-11)/5, (∞-5)/11
one of my favorites:
(∞-8)/8
also:
(∞-6)/10 = ...432948730/10 = ...43294873
(∞-26)/10 ...432948731


2∞, 4∞, ∞/2, ∞/4, √∞, log ∞, ∞^2, 2^∞ can all be investigated.

1+2+3+4...+∞-1 can be calculated
1+2+3+4...+∞ can be calculated
(I have these written out somewhere if someone wants to know the answer I got. Or try for yourself and see what you come up with based on those digits)

∞^∞ can be calculated.

And so on.

[mr_e_man]

If an apeirogon has mirror symmetry from top to bottom like a circle, it should have a vertex at the top and at the bottom. If there was a vertex at the top, and an edge at the bottom, this would be asymmetrical.

Thus, infinity is even.

Now that we know infinity is even, we can start to investigate what the digits of infinity must be:

All even numbers have to end in 0,2,4,6, or 8. So if there are digits of infinity, infinity should end in 0,2,4,6, or 8.

Since an apeirogon should have the symmetry of a circle, we should expect to find mirror symmetry not just from top to bottom, but also from left to right. This tells us that infinity should be a multiple of 4. We should expect to find vertices in between, as well, which gives us a multiple of 8, and in between those, which gives us a multiple of 16, and so on, like the series 2x2x2x2... and so on. With sides of polygons, this will eventually terminate when the edges meet together at 180°.

From this we are starting to get the picture that infinity must be a power of 2.

But a circle also has mirror symmetry over a line at 60°; it has the symmetry of a triangle. Therefore infinity must be a multiple of 3.

And a circle also has the symmetry of a pentagon, so infinity must be a multiple of 5. And so on.

[quickfur]

If an apeirogon has mirror symmetry from top to bottom like a circle, it should have a vertex at the top and at the bottom. If there was a vertex at the top, and an edge at the bottom, this would be asymmetrical.

Thus, infinity is even.

Now that we know infinity is even, we can start to investigate what the digits of infinity must be:

All even numbers have to end in 0,2,4,6, or 8. So if there are digits of infinity, infinity should end in 0,2,4,6, or 8.

Since an apeirogon should have the symmetry of a circle, we should expect to find mirror symmetry not just from top to bottom, but also from left to right. This tells us that infinity should be a multiple of 4. We should expect to find vertices in between, as well, which gives us a multiple of 8, and in between those, which gives us a multiple of 16, and so on, like the series 2x2x2x2... and so on. With sides of polygons, this will eventually terminate when the edges meet together at 180°.

From this we are starting to get the picture that infinity must be a power of 2.

But a circle also has mirror symmetry over a line at 60°; it has the symmetry of a triangle. Therefore infinity must be a multiple of 3.

And a circle also has the symmetry of a pentagon, so infinity must be a multiple of 5. And so on.

Yes, which means that infinity must be divisible by every finite number.

Which means that ...7437428736 cannot be the last digits of infinity, because none of the numbers in the sequence 2, 2^2, 2^2^2, ... are divisible by 3, 5, 7, 11, 13, ... etc..

[adam ∞]

Which means that ...7437428736 cannot be the last digits of infinity, because none of the numbers in the sequence 2, 2^2, 2^2^2, ... are divisible by 3, 5, 7, 11, 13, ... etc..

Assuming that infinity must be divisible by 3, 5, 7, 11, and 13, etc, is a false assumption. It is not possible for a number to have all properties of all numbers all at the same time, including contradictory ones.

If infinity was a multiple of 11, it would be far less symmetrical than what I have defined.

If you are claiming that infinity really is just all consecutive numbers up to some point multiplied together, which I don't think you actually believe by the way, it would result in an infinity sided polygon that lacks a lot of the symmetries attributed to circles. They can't both be right at the same time.

Also, this would contradict your earlier claim about not being able to reach infinity by modifying finite numbers.

You also said that infinity minus 1 stays the same as infinity in your view, so that's a contradiction if ..00000000 are the last digits, but ..00000 -1 is not ...999999.


Just because it is infinity doesn't mean that the law of non-contradiction goes out the window.


Even so, there is something known as a "benchmark" that is sometimes used for extending calculations to infinity to a well defined infinite sequence. If your "benchmark" for infinity is 1x2x3x4x5x6x7x8x9x10x11... up to some number (which one?), and you continued with trying to define digits and prime factorizations, you could probably construct a mostly coherent theory based on that, where, for example, infinity -1 would end in ...99999, infinity plus 1 might be prime, etc. Essentially you would be saying, if infinity is this number, then these properties should follow. Without an agreed upon benchmark, that might be as close to a consensus as people are going to get on something like this.

I have explored infinite numbers of this type before, but it is difficult to find patterns for something so irregular.

I don't think factorial numbers are anywhere near as regular as powers of 2, so there is a lot of stuff that you probably wouldn't be able to figure out that would be calculable for powers of two. It would be interesting if you could figure out though, for example, what the digits would be in binary. Or, if p!= infinity, what is p?

The square root of infinity wouldn't be a whole number, neither would log infinity. It would be very irregular.

it would be interesting to see how you would distinguish 1x2x3x4x5x6x7x8x9x10x11... and (1x2x3x4x5x6x7x8x9x10x11...)-1 apart from 10^10^10^10... and (10^10^10^10)-1, since both would end in ...00000000000000 and ...999999999999999999

Figuring out the numbers of digits might be really hard too!

If you did this, your version of infinity still wouldn't have all of the symmetry attributed to circles, so applying that objection to my theory is not unique.

If you really do believe this, I encourage you to explore it! If you share your results, it will help me figure out stuff about my theory that I have not been able to figure out.

If anyone explored different potential benchmarks, defining a consistent system of digits and factorizations, it is contributing something to the theory that has probably never been explored. I have never found another person who believes there are digits of infinity and tried to figure out what they are. It's possible there is a way to convert between different benchmarks, so what is figured out in one version might spill over into another.

Anyways, I have been working on this for decades. It's easy to shoot things down with one sentence and move on. It's a lot harder to actually construct a consistent theory like this.

If infinity is 1x2x3x4x5x6x7x8x9x10x11..., what do you get when you add 1+2+3+4... up to infinity-1? or up to infinity?

[adam ∞]

Which means that ...7437428736 cannot be the last digits of infinity

The last ten digits of infinity in the Ultranatural numbers are ...3432948736, not ...7437428736. The digits ...7437428736 are from the very large finite number 2^2^2^2^2^2, with six 2's.

The other day I finally figured out how many 2's there are for the power tower 2^2^2^2... = infinity. If anybody expresses interest in any of this, I am glad to go into more detail about it. It took a really long time to write that original post, though!


If anyone is planning on seriously investigating the digits and factorizations of 1x2x3x4x5x6x7x8x9.. = infinity, let me know, I may have some useful notes from what I have already explored in this area.

[quickfur]

Which means that ...7437428736 cannot be the last digits of infinity, because none of the numbers in the sequence 2, 2^2, 2^2^2, ... are divisible by 3, 5, 7, 11, 13, ... etc..

Assuming that infinity must be divisible by 3, 5, 7, 11, and 13, etc, is a false assumption. It is not possible for a number to have all properties of all numbers all at the same time, including contradictory ones.

If infinity was a multiple of 11, it would be far less symmetrical than what I have defined.

If you are claiming that infinity really is just all consecutive numbers up to some point multiplied together, which I don't think you actually believe by the way, it would result in an infinity sided polygon that lacks a lot of the symmetries attributed to circles. They can't both be right at the same time.

I arrived at my conclusion simply by following the logic you presented. I did not invent anything new. What the symmetries of an apeirogon? If we consider an apeirogon to be the limit of n-gons as n grows without bound, then it would approach a perfect circle. Certainly, a circle would have mirror symmetry. According to your logic, as you described yourself, this means infinity must be an even number. But a circle has more than just bilateral symmetry. It also has triangular symmetry (among many others). This is self-evident: rotating a circle by 60° yields the exact same circle, unchanged. So if we accept your postulated apeirogon-circle equivalence, that means infinity must also be divisible by 3, which means it must be odd.

So based on these two simple observations, which are entirely based on the train of thought you yourself presented, we have to conclude that infinity is both odd and even.

Also, an apeirogon having 11-fold symmetry does not make it less symmetrical; in fact, it makes it more symmetrical, because it means I can rotate the apeirogon by 16.36...° degrees and it remains unchanged. If your apeirogon did not remain the same after rotation by 16.36...°, that means it would be something different from a perfect circle, since it lacks some of the symmetries that a perfect circle has. An apeirogon that does not have 11-fold symmetry is less symmetrical than one that does.

Also, this would contradict your earlier claim about not being able to reach infinity by modifying finite numbers.

I made the statement about 11-fold (among others) symmetry not because I actually think that infinity is divisible by 11; I made that statement to show you that your proposed system of infinity is inconsistent. You started out by making an equivalence between an apeirogon and a circle, and then proceeded to derive the properties of infinity based on the bilateral symmetry of the circle. However, you failed to consider the other symmetries of the circle, namely, 3-fold symmetry, 5-fold symmetry, and so on. If you had considered these other cases, you would have arrived at different conclusions, conclusions that contradict the results you obtained earlier. Taken together, this means that something went wrong in your line of reasoning that led your to your current conception of infinity. Your system appears to have the semblance of a consistent system when you only consider limited parts of it, but when examined closer, one discovers contradictions hidden therein.

Just because it is infinity doesn't mean that the law of non-contradiction goes out the window.

Precisely. So how do you explain why a circle has 3-fold symmetry (and 2-fold symmetry at the same time) but your apeirogon apparently doesn't?

Even so, there is something known as a "benchmark" that is sometimes used for extending calculations to infinity to a well defined infinite sequence. If your "benchmark" for infinity is 1x2x3x4x5x6x7x8x9x10x11... up to some number (which one?), and you continued with trying to define digits and prime factorizations, you could probably construct a mostly coherent theory based on that, where, for example, infinity -1 would end in ...99999, infinity plus 1 might be prime, etc. Essentially you would be saying, if infinity is this number, then these properties should follow. Without an agreed upon benchmark, that might be as close to a consensus as people are going to get on something like this.

I have explored infinite numbers of this type before, but it is difficult to find patterns for something so irregular.

Has it ever occurred to you that perhaps, just perhaps, the reason for your difficulty is that your desired properties of infinity might be untrue?

I don't think factorial numbers are anywhere near as regular as powers of 2, so there is a lot of stuff that you probably wouldn't be able to figure out that would be calculable for powers of two.

But why limit yourself to powers of 2? You could start with 3-fold symmetry, for example, and construct a sequence of the form 3, 3^3, 3^3^3, 3^3^3^3, ... etc.. If you follow that line of reasoning, you would eventually arrive at the conclusion that the last digits of (3-fold) infinity ought to be ...464195387. So either we have a contradiction, or there must be multiple, different infinities. But if there were multiple different infinities, then which infinity is the one that the apeirogon has? Since the apeirogon has both 2-fold and 3-fold symmetry. (It also has 5-fold symmetry, if we continue to maintain the equivalence between an apeirogon and a circle. And many others.) Surely the number of vertices in an apeirogon cannot be multiple, different numbers at the same time, so this is also a contradiction.

Since we can't escape from a contradiction with this line of reasoning, we're forced to step back and reconsider the idea of apeirogon = circle. This equality cannot hold, because if it did, we inescapably run into the contradictions described above (and many others, if you only look a bit closer). So we're forced to conclude that the apeirogon is not a circle.

Which in turn means we cannot draw conclusions about the nature of infinity in an apeirogon by extrapolating from the properties of a circle, because the two are not the same thing. So what's true in a circle does not necessarily hold in an apeirogon, and conclusions drawn by examining the properties of a circle cannot be carried over to an apeirogon without further proof.

[...]
Anyways, I have been working on this for decades. It's easy to shoot things down with one sentence and move on. It's a lot harder to actually construct a consistent theory like this.

It is hard to come up with a consistent theory. Cantor's theory of infinity, like it or hate it (and yes, there are things about it I also hate), is one of the systems that have survived the test of time (so far, anyway). It is definitely not the first theory of infinity invented; I think there must be at least as many theories of infinity as there are mathematicians, but so far none of the others have withstood scrutiny. I'm also pretty sure you are not the first one who tried to come up with a system of infinity based on apeirogons and circles, but I have not heard of any such system that withstood scrutiny by an international community of mathematicians. Hate what you may about Cantor's system of infinity, but so far it has proven to be a consistent (if weird) system. One could apply you said above to Cantor's system too: it's easy to criticize it for its weird properties, but it's at least consistent within itself (yes, it is self-consistent, it just has properties that defy our intuition). It's a lot harder to come up with a different system of infinity that's consistent and stands up to scrutiny.

When trying to come up with a self-consistent system of infinity, one must hold oneself to higher standards of scrutiny. It's easy to let loose and indulge in computations of digits and properties and stuff, but one ought not to forget that the very act of computing digits, for example, is making an implicit assumption that there are digits to be computed in the first place. Until this is proven and established, it's on shaky ground, and may turn out to be based on false premises after all. Similarly, computing logs and stuff makes the assumption that there is a log to be computed at all (for example, negative numbers do not have logs unless you're using complex numbers). Until the existence of a log is proven, any such computation is on shaky ground, and should be regarded with caution. As elementary logic tells us, starting from false premises one can prove anything, so the fact that something can be computed does not necessarily mean the answer is actually meaningful. One must first be assured that the premises are valid to begin with, otherwise any conclusions drawn from them would have no meaning.

[quickfur]

And by the way, the properties of your proposed system of infinity are easily satisfied by a finite system of arithmetic where there's a specially-designated large finite number. I mentioned Graham's number in one of my replies to you, but I don't know if you picked up on my intended implications. Basically, given a sufficiently-large finite number G (and by "sufficiently-large" I mean extremely large, such that it cannot be arrived at using conventional arithmetic with everyday numbers), in arithmetic involving G and small, everyday numbers, G would appear to have properties that resemble infinity, even though it's actually a finite (albeit extremely huge) number.

The basic idea is this: the numbers we normally use are not merely finite; they're actually quite small. By "small", I'm not just talking about single or double digit numbers; I'm talking about numbers that can be written down in the usual notation, using digits, arithmetic operations like +, -, *, /, ^, in a practical amount of time. For our purposes, we can arbitrarily say that any number that can be written on a piece of letter-sized (or A4-sized, if you prefer) piece of paper, is considered a "small" number. Numbers like 1,000,000 or 1,000,000,000 fall in this category, but numbers like 10^100 (googol) or 10^10^100 (googolplex) are also included in this category. Even a number like 10^10^10^... (100 times) is included in this category. We may even allow the factorial function in our set of elementary arithmetic operations, so you could write down 11!!!!!!!!!!!!!!!!!!!!!!!!!!!... (filling the entire page with !'s) to describe a pretty large number, but for our purposes, we will still consider that as "small", because these numbers are tractible to conventional arithmetic operations, and can be written down in a practical amount of time in a practical amount of space.

But finite numbers include far more than merely those we can write down in a practical amount of time/space using conventional arithmetic operations. When you have some time, do read through Wikipedia's article on Graham's number to get an idea for how large a finite number can be. Graham's number is one of the candidates for G. Even though it's a finite number -- it has a finite number of digits, you can do arithmetic with it, and you can reduce it to zero using conventional arithmetic operations, albeit only after an unreasonably long amount of time, something on the order of a googolplex lifetimes of the universe and then some -- it is so huge that, for all practical purposes, it might as well be infinite. It's not actually infinite, of course; but it behaves a lot like infinity.

For example, G + any "small" number (using the above definition of "small") is so insignificantly different from G that it might as well be "approximately equal" to G. Multiplying G by any "small" number changes its relative magnitude so little that we might as well consider it "approximately equal" to G. Why? because it's so large that even though 10*G is ten times larger than G, from our perspective it hasn't changed the value of G by much. It's still that insanely highly-nested tower of 3^3^3^..., somewhat modified, but for all normal intents and purposes it's still an unimaginably huge number we cannot describe with normal words -- approximately the same kind of unimaginably huge number that G itself is. Similarly, G^G doesn't really change its value by that much; using Graham's system of notation, for example, G^G is still extremely close to g₆₄ (which is G itself), because the next number in the system, g₆₅, is so much larger than G that G^G, G^G^G, G^G^G^G^G^G^G, etc., have barely even begun to bridge the distance between g₆₄ and g₆₅. Relative to the distance between g₆₄ and g₆₅, G^G^G^G^G^G^G^G^G^G^G is less than the size of an electron relative to the width of the visible (physical) universe. So G^G^G^G^G...^G (where the number of G's is a "small" number as defined above) might as well be "approximately equal" to G.

So once we adopt G as a number in our everyday arithmetic, it more-or-less behaves like an infinite number, even though it's actually still finite. Given any "small" numbers x, y, the following holds:

G + x ≈ G
G * x ≈ G
G ^ x ≈ G
x ^ G ≈ G
x - G ≈ -G
1/G ≈ 0 (it's so miniscule that we can hardly tell its difference from 0)
x / G ≈ 0 (ditto)

G + G ≈ G
G * G ≈ G
G ^ G ≈ G
x + y < G
x * y < G
x ^ y < G
x ^ x ^ x ^ .... (y times) ... ^x < G

Starting to sound familiar? These properties resemble the behaviour of infinity in, for example, calculus. The only thing is, G is actually not infinite; it's actually a finite number. It just so happens to be a rather large finite number: so large that it's indiscernible with the usual arithmetic operations. Even though G*2 is actually twice as large as G, relative to G's immense magnitude it's "close enough" to G that we can say G*2 ≈ G. For everyday arithmetic with everyday numbers (i.e., "small" numbers as we defined above), G for all intents and purposes behaves as if it's infinite, even though it isn't.

And best of all? G-1 is actually distinct from G (even though it's so close that we can't really tell the difference!). G+1 is also distinct from G. So is 2*G, 3*G, G^2, G^G and so forth. You can take its factorial, compute √G, log G, etc., and all of these will have well-defined values that are distinct from G. (Albeit all of them would be so close to G that they are "approximately equal" to G.) And you can obtain G by repeated applications of the successor function.

You can also factor G (it happens to be a rather boring number in terms of factors: being a power tower of 3's, it's just 3 exponentiated to some unreasonably huge number -- a number that's -- you guessed it, "approximately equal" to G). It just so happens that G is an odd number, but we could easily have picked a different number than Graham's number: we could, for example, replace all the 3's in the construction of Graham's number with 2's instead, and we'd end up with a candidate for G that has many (perhaps even all!) of the properties your proposed "infinity" does.

Or we could construct a number that's the product of 1, 2, 3, ... Graham's number, and obtain a candidate for G that's divisible by basically every number we could write down on a piece of paper. It's actually not divisible by all numbers, of course: 1 + Graham's number, for example, would likely not be a factor. Above Graham's number its factors would quickly thin out. But from our POV, it might as well be a number divisible by every number, because any of the usual numbers we could think of, anything we could write down on a normal-sized piece of paper with +, -, *, /, ^, would be a factor of this number. The numbers that do not divide this number are so large and so far beyond the scope of numbers we usually work with, that we basically never encounter them. Such a number, for example, would be a pseudo-candidate for the "infinity divisible by every number" that I described in one of my posts. It's not an actual candidate, of course, because it's actually not divisible by every number. But it would pass any division test by any number we normally work with, i.e., any "small" number.

At this point, we might as well designate the "small" numbers as defined above to be "finite numbers" (or, to avoid confusion, let's call them "pseudo-finite numbers"), and rename G as "pseudo-infinity", and we'd have a system of finite arithmetic that, for almost all practical intents and purposes, have exactly the properties that you described in your proposed system of infinity. Of course, with the caveat that it's not an actual infinity we're talking about; G is actually a finite number. But it behaves so much like an infinite number that we could get away with pretending it's infinity and do arithmetic with it without running into contradictions. The only time you'd run into contradictions, or rather, what appears to be contradictions, is when you try to perform an operation that's on par with the derivation of Graham's number (or whatever system is used to derive the large finite number we designate as G). Then its infinity-like properties will start coming apart, and its finiteness comes back up to the surface. But as long as we don't do such operations (and we pretty much never do in "normal" arithmetic, of the kind we do in geometry), we're pretty safe in pretending that G = infinity.

[adam ∞]

Quickfur,

You have been pretty presumptuous about what you think I know, what you think I have looked into, what you think I understand, and you have been wrong about your presumptions. You jumped to conclusions about what you thought I knew and didn't know about set theory. You assume, that because I didn't mention certain types of symmetries in my introductory post, that I must have never even thought of it. Come on, give the idea a chance. Don't just assume I didn't think it through.

I know you are very knowledgeable about geometry, but your objections are the same objections people who have very little familiarity with geometry, who didn't follow the argument, come up with as a response without giving it much consideration. I know from your posts over the years, and from your website, that you are extremely intelligent and very insightful about geometry. But if you were to explore this aspect of the subject in depth, I think you would see that what you are saying is a bit naive.

It is provable, for example, that if infinity is not a power of 2, that an infinitely long line broken up into segments as edges cannot be split in half, then into quarters, then into eighths, etc, without splitting some of the edges themselves. Maintaining this property of being able to split things up evenly like this without splitting edges, for example, is far more crucial than maintaining 11-fold and 13-fold symmetry, which you mistakenly think will make it more symmetric. I think you are not appreciating why it is that you cannot have all of these types of symmetries all at the same time without losing other ones.

Give it some honest consideration, can you not see why the apeirogon cannot have 3-fold symmetry without seriously limiting other types of symmetry? Do you not see why some symmetries involved in this necessarily have precedence over others, and that is part of the whole challenge here, regardless of whether I emphasized this when I first introduced the concept? Maybe I took this as being understood to some extent when I was writing, and I should have focused on it more, and in the future I should be sure to spend time on it when explaining it, but at this point, you can see why every combination of all symmetries cannot work, right?

Maybe when I present all of this, I need to spend a lot more time on this aspect. A lot of it seems self evident to me, but it's one thing that a lot of people seem to get hung up on when I try to present the idea.

It's interesting, that one of your objections is that you don't think regular polygons converge to a circle.

That's fine if you disagree with that part, and if that part is not true, many of my conclusions would not be true, but that part is not my own unique claim. That is the main part of my theory that is most widely accepted.

I am interested to hear your interpretation of what the sequence of regular polygons does or does not tend to or converge to. Do you think it is something that is in any way related to a circle?


Given the conventional interpretation of the series of regular polygons converging to a circle, there is a deep connection between circles and infinite lines demonstrated by the apeirogon, and there are far reaching consequences of this!

I see it that the conventional interpretation of the properties of the apeirogon being like a circle and like an infinite line at the same time demonstrate that taking edges, and placing them next to eachother, in one direction, infinitely, will reach over to the other end an back to where you started. This has serious implications for space and time, for the number line, and for size itself!

Actually, given your difference of opinion about both the nature of infinity being inherently disconnected from the finite, and disagreeing about polygons converging to a circle, the idea no doubt lacks appeal to you.

But from my perspective, if this circle convergence interpretation is the case, the numerical properties of the number of edges of an apeirogon cannot be arbitrary. It can't be potentially infinite, or as I defined earlier, indistinct, disconnected, non-successive or non-arithmetical. If there was one more edge, or one less, it would not get exactly back to where it started. The number of edges has to be an exact number with specific properties. This is the natural limit of infinity. If we colored those edges, they would have to meet back up at the first edge, the coloring cannot be some special number with a paradoxical combination of properties of every number all at once. It can't be contradictory like it is in set theory.


You may be surprised to learn (if I haven't already mentioned) that Cantor is actually one of my biggest heroes! I really am fascinated by what he started. I do think he went down the wrong track with these diagonal arguments and focusing on real numbers, though, instead of being informed by geometry. I suppose they didn't have apeirogons back then just yet.

Anyway, I hope that you are having a good day, and I am appreciative to have this exchange, I have been enjoying the conversation.

Peace

[adam ∞]

I can go into more detail another time about what the difference is between an incredibly large finite number, a last finite number, and an infinite number in my theory. It's a good point to bring up, and it's on my list of questions I ask about different theories of infinity.

The key difference for me is that, in terms of pure mathematics, a polygon with G sides probably does not have edges that meet at 180°, and being even infinitesimally short of this will not have the topological properties of an apeirogon. The number of sides of an apeirogon has to be exactly one number, and not anything more or less. So approximately equal doesn't work.

I ask set theorists this same type of question. What if ω was really something like Graham's number and you just didn't know it? How can you be so sure that ω really is infinite, other than the fact that it has been declared to be so by definition? How is it any different to use an insanely huge finite number than an actual infinite number if you are going to just write it as a symbol with no specific numerical properties?

we could, for example, replace all the 3's in the construction of Graham's number with 2's instead, and we'd end up with a candidate for G that has many (perhaps even all!) of the properties your proposed "infinity" does.

True that constructing the number I discovered follows a very similar formula as Graham's number with 2's instead of 3's (though I based nothing on Graham's number at the time), but replacing the 3's with 2's in G would not have all of the properties of Ultranatural infinity. It's a damn good approximation, though, if you need one.

Graham's number replaced with 2's is of the same "species" as infinity, it is more or less a very large finite version of my number, (assuming G is finite) with the same numeric properties, but not the same "topological" properties. I suspect it's NOT a coincidence that G's 3's and my 2's are so similar, but I haven't found the connection. Numbers associated with hypercubes have similar propoerties as my number.

But outside of something like that, I am not dealing with approximately equivalent numbers or numbers that might as well be the same from an infinite perspective.

If you're going to use a functional finite stand-in for infinity, you're much better off pretending that some much smaller number of the form 2^2^2^2.. with the same numerical properties as infinity, rather than something based on three's that just happens to be unimaginably huge. The point of my number system is to keep track of precise, specific numeric qualities. Infinity and infinity minus 15 are approximately the same amount, but the types of numbers they are made up of are so different that they are like a totally different species or genus or family. Sixteen is way more similar to infinity than infinity minus 15 is. That is hard for some people to understand, but I think you have the comparison to higher dimensional shapes already figured out.

I use 65536 a lot in my quick calculations as a miniature analog for infinity, similar to how we use dimensional analogs to make sense of higher dimensional polytopes.

What you were talking about with 11-fold symmetry stuff is like using some really irregular concave star polyhedron with irregular faces or whatever, as an analog for an 8-dimensional hypercube just because the number of faces is almost the same, instead of using a lower dimensional cube or hypercube as an analogy. Hypercubes, 2-cube, 3-cubes, 4-cubes, 5-cubes, etc, are very closely related. More closely related than something that just happens to have approximately the same number of facets.


The "6" in 16 is in a way the same "species" of 6, it's there for the same reason, as the last 6 in the digits of infinity ...8736. Same with the 36 in 65536.

16 and 65536 are the lower level analogs of infinity, and pretty much anything of the form 2^2^2^2^2... will give you the hallmark traits in terms of the number theory properties. Graham's number replaced with 2's has as many "pieces" of the numerical properties of infinity in it as you'd like for numerical properties, but it will never have the same topological properties. The number 16 only has one little piece of it, 65536 has two pieces. One or two last digits can tell you a lot about a number though.


These are the numbers that are like infinity, not just doing 1x2x3x4x5x6x7x8x9x10x11.. until you reach some unimaginably high number, whether or not the total amount is closer to being infinite.

I hope that makes sense.

[quickfur]

[...]
It is provable, for example, that if infinity is not a power of 2, that an infinitely long line broken up into segments as edges cannot be split in half, then into quarters, then into eighths, etc, without splitting some of the edges themselves.

By the same logic, if infinity is not a power of 3, then an infinitely long line segmented into edges cannot be split in thirds without splitting some of the edges. So why is this less important than division into halves?

What makes splitting into halves more important than thirds? Or, for that matter, any other equally-divided segments? Because a circle certainly can be divided into n equal parts, for any integer n≥2. So if indeed the apeirogon is identical to the circle, as you assert, then it also ought to be divisible into n equal parts for any integer n≥2. That this cannot be done without contradiction is a sign that perhaps our assumption that apeirogon = circle is problematic. Either that, or some of our assumptions about the nature of infinity is invalid.

Maintaining this property of being able to split things up evenly like this without splitting edges, for example, is far more crucial than maintaining 11-fold and 13-fold symmetry, which you mistakenly think will make it more symmetric. I think you are not appreciating why it is that you cannot have all of these types of symmetries all at the same time without losing other ones.

I am acutely aware that you cannot have all these symmetries at once under your construction. That's why I deliberately focused on this particular point -- because this is where your system contradicts your assertion that apeirogon = circle. Do you agree that a circle is divisible into n equal parts for all integer n≥2? I hope it's self-evident that this is so, otherwise we have incompatible definitions of "circle" here, and further discussion is futile. Now, accepting that a circle is divisible into n equal parts, if we assume your postulate that an apeirogon is equal to a circle, then it follows that the apeirogon must also be divisible into n equal parts for all n≥2. The fact that this leads to contradiction under your construction proves that either the assertion that apeirogon = circle is wrong, or something is amiss with the construction of your system of infinity.

Give it some honest consideration, can you not see why the apeirogon cannot have 3-fold symmetry without seriously limiting other types of symmetry? Do you not see why some symmetries involved in this necessarily have precedence over others, [...]

And by what criteria are we to decide the precedence of one symmetry over another? I don't see any that would simultaneously satisfy your objections and at the same time preserve your assertion of the equivalence of apeirogon and circle.

It's interesting, that one of your objections is that you don't think regular polygons converge to a circle.

I'm glad you brought up this point. Note that I never said regular polygons don't converge to a circle; what I said, repeatedly, is that the assertion apeirogon = circle leads to contradiction under your construction.

But more pertinently: just because a sequence of something converges to something, does not necessarily mean the sequence equals that thing. The object at the limit of the sequence may have properties that are incompatible with every object in every finite initial segment of the sequence.

For example, consider the binary representation of a real number between 0 and 1. For example, 0.01010101... = 1/3. We can construct a sequence by taking initial segments of that infinite binary expansion, i.e., 0, 0.0, 0.01, 0.010, 0.0101, 0.01010,, 0.010101, and so on. Notice that by definition of binary digits, every position in the mantissa represents a (negative) power of 2. For example, 0.01 = 1/4, 0.0101 = 1/4 + 1/16, and so on. The denominator of every non-zero term is a power of 2, meaning that multiplying the number by 3 can never eliminate the denominator. There will always be a residue of the form k/2^i for some k, i. This means every member x of our sequence has the property that 3*x is non-integral. However, we cannot conclude from this that the limit of the sequence retains this property; indeed, the sequence converges to 1/3, and 3*(1/3) = 1, which is an integer. Equivalently, just because 3*0.01010101... = 1 = an integer, does not permit us to conclude that every member of the sequence 0, 0.0, 0.01, 0.010, 0.0101, ... must also produce an integer when multiplied by 3.

I.e., properties that hold for finite initial segments of a sequence do not necessarily carry over to the object at the limit. The limiting object may have properties that no member of the sequence has, and conversely, the limiting object may lack some properties that every member of the sequence possesses.

[...]
I am interested to hear your interpretation of what the sequence of regular polygons does or does not tend to or converge to. Do you think it is something that is in any way related to a circle?

The answer is that you may obtain different results depending on exactly what sequence you're constructing. Here are two of the most obvious ones:

1) Construct a sequence of n-sided regular polygons of identical out-radius. Let's say this out-radius = r. Then the object obtained at the limit is a circle of radius r. Since the edges of the polygons decrease in length as n increases, at the limit the edge length becomes equal to 0, i.e., the edges become indistinct, so some properties of finite polygons such as having alternating vertices and edges may no longer hold at the limit. Other properties still hold, though: such as the polygon being contained in a circle whose radius is equal to the outradius. In this case, the circle is identical to a circle with radius equal to the outradius.

2) Construct a sequence of n-sided regular polygons of fixed edge-length. Let's say we fix the edge length to be 1. Then the object obtained at the limit is an apeirogon, which preserves the alternating vertex/edge structure of the finite polygons, but the outradius diverges to infinity, so the apeirogon cannot be inscribed in any circle.

There are many other constructions that either lead to limiting objects that are either isomorphic to one of the above, or that diverge altogether (and so yield no useful information).

Given the conventional interpretation of the series of regular polygons converging to a circle, there is a deep connection between circles and infinite lines demonstrated by the apeirogon, and there are far reaching consequences of this!

There may very well be far-reaching consequences; but that needs to be explored carefully and rigorously. For example, in what sense exactly is a circle equal to an apeirogon? A circle has a finite radius, for example, but an apeirogon does not. A circle has full circular symmetry, but according to your analysis, an apeirogon cannot be divisible by 3, so it lacks triangular symmetry, and consequently, lacks circular symmetry. So obviously, the two cannot be the same thing. Maybe they share some properties in common, but it seems erroneous to simply assume without proof that they are equivalent and that properties that hold for one can simply be carried over to the other.


But, if you would indulge me, a little footnote: if you construct a n-polygon where n = G, a very large finite number like I describe in my other post, then it could indeed have the properties that you ascribe to the apeirogon. At least, it would have properties sufficiently close to an apeirogon that, for the most part, we could assume without contradiction that it's an apeirogon. Consider it. It's one way of constructing a fully-consistent, contradiction-free system that has virtually all the properties you desire in your constructions.

[adam ∞]

But, if you would indulge me, a little footnote: if you construct a n-polygon where n = G, a very large finite number like I describe in my other post, then it could indeed have the properties that you ascribe to the apeirogon. At least, it would have properties sufficiently close to an apeirogon that, for the most part, we could assume without contradiction that it's an apeirogon. Consider it. It's one way of constructing a fully-consistent, contradiction-free system that has virtually all the properties you desire in your constructions.

Do you mean a number like Grahams number, but with 2's instead of 3's? If so, that number is identical to one of the numbers in my infinity sequence 2^2^2^2^2... My notation just gets there way slower. Graham's number with 2's instead of 3's is part of my sequence either on the way to infinity or past it 2^∞, 2^2^∞ toward ∞^∞. Technically it is possible that they are exactly the same number.

We assume that Graham's number is finite, but I don't know if we can safely assume that. I certainly can't make that assumption. For you, it probably has to be finite, according to the definitions you accept. But put another way, the number of edges of Coxeter's apeirogon with edges meeting at 180 degrees might by way way way way WAYY less than Graham's number! But with both of them still being infinite! Actually, just the number of digits of graham's number, or the number of digits of the number of digits could be infinite!

I know this is very controversial. To most people, it sounds like what I am saying is clearly impossible, how could I be claiming that Graham's number could even in principle be infinite? But I don't hold to the definition that infinity cannot be obtained by some modification of finite numbers.

How could this be so? It's because of how huge these notations can get very quickly, because it is not staying to one dimension, and how unimaginably larger 2^n can be compared to n^2 for large numbers and especially for infinite numbers. The first infinite number, confined to one dimension, can become pretty small compared to having infinitely more, then infinitely infinitely more, then infinitely infinitely infinitely more units for each new dimension. The differences between infinity of higher dimensions is so incredibly large, infinitely infinitely infinitely infinitely large, that when we use higher dimensions for notation (even just with exponents) it can get so much bigger than we realized, compared to just one line in one dimension.

Graham's number pertains to something like all of the connections between edges of some hypercube in some unimaginably high dimension, right?

And in some incredibly high number of dimensions where some new unexpected property happens for the first time..

It's not entirely outside of the realm of possibility that the hypercube in question is in dimension n=square root of infinity, Graham's actual number (with 3's) corresponds to the number of connections between edges, while also maintaining the possibility that my number is identical to graham's-number-with-2's, corresponding to the number of connections between vertices of the same hypercube rather than edges. I am just saying that something like this is totally possible within what I have proposed with the definitions I have used.

I only came to the conclusion that the number of digits of infinity can be finite, and that infinity can be a number that is a modification of a finite number, relatively recently. I haven't had very much time to really work out all of the implications of this, or go back and revisit graham's number in depth in light of this.

But one of the first things I thought of when I came to that conclusion was, holy crap! That means that Graham's number could possibly be infinite! That is so weird, and to be honest, it kind of creeps me out! The thought of figuring out infinity in enough detail to be able to write it on a page, or in a thousand pages, in similar up arrow notation, similarly, for some reason, really gives me a very eerie, spooky feeling.

[adam ∞]

Hey Quickfur, and also mr_e_man, or Wendy, or anyone else reading this if you have a similar view!

I am genuinely interested to hear this if it is the case that according to your own view, that the nature of space, geometry, numbers, etc, is infinite, but with some kind of discontinuity between the finite and the infinite-

How does that play out, like, what is your conception of what would happen if you conceptually started at some origin point in space (either physical space or geometrically) and conceptually moved from some point you start at, over to one side, for example in a straight line, say, as fast as you'd like..

Do you think of it as just never getting to anywhere that could be considered infinite, that no matter what, it is just never ending no matter what? Or that in some sense, there is some final destination you can get to which is in some sense infinitely far away, completing some kind limit at which you have traversed an infinite distance?

[ To be clear, when I say "infinite" here, I am asking about an ACTUAL infinity. Unless you explicitly only believe in a POTENTIAL infinity which is "never ending", never reaching some limit.. in which case, please make that clear.]

Like, if the finite does not lead to the infinite, if that is what I understand your meaning to be, if there is, say, a point at infinity in projective geometry, or an infinite 3-dimensional space- what does it mean to infinitely traverse this space, or for some line to start at some point and be so long that it is considered infinitely long without a discontinuity presumably in the line itself (or do you believe that infinite lines have some kind of gap in them at some point? If so, what does that even mean? Like some kind of hole that is just missing or a mystery zone?) Is there literally no transition from finite to infinite, or it is just completely unknown?

Like do you literally think of just a blank, missing area that you are unsure of, that is somehow in between the two that just can't be fathomed? Or like a part you could just never see?

I am really trying to wrap my mind around this concept of what it means for there to be a discontinuity between the finite and infinite.

It seems that this type of thing is just something that is deeply ingrained philosophically, where whether we interpret space as being continuous or discrete, or finite on a small scale with continuity to the infinite on the large, or finite on a small scale, but infinite on the large scale but somehow without the two connected, It's very difficult to establish which view should be adopted, because all of the evidence, to me suggests and support one interpretation, and all of the evidence to you seems to you to support your interpretation.

<I had a similar conversation with someone about the nature of time, whether only the present is currently real and happening, or if in some sense, the past is real, or that the future is real, and it is all there in some sense. All of the evidence we mentioned to support our position was interpreted by the opposite person to obviously support their position, in a way that seemed obvious to both of us.>



Either way, I would be very appreciative if you could share your perspective on the discontinuity between the finite and infinite in a way that might help me grasp what it is you mean in a real world physical or geometric scenario.


Like, suppose we have a grid with a black square in the middle, a line of blue square to the right, and red squares to the left, and this pattern continues. It's a zoomed in view of an infinite space which extends far beyond what is seen in the picture.



If you conceptual followed the blue path, to the right, starting at the black square-

Do you just think of it as always staying finite?

Suppose there is a black square infinitely far away.

Do you think of it in such a way that, if you followed the blue path toward the black square, you could in some sense never get there (even conceptually)? That you could only get there by being magically transported, skipping the space in between? I don't get it.

What if you traveled conceptually at a speed that is like, 16ths of infinity within a minute or something like that.

Or does a concept like that even amount to something in your view? Is there a way conceptually to start at some point, starting with a finite amount, traversing it in one minute, from 1 to infinity? Starting out at some finite speed, and ending at a speed that is some fraction of infinity?


Like, there is notion that you can (conceptually) "count" to infinity in a short period of time, if you just speed up each time you "say" a number, like a converging infinite series 1 + 1/2 + 1/4 + 1/8... etc.

How do you make sense of something like that, either the counting thing, or the converging series, if there is a discontinuity between the finite and the infinite?

If there is no continuity between finite and infinite, can it ever converge? In what sense does it "get" to infinity if there is not some kind of continuity or transition from the finite to the infinite?

Sorry if this is repetitive, I am trying to reiterate my point a few different ways. Genuinely interested to try to comprehend your perspective on this. Thanks.

[quickfur]

Well, clearly the problem here is that we're using the same words with different definitions, so no wonder we're talking past each other.

The generally-accepted definition of a finite (natural) number is any number reachable from 0 by repeated applications of the successor function. Or, to be absolutely unambiguous, a formal definition:
(a) 0 is finite;
(b) if x is finite, then x+1 is also finite.

It can be proven that Graham's number is finite according to this definition, even though it's so large that it exceeds most people's intuition of "infinity".

Based on the above definition, one can prove that the sum, multiplication, or exponentiation of any two finite numbers must also be finite. This immediately excludes the possibility of ever reaching an infinite number by finite applications of any arithmetic operation. The only way it can be otherwise is if you use a different, incompatible definition of "finite".

In fact, given any definition of "infinity" that involves reaching "infinity" from finite applications of any arithmetic operation on finite numbers, it can be proven that such an "infinity" is actually finite under the above definition. This is why I brought up Graham's number: based on your descriptions of what your concept of "infinity" is, I suspected that what you call "infinity" is actually not the same infinity as used in mathematics, but rather a large finite number according to the above definition.

And mind you, Graham's number is but a convenient example of a large finite number that came to mind; there isn't really any special properties that would set it apart from any other large finite number. One can construct far larger finite numbers than Graham's number, that make it look like child's play in comparison. One common way of doing this is by using functions from the so-called "fast-growing hierarchy", which are indexed by ordinals. Graham's number can be generated by functions relatively low in the hierarchy; so correspondingly it's not very large as far as large finite numbers go. A much larger (but still finite!) number can be generated by using the function indexed by the so-called Fefermann-Schütte ordinal. Numbers produced by this function are so huge that they defy all conventional descriptions; the fast-growing hierarchy is pretty much the only way to describe them. But they're hardly the "largest" among the finite numbers. One can generate even larger numbers by using the function indexed by, for example, the Bachmann-Howard ordinal. Such numbers are provably finite (according to the above definition), but are so intractably huge that it's virtually impossible to prove any concrete properties about them. Such properties certainly exist, but the magnitude of the numbers involved make it impractical to compute any of them.

And it's possible to go even farther. One can reach for the Church-Kleene ordinal, for example, to index the fast-growing hierarchy, which produces a function with comparable growth rate to the so-called Busy Beaver function. This function grows so fast that it's actually uncomputable: it can be proven that there does not exist any method of computation that can compute all of the values of this function. Certain specific values can be computed, of course, by painstaking ad hoc proofs of the non-termination of large numbers of Turing machines: the first 5 or 6 values of the Busy Beaver function are known. But beyond these scant few known values, the function grows so fast that it defies all methods of computation. It can be rigorously proven that this function has an incredible growth rate that outstrips all computable functions. Correspondingly, applying this function to a suitably large number (probably 100 or 1000 or somewhere thereabouts would be sufficient) would produce a finite number so unimaginably gigantic that it's actually impossible to compute. Not just infeasible to compute, mind you, but provably impossible even in theory.

Yet at the same time, it can also be proven that this number is nevertheless finite (in the sense defined above). Finite numbers, as defined above, include SO MUCH MORE than most people's intuition of "infinity" that it's almost laughable to read about supposed "infinities" that can be reached by elementary arithmetic operations. Such "infinities" are so small they're obviously finite, and rather low down among the smaller finite numbers at that, that calling them "infinity" frankly sounds like a joke. Unimaginably huge (finite!) numbers like the uncomputable one described above aren't even close to (the mathematical sense of) infinity; entire hierarchies of ever-faster-growing functions exist above the Busy Beaver function; it is but the smallest and slowest among them! Correspondingly, the kinds of uncomputably large finite numbers produced by its bigger brothers dwarf our uncomputably-huge number by far. And even these bigger uncomputable numbers are merely finite; we've barely even begun to take a single step in the direction of a real infinity. There's a long, long, way to go before we can even get anywhere close.

[adam ∞]

Quickfur,

Right, I believe infinity is successive, you believe it's non-successive, and therefor you think that something that is successive must be finite.

But why do you believe this, other than just accepting that as the definition, "by definition". Where does this definition come from? Why do you think infinity can only mean that one particular definition and not something else?

Obviously a huge amount of people believe that 1+1+1+1... equals infinity, by just adding ones until it gets there. So the definition you gave is not the only one that is widely accepted.

I was trying to establish some terminology in my original post so that we could at least discuss it in a way where we make distinctions between these concepts without having to accept someone else view on it.

You are convinced that a nonsuccessive infinity has to be the definition of infinity. I see it as being meaningless, contradictory, and a major problem that needs to be resolved in any theory of infinity. It's like a broken part of the theory.

It's interesting that you don't see a discontinuous gap as a major foundational problem.

Finite numbers, as defined above, include SO MUCH MORE than most people's intuition of "infinity" that it's almost laughable to read about supposed "infinities" that can be reached by elementary arithmetic operations. Such "infinities" are so small they're obviously finite, and rather low down among the smaller finite numbers at that, that calling them "infinity" frankly sounds like a joke.

There is a flip side to this, though.

Going so far over the smallest infinity, and believing in infinite orders of infinite orders of infinite order of infinity like ∞^∞^∞^∞^∞... infinity times, or some of Cantor's more imaginative incredibly high ordinals, to me comes across as pretend. It's total fantasy. It doesn't correspond to anything real or meaningful. Infinity is some exact, precise amount. Greatly underestimating it can seem silly, but so can overestimating it by so much that nothing that is being said has any coherent meaning and no longer corresponds to reality is just as silly, or in a way, much much sillier.

It's just potential infinity mislabeled as something else.

There is still a lot of potential infinity left over in your conception of infinity.

[adam ∞]

Here are some depictions of number lines, etc, in various number systems that use infinite numbers!

If infinity is defined as being disconnected from the finite, with no transition between the two, does that notion apply to any of these images?
If it does apply, what is it intended to mean? To me these all appear to have very direct, smooth, connected transitions from the finite to the infinite, following a very explicit path from one to the other.




^ The supernatural numbers



^Surreals



^Hyperreals


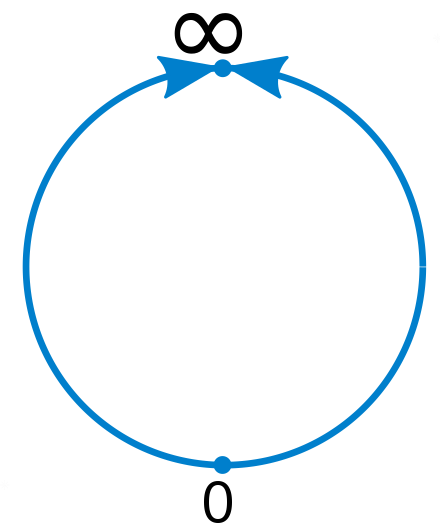
^ Projectively Extended Real Number Line



https://upload.wikimedia.org/wikipedia/commons/thumb/6/6c/Extended_Real_Numbers.svg/1024px-Extended_Real_Numbers.svg.png

^Extended Real Numbers

[mr_e_man]

I'll take the position that "actual infinity" or "completed infinity" doesn't exist. There's only "potential infinity". (Though, I'm not sure my ideas of these are the same as yours.)

Of course, given any system of numbers, we can always introduce a symbol '∞' which is at first meaningless, except that it doesn't represent a number in the system; and then define operations and relations between this new "number" and other numbers. For example, for any Natural Number n (that is a number which can be reached by succession from 0), we define n+∞ = ∞+n = ∞+∞ = ∞, and n < ∞ and not ∞ < ∞ nor ∞ < n. Then we can prove that certain properties of the system; such as commutativity, x+y=y+x; or transitivity, x<y and y<z implying x<z; or additivity, x<y implying x+z<y+z; are still satisfied, or are not, in the extended system with ∞. This is just one of many possible meanings of '∞'. Other meanings could have ∞+n ≠ ∞.

If you conceptual followed the blue path, to the right, starting at the black square-

Do you just think of it as always staying finite?

Suppose there is a black square infinitely far away.

Do you think of it in such a way that, if you followed the blue path toward the black square, you could in some sense never get there (even conceptually)? That you could only get there by being magically transported, skipping the space in between? I don't get it.

Yes, it always stays finite.

There's no such thing as "infinitely far away". The Euclidean plane is potentially infinite. Given any line segment, we can attach to it another line segment, extending in the same direction, thus increasing the length of the segment; still the length is finite, but there's no upper limit on the length. For any two black squares in the plane, there is a finite distance between them.

There could be a black square not in the plane, such as "above" the first black square, in a parallel plane. Then there is no finite distance between the two squares, in the sense that a Flatlander at the first square can never reach the second square. I'm not saying that a point at infinity is necessarily equivalent to a point in a higher dimension, but there is some analogy.

Like, there is notion that you can (conceptually) "count" to infinity in a short period of time, if you just speed up each time you "say" a number, like a converging infinite series 1 + 1/2 + 1/4 + 1/8... etc.

How do you make sense of something like that, either the counting thing, or the converging series, if there is a discontinuity between the finite and the infinite?

If there is no continuity between finite and infinite, can it ever converge? In what sense does it "get" to infinity if there is not some kind of continuity or transition from the finite to the infinite?

It never gets to infinity. The sequence 1, 1.5, 1.75, 1.875, ... never reaches 2. What it means to say that it "converges" to 2, is... Well, we need to talk about quantifiers.

Also we need to define the sequence! The first few terms do not determine the rest of the terms. Your sequence 1, 1/2, 1/4, 1/8, ... could have the n'th term being 1/2ⁿ, or it could have the n'th term being

24/(24 + 14n + 11n² - 2n³ + n⁴)

(taken from Mathologer's recent video). A sequence is infinite; it's not enough to know any finite number of terms. But we can't write down an infinite number of terms either. Instead we need some finite "rule", such as the algebraic expression above, that tells us what the n'th term should be, for any n, any one of potentially infinitely many possible values of n.

(Actually, there may be some sequences which can't be described by any finite rule. But that's tangential.)

If the n'th term is indeed 1/2ⁿ, then the sum of the first m terms is

1 + 1/2 + 1/4 + 1/8 + ... + 1/2^m = 2 - 1/2^m,

which can be proven by induction. This "obviously" converges to 2 because 1/2^m converges to 0 as m approaches infinity.

Definition of convergence: A sequence x_n (of rational numbers, or real numbers) converges to a number c if the difference (x_n - c) converges to 0. In turn, a sequence x_n converges to 0 if the following statement holds:

For any positive rational number ε, there is some natural number M (generally large, generally depending on ε), such that the inequality -ε < x_n < ε is true for all natural numbers n > M.

The universal quantifiers "any" and "all" signify a potential infinity here.

For our sequence 1/2ⁿ, obviously the left inequality is true: -ε < 0 < 1/2ⁿ, therefore -ε < 1/2ⁿ, regardless of the values of ε and n. So let's look at the right inequality: 1/2ⁿ < ε. We need to find a rule that gives a number M, depending on ε, that makes the inequality true whenever n > M. One possible rule is M = ⌈log₂(1/ε)⌉ (if you accept the existence of the logarithm and ceiling functions). Indeed, if n > M, then

2ⁿ > 2^M
1/2ⁿ < 1/2^M

and, by definition of ceiling and log,

M ≥ log₂(1/ε)
2^M ≥ 1/ε
1/2^M ≤ ε

and it follows that 1/2ⁿ < ε, as required. This proves that 1/2ⁿ converges to 0.

The idea behind the definition of convergence is that x_n gets "close enough" to c, as close as can possibly be desired without requiring it to be exactly c, when n gets "large enough" (but still finite).

Now I'm wasting time teaching you calculus. You can learn that anywhere (if you haven't already).

[adam ∞]

Thank you for explaining your view on this, mr_e_man! That is very interesting!

It sounds like you are describing something that is always finite, but somehow also "unlimited" at the same time.. like an "unlimited finity", is that accurate?

There is no upper limit on length, but all lengths are necessarily finite.

Is there no sense, then, of a total length of space? Or a total volume of the entire Universe?

Is the entire Universe limited or unlimited?

I am not sure what you are saying, but if it is the case that you are saying that something can be finite and unlimited at the same time, How can that be? If something is unlimited, it's not also finite, right? But if the unlimited cannot be achieved, how can there be a geometric space or a Universe that is considered unlimited?

I'll take the position that "actual infinity" or "completed infinity" doesn't exist. There's only "potential infinity". (Though, I'm not sure my ideas of these are the same as yours.)

When you say "potential infinity" you mean a finite potential infinity right, and not an infinite potential infinity? I am pretty sure you mean a finite potential infinity, but I am not 100% sure. Finite potential infinity meaning, no matter what, it is always finite, and only potentially reaches toward some nonexistent concept of a completed infinity.

Versus an infinite potential infinity, which can be an unlimited, never ending amount, but is at least more than some smallest actually infinite amount. It is unlimited, but has surpassed the first infinite number.

When you say "potential infinity", to you, does that amount to something that is finite, or something that is infinite?

Yes, it always stays finite.

There's no such thing as "infinitely far away". The Euclidean plane is potentially infinite. Given any line segment, we can attach to it another line segment, extending in the same direction, thus increasing the length of the segment; still the length is finite, but there's no upper limit on the length.

You mention line segments, but what about lines and rays? Does a ray in any sense end? If it doesn't end, that seems to suggest it is not finite.

To clarify here, are you saying that, although I proposed that the space with colored squares is infinite, are you saying that it cannot be infinite, and that it is actually finite? Or are you saying that it is an infinite space, but that this means that space is unlimited, with every distance being limited and finite? But if that is what you mean, what about the distance of the entire space, which I thought you are saying is unlimited...I'm having a hard time making sense of this.

Is the entire space finite or infinite?

Can there be something so far away that no finite line can reach it?

For any two black squares in the plane, there is a finite distance between them.

Suppose you start at that black square (square "0"), and you have two new black squares (1 and 2) moving farther apart from eachother, square 1 to the right moving along the blue path, square 2 is to the left, moving along the red path. If these two black squares can never get infinitely far from each other, as you said, does this mean that as they get further and further apart, there will always be a blue square to the right of square 1, and a red square to the left of square 2? If so, doesn't this imply that there is in some sense always a greater distance between some blue square and some red square beyond the two black squares no matter how far apart they move? If so, if there is no limit to how far the black squares can be apart, but also always have to be a finite distance apart, but the red and blue squares are extending in both directions unlimited, but in some way, further than the black squares, how does that make sense?


Do you believe that the physical space of the Universe, at some exact instant, has a total volume? You don't think of spatial extension as coming to some kind of end, right?

How can it have no upper limit on length, and be limited at the same time?

[quickfur]

Quickfur,

Right, I believe infinity is successive, you believe it's non-successive, and therefor you think that something that is successive must be finite.

But why do you believe this, other than just accepting that as the definition, "by definition". Where does this definition come from? Why do you think infinity can only mean that one particular definition and not something else?

It's not a matter of belief. It's a matter of simple definition. We start from 0, and repeatedly add 1 to obtain 1, 2, 3, and so forth. We can go as high as we like, and it doesn't matter: there's no upper limit. No matter how big a number you obtain, adding 1 gives you a bigger one. This is pure logic, there's no belief involved here. For convenience, we give the label "finite" to any number that we can possibly obtain this way, so that we have an easy way of talking about the things that we may obtain by this process. If you don't like the label "finite", call it something else. Call it "reachable numbers" if that suits you better. It doesn't really matter what the exact name is, as long as we use the label consistently. Using this system of "reachable numbers" (what most people call "finite numbers" but to drive the point home I'm going to just use "reachable numbers" in this post), we can do arithmetic, we can interpolate between them to create fractional numbers, and so forth.

Sometimes, though, we run into things that this system of numbers cannot express. For example, we may represent straight lines by the linear equation y = ax + c, where a and c are fixed constant reachable numbers, and y and x are allowed to freely vary among the reachable numbers. This equation can represent almost any line: except on particular one: the vertical line. Why is that? Think about it. The reason is that for every other line, a single value of y corresponds with a single value of x, and x and y cover every reachable number. But for the vertical line, the only allowed x value is a single fixed number, and the allowed y values are ... every reachable number. Since y can't represent multiple values at the same time, our equation breaks down, and we can't use it to represent vertical lines.

Now, the constant a is a nice way of describing the slope of a line: it's the ratio of the y value to the x value of any point on the line. In a vertical line, however, this ratio cannot be defined within our system, because y becomes multi-valued and x becomes fixed. Conceptually, however, we'd like to imagine that the slope of a vertical line is some kind of special thing, commonly called "infinity", but really, that's just a label for an object that doesn't exist in our system of reachable numbers. Since the slope of nearly-vertical lines grows without bound as the line becomes closer to the vertical, we make the conceptual leap that the slope of a vertical line must be in some sense "infinite", or, to be consistent with the terminology we chose, it's "unreachable" -- in the sense that it's not among our reachable numbers, since it can be proven that no matter what reachable number you pick for the constant a, it will not produce a vertical line. The larger the value you pick, the closer it is to being vertical, but it will never be exactly vertical. So this imaginary slope of a vertical line cannot be a reachable number; it's some special object outside our system with special properties not shared by any reachable number.

Let's call this special object S (I don't want to use the infinity symbol because I want to emphasize that this is purely a label, with no other connotations attached). S has some unusual properties that reachable numbers don't have. For one thing, when you substitute S into the above linear equation, you get y = Sx + c, but this new equation doesn't behave like the old one, because it only holds when x is a specific value (because that's the definition of a vertical line: x is fixed). When x is not that special fixed value, the equation doesn't hold no matter what value of y you plug in. Also, when x is that special fixed value, then the equation holds no matter what value y has. So clearly, S is not an ordinary object that obeys the usual laws of equations. When S multiplies x, it produces a quantity that does not correspond with any of the reachable numbers: S*x equals (y-c) for every value reachable value of y when x is the horizontal coordinate of our vertical line, but S*x does not equal any reachable number when x is not the horizontal coordinate of the vertical line. It's something that lies outside of our system, and has unusual properties that no reachable number has. Therefore, it is not a reachable number; it must be something else altogether. It's an ideal object that we invented to represent the slope of a vertical line, but otherwise doesn't behave like a reachable number. When you put S into the above linear equation, it changes the rules of how equations work.

Normal arithmetic laws dictate that if y = ax + c, then a = (y - c)/x. But if a = S, then we get S = (y - c)/x, which immediately leads to a contradiction. For example, suppose our vertical line has x=0. Then we have S = (y - c)/0, which no reachable number satisfies. Furthermore, suppose our vertical line has x = 1. Then we have S = (y - c)/1 = (y - c), but then (y - c) has multiple values. In fact, it can take on the value of any reachable number, which implies that S is simultaneously equal to every reachable number. Clearly, no reachable number has that property either, so we are forced to conclude that S cannot behave like an ordinary reachable number. It must have some property that causes it not to obey normal arithmetic rules.

Well, either that, or S doesn't exist -- but if that's the case, then our entire discussion here is null and void. So we assume that S exists, and conclude from the above that it must possess some peculiar properties indeed. Definitely does not behave like a reachable number. You can call it whatever you want, infinity, non-number, S, whatever... it's just a label. The pertinent fact is that it's a thing that behaves differently from reachable numbers.

Obviously a huge amount of people believe that 1+1+1+1... equals infinity, by just adding ones until it gets there.

Unfortunately, you can't build a consistent system of mathematics upon belief. 1+1+1+... never equals infinity (I'm talking about infinity in the sense mathematicians define it, not what most people imagine it to be); it only approaches it. Or, if you don't like the word "infinity" as mathematicians use it, think about the "reachable numbers" defined above. Obviously, 1+1+1+... always remains within the realm of reachable numbers. You may say, well at some point it must reach something called "infinity"? Sure -- but that "infinity" would be a different thing from what mathematicians understand infinity to be. As far as I'm concerned, that's just some large finite number (large reachable number, if you prefer) like Graham's number. It's not the same thing as the infinity mathematicians talk about, though.

So the definition you gave is not the only one that is widely accepted.

Strange, last I checked, the definition I gave is the most widely-accepted among mathematicians.

I was trying to establish some terminology in my original post so that we could at least discuss it in a way where we make distinctions between these concepts without having to accept someone else view on it.

That's fine. Just be aware that your definition of finite numbers differ from the one used by mathematicians, so you should at least note this fact so that people don't confuse your use of "finite number" with its mathematical usage.

Also, do note that the mathematical definition of "finite number" includes all all numbers reachable by applications of the successor function; meaning that if your version of infinity is reachable in this way, then according to the mathematical definition it's not an infinite number, but rather a (large) finite number. If you wish to nevertheless call it infinity, that's fine, but don't be surprised if mathematicians consider it as a finite number -- because that's what it is, according to their definition.

You are convinced that a nonsuccessive infinity has to be the definition of infinity. I see it as being meaningless, contradictory, and a major problem that needs to be resolved in any theory of infinity. It's like a broken part of the theory.

I never said I was convinced that it must be non-successive. All I said was, if you define the natural numbers as I defined the reachable numbers above (which is, btw, how mathematicians define the natural numbers), then none of them are a candidate for infinity.

There are actually many theories that introduce infinity-like objects that behave in different ways from each other. For example, in the 1-point compactification of the complex numbers, you add a special element E (usually the infinity sign is used, but that's really just a symbol; you can use any other symbol as long as the definition remains the same) that behaves like a "point at infinity", and that gives you a Riemann sphere. This element E does not behave like any of the Cantorian infinities. It's simply a special element added to the system in order to confer some nice closure properties to the complex plane. The surreal numbers also feature many non-finite elements, many of which do not behave like any of the Cantorian infinites (even though Cantorian ordinals are used to construct the surreal numbers). There are a multitude of other objects that behave in some way like infinite elements, but they are not necessarily "the" infinity.

The important thing about all these systems is that they must be consistent, and free of contradictions.

It's commendable that you've set out to solve what you perceive to be a major problem that needs to be resolved in a theory of infinity; but it's unfortunate that from what you've said so far, what you call "infinity" in your system appears to be merely a large finite number (large reachable number, if you prefer), not an actual infinity. When you nevertheless equate it with an infinite quantity, then you run into contradictions. For example, given any supposedly-infinite number E that's reachable by repeated applications of the successor function, it can be proven that a regular polygon with E vertices does not have a 180° angle between adjacent edges. It's very close to 180° when E is very large, but it never equals 180°. Any number X for which a regular polygon with X vertices has a 180° angle between adjacent edges must have at least one infinitely-long "gap" that cannot be bridged by the successor function. It doesn't have to be right before X like the Cantorian infinities; under a suitable rearrangement the gap can be shuffled to a different place (the middle, for example). But it cannot be eliminated, and it cannot be crossed with the successor function. Anything that can be crossed with the successor function is provably finite (according to the mathematical definition).

You don't have to accept the mathematical definition of finiteness; but just be aware that chances are, whatever you construct that's reachable with the successor function will ultimately fall under the mathematical definition finite numbers, no matter what you label it to be.

It's interesting that you don't see a discontinuous gap as a major foundational problem.

If you follow (even if just for the sake of argument) the mathematical definition of the natural numbers, and consider a situation where an infinite quantity is needed (e.g. the slope of a vertical line scenario I gave above), then you'll be forced to conclude that any such infinite quantity cannot possibly be reached from the natural numbers by applications of the successor function. Whether or not this is a major foundational problem, it's nonetheless an inescapable fact. This "gap" is an inherent feature of an infinite quantity, like it or not.

You can try to define finiteness differently in order to work around this, but you should be aware that the mathematical definition is a superset of any such other definitions, so any candidate infinities you construct that way will, in all likelihood, fall under the mathematical definition of "finite", and will fail to possess the needed properties to satisfy the role of an infinite quantity in that situation. For example, you may define a system wherein some element E is reachable from 0 by the successor function but isn't finite (under a custom definition of "finite"). You can label it as "infinity" or whatever else you wish, but it will be provable that E is merely a finite number in the mathematical definition of finite numbers, and consequently, E will lack the properties that characterize infinite quantities -- e.g., E cannot serve as the slope of a vertical line because the resulting line would not actually be vertical.

[adam ∞]

Quickfur, you wrote:

I'm talking about infinity in the sense mathematicians define it

It is not the case that there is only one accepted mathematical definition of "infinity".

There are different branches of mathematics that use the term differently, there are different number systems with different definitions of infinity, there are different philosophical views in the philosophy of mathematics held by mathematicians in regard to whether any mathematical object can or cannot ever be infinite and in regard to what infinity means. The term infinity is variously used by mathematicians to refer to finite, only potentially infinite objects, and other times to actual infinite objects.

There are mathematicians who accept only potential infinity which is not even infinite, there are those who accept actual infinity, and there are finitists and ultrafinitists and others with other views on the matter.


You are viewing this all according to your own philosophical position without taking into consideration what the other positions and other mathematical definitions are.

For example, you assert that there is no upper limit to the successor function. There are mathematicians who would agree with you and there are many mathematicians who would disagree with you, and there are mathematical definitions that you would not be in accordance with by making this assertion. Some mathematicians hold the position that there is a finite upper limit. Some hold the position that if you cannot calculate a number, it is meaningless. Or that, if a number is larger than the number of atoms in the Universe, it no longer has meaning. Many mathematicians see the first infinite number as being the limit of the successor function for the finite numbers.

Your idea of "reachable" number is based on the philosophical viewpoint you currently hold. You don't seem to realize that what you think of as "reachable" does not mean "finite" in most contexts, it only means "finite" in the limited context of this aspect of the subject in regard to your philosophical view on the subject. Graham's number is not universally regarded as reachable by mathematicians. Many mathematicians who accept actual infinity do hold the view that the successor function will eventually reach actual infinity. In the number lines based on different number systems I presented a few posts back, the idea that the finite does not lead to infinite is not tenable. That is a lot of mathematical number systems, with mathematical definitions, from mathematicians, to disagree with if you are insisting that your view on this is the only possible mathematical interpretation of the topic.

How, in any of those number systems, in those diagrams I posted, could you suggest that the finite does not lead to the infinite, or that the successor function would not eventually get to those infinite numbers? How can you support this claim?

Practically speaking, if G represents Graham's number, a distance of G planck lengths away is unreachable.

A distance of infinity planck lengths away, where infinity is actual infinity, the infinity of the apeirogon, is reachable.

Any number X for which a regular polygon with X vertices has a 180°angle between adjacent edges must have at least one infinitely-long "gap" that cannot be bridged by the successor function.

This is your claim, but it has not been substantiated and you haven't given any kind of intuitive explanation of how this could be possible, any coherant definition of what this even means, why it should be true, or how it would play out if it was true.

If an apeirogon has edge #1, #2, #3, #4, and so on, with edge #∞ to the left of edge #1, and edge #∞-1 to the left of edge #∞, where is this supposed discontinuity? How will moving to the right, starting with 1 then 2 then 3, without limit, not get to edge ∞ and back to edge 1? I am having a very hard time seeing how you could possibly think this could ever be true in any way, let alone why you would take it as some kind of self evident, universally accepted axiom that must be true no matter what. Can you give an explanation of this, or is this just an assumption that you hold regardless of evidence against it?

And if you really believe this, it seems to suggest that you don't believe apeirogons, any apeirotopes, any tilings or honeycombs or any infinite dimensional objects are genuine geometric objects. How can there be a valid geometric object that supposedly has some infinite gap in it, which is still treated as if it is connected?

Do you think of a square tiling as having infinite gaps in it? How is that even meaningful in terms of how these shapes are defined? They are defined as not having gaps. What do you even mean by this?


How do you not see that as a major problem with your position on this?

[adam ∞]

Or think of it this way:

If you accept the premise that there is some first infinite number,

how could adding 1 an unlimited amount of times not eventually reach this first infinite number if by definition it is an unlimited amount of times?

If it is unlimited, how can you claim it is limited in its extent to reach the first infinite number?

Are you suggesting that you think of "infinity" as being in some sense higher than any unlimited amount? If so, do you not see how this is a contradictory definition?

[steelpillow]

Just some thoughts. I haven't taken in all the preceding discussion.

Infinities are notoriously difficult to deal with, especially when you discover an infinity of infinities and try to organise them by, say, developing an algebra of infinities. I recall somebody trying around 1970-80-ish. He eventually found that his algebra was already a well-known one, just he was declaring that he was applying it to infinities. Cantor's ideas are about the best we have - as far as they go.

Some infinities vary in their characteristics depending on where you encounter them.
For example in the Euclidean plane a line is an unending thing, with infinity an ideal that is forever outside the geometry. The line thus has no ends.
But in the projective plane every line crosses infinity; it has no ends because it is a closed loop. There is a line at infinity, sometimes called the Absolute line. Every other line crosses it just once, and simply comes back into the finite plane from the other side. To cope with this analytically, we add an extra coordinate to the usual x,y ones, say w. Infinite distances are represented by ratios such as x/w, where w = 0. This system is known as homogeneous coordinates.
The hyperbolic plane is bounded by infinity. But a transfinite geometry also exists outside the plane.

An apeirogon can be constructed on any line in these geometries, or indeed any curve (as long as it is not a finite discrete geometry!). There are several ways to do this. One is endless subdivision; on a circle, the circle itself is the limiting shape. Another is endless extension along an infinite line or curve. Projective measures are examples of apeirogons which may appear like this last, but typically converge at one or both ends to a single point. The defining characteristic of such as sequence is that cross-ratios of the gaps between four consecutive members are always preserved. These gaps may be segments between points on a line or curve, or angles between lines in a point.

The number line extends "to infinity", which is paradoxical, but for a moment let us go the other way, to infinitesimals. Some infinite series converge on a finite number. Between any two numbers in any system (other than staying with integers) we may insert an infinity of more finely-grained numbers. And between any two of those, we can do the same. Infinitely many times. If we can create a system for discovering the next number, we call it a countable infinity. If we cannot, we call it un-countable. It is generally held that un-countables are larger than countables, but I am not sure if that can be proved without assuming something like it in the first place, rendering the proof circular.

A number very much larger than any you are currently considering is generally referred to as indefinitely large. Some people are happy to describe them as infinite, others not. Ultimately, "infinity" means what you want it to mean. There are many kinds, each with their own philosophical and mathematical baggage. Getting them muddled up is all too common.

One thing scientists love to say is that there are no infinities in nature. Where they appear in the maths, they are boundary conditions where the maths breaks down and no longer describes nature. Cosmologists point to the proposed singularity at the heart of a black hole as an example of such a breakdown. However it is quite remarkable how many of them will then turn round and vehemently declare that the Universe is infinite - and they have the maths to prove it!

[PatrickPowers]

It is generally held that un-countables are larger than countables, but I am not sure if that can be proved without assuming something like it in the first place, rendering the proof circular.

Cantor's proof is both convincing and very simple.

I used to think there were no infinities in nature until it became popular to suppose our Universe is infinite. Maybe it is, maybe it isn't, but it doesn't make much practical difference.

[steelpillow]

Cantor's proof is both convincing and very simple.

It does not convince me. Cantor assumes that you can reach the end of the infinite series and declare something is missing. But you can never reach the end of an infinite series, so you can never make such a declaration. This kind of fallacy, that by considering finite steps you can prove something about infinity, also trips up the Leibniz/Newton "proofs" of the mechanics of infinitesimals (the differential and integral calculi): just because a sequence of finite approximations appears to converge on a particular result does not prove that, at the limit where the difference between terms reaches zero, the apparent result is valid. I recall someone claiming ca.1970 that they had fixed this flaw, only to have that claim rebutted shortly afterwards. There has since been another fix offered, which is generally accepted. Frankly, it has never made any better sense to me than the last fix. But what do I know.
Two more accepted examples of such "fallacies to infinity" include:
1) The idea that infinity is a thing in Euclidean geometry. For example in the plane, infinity is not a part of the Euclidean plane. It does not extend "to infinity", it extends "indefinitely" and never reached infinity. There is no infinity within the geometry itself. You might think that is just sophistry, but in projective geometry the use of homogeneous coordinates allows the geometry to continue to, across and beyond infinity. Indeed, the Euclidean plane has been described as the projective plane with the line at infinity ripped out.
2) JW Dunne's idea that there are infinite series of time dimensions and consciousness levels, at the end of which God resides. It was pointed out that he proposed that his series must be infinite because they never ended; he could not really make any claim about what might lie at the non-existent end. He came to accept this argument and rewrote his seminal work, An Experiment with Time. with all references to "infinity" removed.

I used to think there were no infinities in nature until it became popular to suppose our Universe is infinite. Maybe it is, maybe it isn't, but it doesn't make much practical difference.

When it became popular to suppose our Universe is infinite, I reacted by losing my faith in those speculative physicists. People who claim that the Universe is infinite are always the ones into wacky speculations like wormholes, doppelgangers, Boltzmann brains, eternal inflation and other games played with finite numbers in an infinite playpen. It's wall-to-wall metaphysics, no better than medieval theologians debating the infinite aspects of God, or which Archangel stands on the right hand of the Saviour. There are enough wonders in reality, and there is enough joy in fantasy, without having to pretend one's fantasies are actually real.